Transformational theory
Encyclopedia
Transformational theory is a branch of music theory
developed by David Lewin
in the 1980s, and formally introduced in his most influential work, Generalized Musical Intervals and Transformations (1987). The theory, which models musical transformations
as elements of a mathematical group
, can be used to analyze both tonal
and atonal music.
The goal of transformational theory is to change the focus from musical objects—such as the "C major chord
" or "G major chord" -- to relations between objects. Thus, instead of saying that a C major chord is followed by G major, a transformational theorist might say that the first chord has been "transformed" into the second by the "Dominant
operation." (Symbolically, one might write "Dominant(C major) = G major.") While traditional musical set theory focuses on the makeup of musical objects, transformational theory focuses on the intervals
or types of musical motion that can occur. According to Lewin's description of this change in emphasis, "[The transformational] attitude does not ask for some observed measure of extension between reified 'points'; rather it asks: 'If I am at s and wish to get to t, what characteristic gesture should I perform in order to arrive there?'" (GMIT, p. 159)
Lewin points out that this requirement significantly constrains the spaces and transformations that can be considered. For example, if the space S is the space of diatonic triads (represented by the Roman numerals I, ii, iii, IV, V, vi, and vii°), the "Dominant transformation" must be defined so as to apply to each of these triads. This means, for example, that some diatonic triad must be selected to be the "dominant" of the diminished triad on vii. In ordinary musical discourse, however, the "dominant" relationship is typically held to obtain only between the I and V chords. (Certainly, there is no diatonic triad that is ordinarily considered to be the dominant of the diminished triad.) In other words, "dominant," as used informally, is not a function applying to all chords, but rather a particular relationship that holds between two of them.
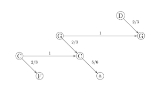
There are, however, any number of situations in which "transformations" can indeed be extended to an entire space. Here, transformational theory provides a degree of abstraction that is potentially a significant music-theoretical asset. One transformational network can describe the relationships among musical events in more than one musical excerpt, thus offering an elegant way of relating them. For example, figure 7.9 in Lewin's GMIT—shown in the illustration on this page—can describe the first phrases of both the first and third movements of Beethoven's Symphony No. 1 in C Major, Op. 21
. In this case, the transformation graph's objects are the same in both excerpts from the Beethoven Symphony, but this graph could apply to many more musical examples when the object labels are removed. Further, such a transformational network that gives only the intervals between pitch classes in an excerpt may also describe the differences in the relative durations of another excerpt in a piece, thus succinctly relating two different domains of music analysis. Lewin's observation that only the transformations and not the objects upon which they act are necessary to specify a transformational network is the main benefit of transformational analysis over traditional object-oriented analysis.
However, several theorists have pointed out that ordinary musical discourse often includes more information than functions. For example, a single pair of pitch classes (such as C and E) can stand in multiple relationships: E is both a major third above C and a minor sixth below it. (This is analogous to the fact that, on an ordinary clockface, the number 4 is both four steps clockwise from 12 and 8 steps counterclockwise of it.) For this reason, theorists such as Dmitri Tymoczko
have proposed replacing Lewinnian "pitch class intervals" with "paths in pitch class space." More generally, this suggests that there are situations where it might not be useful to model musical motion ("transformations" in the intuitive sense) using functions ("transformations" in the strict sense of Lewinnian theory).
Another issue concerns the role of "distance" in transformational theory. In the opening pages of GMIT, Lewin suggests that a subspecies of "transformations" (namely, musical intervals) can be used to model "directed measurements, distances, or motions ". However, the mathematical formalism he uses—in which "transformations" are modeled by group elements—does not obviously represent distances, since group elements are not typically considered to have size. (Groups are typically individuated only up to isomorphism, and isomorphism will not necessarily preserve the "sizes" that are assigned to group elements.) Theorists such as Ed Gollin, Dmitri Tymoczko, and Rachel Hall, have all written about this subject, with Gollin attempting to incorporate "distances" into a broadly Lewinnian framework.
Tymoczko's "Generalizing Musical Intervals" contains one of the few extended critiques of transformational theory, arguing (1) that intervals are sometimes "local" objects which, like vectors, cannot be transported around a musical space; (2) that musical spaces often have boundaries, or multiple paths between the same points, both prohibited by Lewin's formalism; and (3) that transformational theory implicitly relies on notions of distance extraneous to the formalism as such.
's three contextual inversion operations on triads (parallel
, relative
, and Leittonwechsel) as formal transformations, the branch of transformation theory called Neo-Riemannian theory
was popularized by Brian Hyer (1995), Michael Kevin Mooney (1996), Richard Cohn
(1997), and an entire issue of the Journal of Music Theory
(42/2, 1998). Transformation theory has received further treatment by Fred Lerdahl
(2001), Julian Hook (2002), David Kopp (2002), and many others.
The status of transformational theory is currently a topic of debate in music-theoretical circles. Some authors, such as Ed Gollin, Dmitri Tymoczko
and Julian Hook, have argued that Lewin's transformational formalism is too restrictive, and have called for extending the system in various ways. Others, such as Richard Cohn
and Steven Rings, while acknowledging the validity of some of these criticisms, continue to use broadly Lewinnian techniques.
Music theory
Music theory is the study of how music works. It examines the language and notation of music. It seeks to identify patterns and structures in composers' techniques across or within genres, styles, or historical periods...
developed by David Lewin
David Lewin
David Lewin was an American music theorist, music critic and composer. Called "the most original and far-ranging theorist of his generation" , he did his most influential theoretical work on the development of transformational theory, which involves the application of mathematical group theory to...
in the 1980s, and formally introduced in his most influential work, Generalized Musical Intervals and Transformations (1987). The theory, which models musical transformations
Transformation (music)
In music, a transformation consists of any operation or process that may apply to a musical variable in composition, performance, or analysis. Transformations include multiplication, rotation, permutation In music, a transformation consists of any operation or process that may apply to a musical...
as elements of a mathematical group
Group theory
In mathematics and abstract algebra, group theory studies the algebraic structures known as groups.The concept of a group is central to abstract algebra: other well-known algebraic structures, such as rings, fields, and vector spaces can all be seen as groups endowed with additional operations and...
, can be used to analyze both tonal
Tonality
Tonality is a system of music in which specific hierarchical pitch relationships are based on a key "center", or tonic. The term tonalité originated with Alexandre-Étienne Choron and was borrowed by François-Joseph Fétis in 1840...
and atonal music.
The goal of transformational theory is to change the focus from musical objects—such as the "C major chord
Major chord
In music theory, a major chord is a chord having a root, a major third, and a perfect fifth. When a chord has these three notes alone, it is called a major triad...
" or "G major chord" -- to relations between objects. Thus, instead of saying that a C major chord is followed by G major, a transformational theorist might say that the first chord has been "transformed" into the second by the "Dominant
Dominant (music)
In music, the dominant is the fifth scale degree of the diatonic scale, called "dominant" because it is next in importance to the tonic,and a dominant chord is any chord built upon that pitch, using the notes of the same diatonic scale...
operation." (Symbolically, one might write "Dominant(C major) = G major.") While traditional musical set theory focuses on the makeup of musical objects, transformational theory focuses on the intervals
Interval (music)
In music theory, an interval is a combination of two notes, or the ratio between their frequencies. Two-note combinations are also called dyads...
or types of musical motion that can occur. According to Lewin's description of this change in emphasis, "[The transformational] attitude does not ask for some observed measure of extension between reified 'points'; rather it asks: 'If I am at s and wish to get to t, what characteristic gesture should I perform in order to arrive there?'" (GMIT, p. 159)
Formalism
The formal setting for Lewin's theory is a set S (or "space") of musical objects, and a set T of transformations on that space. Transformations are modeled as functions acting on the entire space, meaning that every transformation must be applicable to every object.Lewin points out that this requirement significantly constrains the spaces and transformations that can be considered. For example, if the space S is the space of diatonic triads (represented by the Roman numerals I, ii, iii, IV, V, vi, and vii°), the "Dominant transformation" must be defined so as to apply to each of these triads. This means, for example, that some diatonic triad must be selected to be the "dominant" of the diminished triad on vii. In ordinary musical discourse, however, the "dominant" relationship is typically held to obtain only between the I and V chords. (Certainly, there is no diatonic triad that is ordinarily considered to be the dominant of the diminished triad.) In other words, "dominant," as used informally, is not a function applying to all chords, but rather a particular relationship that holds between two of them.
There are, however, any number of situations in which "transformations" can indeed be extended to an entire space. Here, transformational theory provides a degree of abstraction that is potentially a significant music-theoretical asset. One transformational network can describe the relationships among musical events in more than one musical excerpt, thus offering an elegant way of relating them. For example, figure 7.9 in Lewin's GMIT—shown in the illustration on this page—can describe the first phrases of both the first and third movements of Beethoven's Symphony No. 1 in C Major, Op. 21
Symphony No. 1 (Beethoven)
Ludwig van Beethoven's Symphony No. 1 in C major, Op. 21, was dedicated to Baron Gottfried van Swieten, an early patron of the composer. The piece was published in 1801 by Hoffmeister & Kühnel of Leipzig...
. In this case, the transformation graph's objects are the same in both excerpts from the Beethoven Symphony, but this graph could apply to many more musical examples when the object labels are removed. Further, such a transformational network that gives only the intervals between pitch classes in an excerpt may also describe the differences in the relative durations of another excerpt in a piece, thus succinctly relating two different domains of music analysis. Lewin's observation that only the transformations and not the objects upon which they act are necessary to specify a transformational network is the main benefit of transformational analysis over traditional object-oriented analysis.
Transformations as Functions
The "transformations" of transformational theory are typically modeled as functions that act over some musical space S, meaning that they are entirely defined by their inputs and outputs: for instance, the "ascending major third" might be modeled as a function which takes a particular pitch class as input and outputs the pitch class a major third above it.However, several theorists have pointed out that ordinary musical discourse often includes more information than functions. For example, a single pair of pitch classes (such as C and E) can stand in multiple relationships: E is both a major third above C and a minor sixth below it. (This is analogous to the fact that, on an ordinary clockface, the number 4 is both four steps clockwise from 12 and 8 steps counterclockwise of it.) For this reason, theorists such as Dmitri Tymoczko
Dmitri Tymoczko
Dmitri Tymoczko is a composer and music theorist. His music, which draws on rock, jazz, and romanticism, has been performed by ensembles such as the Ansermet Quartet, the Brentano Quartet, Janus, Newspeak, the San Francisco Contemporary Players, the Pacifica Quartet, and Ursula Opens...
have proposed replacing Lewinnian "pitch class intervals" with "paths in pitch class space." More generally, this suggests that there are situations where it might not be useful to model musical motion ("transformations" in the intuitive sense) using functions ("transformations" in the strict sense of Lewinnian theory).
Another issue concerns the role of "distance" in transformational theory. In the opening pages of GMIT, Lewin suggests that a subspecies of "transformations" (namely, musical intervals) can be used to model "directed measurements, distances, or motions ". However, the mathematical formalism he uses—in which "transformations" are modeled by group elements—does not obviously represent distances, since group elements are not typically considered to have size. (Groups are typically individuated only up to isomorphism, and isomorphism will not necessarily preserve the "sizes" that are assigned to group elements.) Theorists such as Ed Gollin, Dmitri Tymoczko, and Rachel Hall, have all written about this subject, with Gollin attempting to incorporate "distances" into a broadly Lewinnian framework.
Tymoczko's "Generalizing Musical Intervals" contains one of the few extended critiques of transformational theory, arguing (1) that intervals are sometimes "local" objects which, like vectors, cannot be transported around a musical space; (2) that musical spaces often have boundaries, or multiple paths between the same points, both prohibited by Lewin's formalism; and (3) that transformational theory implicitly relies on notions of distance extraneous to the formalism as such.
Reception
Although transformation theory is more than twenty years old, it did not become a widespread theoretical or analytical pursuit until the late 1990s. Following Lewin's revival (in GMIT) of Hugo RiemannHugo Riemann
Karl Wilhelm Julius Hugo Riemann was a German music theorist.-Biography:Riemann was born at Grossmehlra, Schwarzburg-Sondershausen. He was educated in theory by Frankenberger, studied the piano with Barthel and Ratzenberger, studied law, and finally philosophy and history at Berlin and Tübingen...
's three contextual inversion operations on triads (parallel
Parallel key
In music, parallel keys are the major and minor scales that have the same tonic. A major and minor scale sharing the same tonic are said to be in a parallel relationship...
, relative
Relative key
In music, relative keys are the major and minor scales that have the same key signatures. A major and minor scale sharing the same key signature are said to be in a relative relationship...
, and Leittonwechsel) as formal transformations, the branch of transformation theory called Neo-Riemannian theory
Neo-Riemannian theory
Neo-Riemannian theory refers to a loose collection of ideas present in the writings of music theorists such as David Lewin, Brian Hyer, Richard Cohn, and Henry Klumpenhouwer...
was popularized by Brian Hyer (1995), Michael Kevin Mooney (1996), Richard Cohn
Richard Cohn
Richard Cohn is a music theorist and Battell Professor of Music Theory at Yale. Early in his career, he specialized in the music of Béla Bartók, but more recently has written about Neo-Riemannian theory as well as metric dissonance.-External links:*...
(1997), and an entire issue of the Journal of Music Theory
JMT
JMT may refer to:* JMT Records, a record label that specialized in contemporary jazz* Jedi Mind Tricks, a hip hop group with two members from Philadelphia, Pennsylvania and one from Camden, New Jersey...
(42/2, 1998). Transformation theory has received further treatment by Fred Lerdahl
Fred Lerdahl
Alfred Whitford Lerdahl is the Fritz Reiner Professor of Musical Composition at Columbia University, and a composer and music theorist best known for his work on pitch space and cognitive constraints on compositional systems or "musical grammar[s]." He has written many orchestral and chamber...
(2001), Julian Hook (2002), David Kopp (2002), and many others.
The status of transformational theory is currently a topic of debate in music-theoretical circles. Some authors, such as Ed Gollin, Dmitri Tymoczko
Dmitri Tymoczko
Dmitri Tymoczko is a composer and music theorist. His music, which draws on rock, jazz, and romanticism, has been performed by ensembles such as the Ansermet Quartet, the Brentano Quartet, Janus, Newspeak, the San Francisco Contemporary Players, the Pacifica Quartet, and Ursula Opens...
and Julian Hook, have argued that Lewin's transformational formalism is too restrictive, and have called for extending the system in various ways. Others, such as Richard Cohn
Richard Cohn
Richard Cohn is a music theorist and Battell Professor of Music Theory at Yale. Early in his career, he specialized in the music of Béla Bartók, but more recently has written about Neo-Riemannian theory as well as metric dissonance.-External links:*...
and Steven Rings, while acknowledging the validity of some of these criticisms, continue to use broadly Lewinnian techniques.
Further reading
- Lewin, David. Generalized Musical Intervals and Transformations (Yale University Press: New Haven, CT, 1987)
- Lewin, David. "Transformational Techniques in Atonal and Other Music Theories", Perspectives of New Music, xxi (1982–3), 312–71
- Lewin, David. Musical Form and Transformation: Four Analytic Essays (Yale University Press: New Haven, CT, 1993)
- Tymoczko, Dmitri, "Generalizing Musical Intervals," Journal of Music Theory 53/2 (2009): 227–254.
- Lerdahl, Fred. Tonal Pitch Space (Oxford University Press: New York, 2001)
- Hook, Julian. "Uniform Triadic Transformations" (Ph.D. dissertation, Indiana University, 2002)
- Kopp, David. Chromatic Transformations in Nineteenth-century Music (Cambridge University Press, 2002)
- Hyer, Brian. "Reimag(in)ing Riemann", Journal of Music Theory, 39/1 (1995), 101–138
- Mooney, Michael Kevin. "The `Table of Relations' and Music Psychology in Hugo Riemann's Chromatic Theory" (Ph.D. dissertation, Columbia University, 1996)
- Cohn, Richard. "Neo-Riemannian Operations, Parsimonious Trichords, and their Tonnetz Representations", Journal of Music Theory, 41/1 (1997), 1–66
External links
- This Week's Finds in Mathematical Physics (Week 234) by John Baez

