Abel's inequality
Encyclopedia
In mathematics
, Abel's inequality, named after Niels Henrik Abel
, supplies a simple bound on the absolute value of the inner product of two vectors in an important special case.
Let {fn} be a sequence of real number
s such that fn ≥ fn+1 > 0 for n = 1, 2, …, and let {an} be a sequence of real or complex number
s. Then
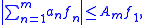
where
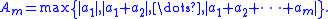
The inequality also holds for infinite series, in the limit as
, if 
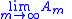exists.
Mathematics
Mathematics is the study of quantity, space, structure, and change. Mathematicians seek out patterns and formulate new conjectures. Mathematicians resolve the truth or falsity of conjectures by mathematical proofs, which are arguments sufficient to convince other mathematicians of their validity...
, Abel's inequality, named after Niels Henrik Abel
Niels Henrik Abel
Niels Henrik Abel was a Norwegian mathematician who proved the impossibility of solving the quintic equation in radicals.-Early life:...
, supplies a simple bound on the absolute value of the inner product of two vectors in an important special case.
Let {fn} be a sequence of real number
Real number
In mathematics, a real number is a value that represents a quantity along a continuum, such as -5 , 4/3 , 8.6 , √2 and π...
s such that fn ≥ fn+1 > 0 for n = 1, 2, …, and let {an} be a sequence of real or complex number
Complex number
A complex number is a number consisting of a real part and an imaginary part. Complex numbers extend the idea of the one-dimensional number line to the two-dimensional complex plane by using the number line for the real part and adding a vertical axis to plot the imaginary part...
s. Then
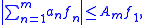
where
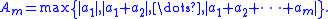
The inequality also holds for infinite series, in the limit as
, if 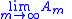exists.