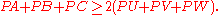Barrow's inequality
Encyclopedia
In geometry
, Barrow's inequality states the following: Let P be a point inside the triangle
ABC; U, V, and W be the points where the angle bisectors of BPC, CPA, and APB intersect the sides BC, CA, AB, respectively. Then
Barrow's inequality strengthens the Erdős–Mordell inequality
, which has a similar form with PU, PV, and PW replaced by the three distances of P from the triangle's sides. It is named after David Francis Barrow.
Geometry
Geometry arose as the field of knowledge dealing with spatial relationships. Geometry was one of the two fields of pre-modern mathematics, the other being the study of numbers ....
, Barrow's inequality states the following: Let P be a point inside the triangle
Triangle
A triangle is one of the basic shapes of geometry: a polygon with three corners or vertices and three sides or edges which are line segments. A triangle with vertices A, B, and C is denoted ....
ABC; U, V, and W be the points where the angle bisectors of BPC, CPA, and APB intersect the sides BC, CA, AB, respectively. Then
Barrow's inequality strengthens the Erdős–Mordell inequality
Erdos–Mordell inequality
In Euclidean geometry, the Erdős–Mordell inequality states that for any triangle ABC and point O inside ABC, the sum of the distances from O to the sides is less than or equal to half of the sum of the distances from O to the vertices. It is named after Paul Erdős and Louis Mordell. posed the...
, which has a similar form with PU, PV, and PW replaced by the three distances of P from the triangle's sides. It is named after David Francis Barrow.