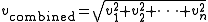Bonnet's theorem
Encyclopedia
In classical mechanics
, Bonnet's theorem states that if n different force
fields each produce the same geometric orbit (say, an ellipse of given dimensions) albeit with different speed
s v1, v2,...,vn at a given point P, then the same orbit will be followed if the speed at point P equals
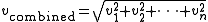
This theorem was first derived by Adrien-Marie Legendre
in 1817, but it is named after Pierre Ossian Bonnet
.
is determined only by the centripetal force
s at each point of the orbit, which are the forces acting perpendicular to the orbit. By contrast, forces along the orbit change only the speed, but not the direction, of the velocity
.
Let the instantaneous radius of curvature at a point P on the orbit be denoted as R. For the kth force field that produces that orbit, the force normal to the orbit Fk must provide the centripetal force

Adding all these forces together yields the equation
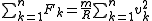
Hence, the combined force-field produces the same orbit if the speed at a point P is set equal to
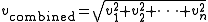
Classical mechanics
In physics, classical mechanics is one of the two major sub-fields of mechanics, which is concerned with the set of physical laws describing the motion of bodies under the action of a system of forces...
, Bonnet's theorem states that if n different force
Force
In physics, a force is any influence that causes an object to undergo a change in speed, a change in direction, or a change in shape. In other words, a force is that which can cause an object with mass to change its velocity , i.e., to accelerate, or which can cause a flexible object to deform...
fields each produce the same geometric orbit (say, an ellipse of given dimensions) albeit with different speed
Speed
In kinematics, the speed of an object is the magnitude of its velocity ; it is thus a scalar quantity. The average speed of an object in an interval of time is the distance traveled by the object divided by the duration of the interval; the instantaneous speed is the limit of the average speed as...
s v1, v2,...,vn at a given point P, then the same orbit will be followed if the speed at point P equals
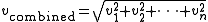
This theorem was first derived by Adrien-Marie Legendre
Adrien-Marie Legendre
Adrien-Marie Legendre was a French mathematician.The Moon crater Legendre is named after him.- Life :...
in 1817, but it is named after Pierre Ossian Bonnet
Pierre Ossian Bonnet
Pierre Ossian Bonnet was a French mathematician. He made some important contributions to the differential geometry of surfaces, including the Gauss-Bonnet theorem.-Early years:...
.
Derivation
The shape of an orbitOrbit
In physics, an orbit is the gravitationally curved path of an object around a point in space, for example the orbit of a planet around the center of a star system, such as the Solar System...
is determined only by the centripetal force
Centripetal force
Centripetal force is a force that makes a body follow a curved path: it is always directed orthogonal to the velocity of the body, toward the instantaneous center of curvature of the path. The mathematical description was derived in 1659 by Dutch physicist Christiaan Huygens...
s at each point of the orbit, which are the forces acting perpendicular to the orbit. By contrast, forces along the orbit change only the speed, but not the direction, of the velocity
Velocity
In physics, velocity is speed in a given direction. Speed describes only how fast an object is moving, whereas velocity gives both the speed and direction of the object's motion. To have a constant velocity, an object must have a constant speed and motion in a constant direction. Constant ...
.
Let the instantaneous radius of curvature at a point P on the orbit be denoted as R. For the kth force field that produces that orbit, the force normal to the orbit Fk must provide the centripetal force
Centripetal force
Centripetal force is a force that makes a body follow a curved path: it is always directed orthogonal to the velocity of the body, toward the instantaneous center of curvature of the path. The mathematical description was derived in 1659 by Dutch physicist Christiaan Huygens...

Adding all these forces together yields the equation
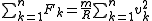
Hence, the combined force-field produces the same orbit if the speed at a point P is set equal to