
Kolmogorov's zero-one law
Encyclopedia
In probability theory
, Kolmogorov's zero-one law, named in honor of Andrey Nikolaevich Kolmogorov, specifies that a certain type of event
, called a tail event, will either almost surely
happen or almost surely not happen; that is, the probability
of such an event occurring is zero or one.
Tail events are defined in terms of infinite sequence
s of random variable
s. Suppose
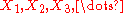
is an infinite sequence of independent
random variables (not necessarily identically distributed). Then, a tail event is an event whose occurrence or failure is determined by the values of these random variables but which is probabilistically independent
of each finite subset of these random variables. For example, the event that the series
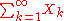
converges, is a tail event. The event that the sum to which it converges is more than 1 is not a tail event, since, for example, it is not independent of the value of X1. In an infinite sequence of coin-tosses, a sequence of 100 consecutive heads occurring infinitely many times is a tail event.
In many situations, it can be easy to apply Kolmogorov's zero-one law to show that some event has probability 0 or 1, but surprisingly hard to determine which of these two extreme values is the correct one.
and let Fn be a sequence of mutually independent σ-algebras contained in F. Let
be the smallest σ-algebra containing Fn, Fn+1, …. Then Kolmogorov's zero-one law asserts that for any event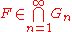
one has either P(F) = 0 or 1.
The statement of the law in terms of random variables is obtained from the latter by taking each Fn to be the σ-algebra generated by the random variable Xn. A tail event is then by definition an event which is measurable with respect to the σ-algebra generated by all Xn, but which is independent of any finite number of Xn. That is, a tail event is precisely an element of the intersection .
.
Probability theory
Probability theory is the branch of mathematics concerned with analysis of random phenomena. The central objects of probability theory are random variables, stochastic processes, and events: mathematical abstractions of non-deterministic events or measured quantities that may either be single...
, Kolmogorov's zero-one law, named in honor of Andrey Nikolaevich Kolmogorov, specifies that a certain type of event
Event (probability theory)
In probability theory, an event is a set of outcomes to which a probability is assigned. Typically, when the sample space is finite, any subset of the sample space is an event...
, called a tail event, will either almost surely
Almost surely
In probability theory, one says that an event happens almost surely if it happens with probability one. The concept is analogous to the concept of "almost everywhere" in measure theory...
happen or almost surely not happen; that is, the probability
Probability
Probability is ordinarily used to describe an attitude of mind towards some proposition of whose truth we arenot certain. The proposition of interest is usually of the form "Will a specific event occur?" The attitude of mind is of the form "How certain are we that the event will occur?" The...
of such an event occurring is zero or one.
Tail events are defined in terms of infinite sequence
Sequence
In mathematics, a sequence is an ordered list of objects . Like a set, it contains members , and the number of terms is called the length of the sequence. Unlike a set, order matters, and exactly the same elements can appear multiple times at different positions in the sequence...
s of random variable
Random variable
In probability and statistics, a random variable or stochastic variable is, roughly speaking, a variable whose value results from a measurement on some type of random process. Formally, it is a function from a probability space, typically to the real numbers, which is measurable functionmeasurable...
s. Suppose
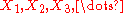
is an infinite sequence of independent
Statistical independence
In probability theory, to say that two events are independent intuitively means that the occurrence of one event makes it neither more nor less probable that the other occurs...
random variables (not necessarily identically distributed). Then, a tail event is an event whose occurrence or failure is determined by the values of these random variables but which is probabilistically independent
Statistical independence
In probability theory, to say that two events are independent intuitively means that the occurrence of one event makes it neither more nor less probable that the other occurs...
of each finite subset of these random variables. For example, the event that the series
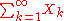
converges, is a tail event. The event that the sum to which it converges is more than 1 is not a tail event, since, for example, it is not independent of the value of X1. In an infinite sequence of coin-tosses, a sequence of 100 consecutive heads occurring infinitely many times is a tail event.
In many situations, it can be easy to apply Kolmogorov's zero-one law to show that some event has probability 0 or 1, but surprisingly hard to determine which of these two extreme values is the correct one.
Formulation
A more general statement of Kolmogorov's zero-one law holds for sequences of independent σ-algebras. Let (Ω,F,P) be a probability spaceProbability space
In probability theory, a probability space or a probability triple is a mathematical construct that models a real-world process consisting of states that occur randomly. A probability space is constructed with a specific kind of situation or experiment in mind...
and let Fn be a sequence of mutually independent σ-algebras contained in F. Let

be the smallest σ-algebra containing Fn, Fn+1, …. Then Kolmogorov's zero-one law asserts that for any event
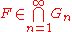
one has either P(F) = 0 or 1.
The statement of the law in terms of random variables is obtained from the latter by taking each Fn to be the σ-algebra generated by the random variable Xn. A tail event is then by definition an event which is measurable with respect to the σ-algebra generated by all Xn, but which is independent of any finite number of Xn. That is, a tail event is precisely an element of the intersection
 .
.External links
- The Legacy of Andrei Nikolaevich Kolmogorov Curriculum Vitae and Biography. Kolmogorov School. Ph.D. students and descendants of A.N. Kolmogorov. A.N. Kolmogorov works, books, papers, articles. Photographs and Portraits of A.N. Kolmogorov.

