Mason's Invariant
Encyclopedia
In electronics
, Mason's invariant, named after Samuel Jefferson Mason
, is a measure of the quality of transistor
s.
"When trying to solve a seemingly difficult problem, Sam said to concentrate on the easier ones first; the rest, including the hardest ones, will follow," recalled Andrew Viterbi
, co-founder and former vice-president of Qualcomm
. He had been a thesis advisee under Samuel Mason at MIT, and this was one lesson he especially remembered from his professor. A few years earlier, Mason had heeded his own advice when he defined a unilateral power gain for a linear two-port device, or U. After concentrating on easier problems with power gain in feedback amplifiers, a figure of merit
for all three-terminal devices followed that is still used today as Mason's Invariant.
. They were beginning to be used for RF
applications, and they were limited to VHF frequencies and below. Mason wanted to find a figure of merit to compare transistors, and this led him to discover that the unilateral power gain
of a linear two-port device was an invariant figure of merit.
In his paper Power Gain
in Feedback Amplifiers published in 1953, Mason stated in his introduction,
Then, according to Madhu Gupta in Power Gain in Feedback Amplifiers, a Classic Revisited, Mason defined the problem as "being the search for device properties that are invariant with respect to transformations as represented by an embedding network" that satisfy the four constraints listed below.
He next showed that all transformations that satisfy the above constraints can be accomplished with just three simple transformations performed sequentially. Similarly, this is the same as representing an embedding network by a set of three embedding networks nested within one another. The three mathematical expressions can be seen below.
1. Reactance padding:
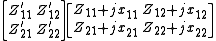
2. Real Transformations:
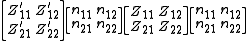
3. Inversion:
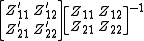
Mason then considered which quantities remained invariant under each of these three transformations. His conclusions, listed respectively to the transformations above, are shown below. Each transformation left the values below unchanged.
1. Reactance padding:
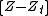
and
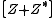
2. Real transformations:
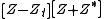
and
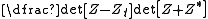
3. Inversion:
The magnitudes of the two determinants and the sign of the denominator in the above fraction remain unchanged in the inversion transformation. Consequently, the quantity invariant under all three conditions is:
Electronics
Electronics is the branch of science, engineering and technology that deals with electrical circuits involving active electrical components such as vacuum tubes, transistors, diodes and integrated circuits, and associated passive interconnection technologies...
, Mason's invariant, named after Samuel Jefferson Mason
Samuel Jefferson Mason
Samuel Jefferson Mason was an American electronics engineer. Mason's invariant and Mason's rule are named after him.He was born in New York City, but he grew up in a small town in New Jersey. It was so small, in fact, that it only had a population of 26. He received a B.S...
, is a measure of the quality of transistor
Transistor
A transistor is a semiconductor device used to amplify and switch electronic signals and power. It is composed of a semiconductor material with at least three terminals for connection to an external circuit. A voltage or current applied to one pair of the transistor's terminals changes the current...
s.
"When trying to solve a seemingly difficult problem, Sam said to concentrate on the easier ones first; the rest, including the hardest ones, will follow," recalled Andrew Viterbi
Andrew Viterbi
Andrew James Viterbi, Ph.D. is an Italian-American electrical engineer and businessman who co-founded Qualcomm Inc....
, co-founder and former vice-president of Qualcomm
Qualcomm
Qualcomm is an American global telecommunication corporation that designs, manufactures and markets digital wireless telecommunications products and services based on its code division multiple access technology and other technologies. Headquartered in San Diego, CA, USA...
. He had been a thesis advisee under Samuel Mason at MIT, and this was one lesson he especially remembered from his professor. A few years earlier, Mason had heeded his own advice when he defined a unilateral power gain for a linear two-port device, or U. After concentrating on easier problems with power gain in feedback amplifiers, a figure of merit
Figure of merit
A figure of merit is a quantity used to characterize the performance of a device, system or method, relative to its alternatives. In engineering, figures of merit are often defined for particular materials or devices in order to determine their relative utility for an application...
for all three-terminal devices followed that is still used today as Mason's Invariant.
Origin
In 1953, transistors were only five years old, and they were the only successful, three-terminal active devicePassivity (engineering)
Passivity is a property of engineering systems, used in a variety of engineering disciplines, but most commonly found in analog electronics and control systems...
. They were beginning to be used for RF
Radio frequency
Radio frequency is a rate of oscillation in the range of about 3 kHz to 300 GHz, which corresponds to the frequency of radio waves, and the alternating currents which carry radio signals...
applications, and they were limited to VHF frequencies and below. Mason wanted to find a figure of merit to compare transistors, and this led him to discover that the unilateral power gain
Gain
In electronics, gain is a measure of the ability of a circuit to increase the power or amplitude of a signal from the input to the output. It is usually defined as the mean ratio of the signal output of a system to the signal input of the same system. It may also be defined on a logarithmic scale,...
of a linear two-port device was an invariant figure of merit.
In his paper Power Gain
Gain
In electronics, gain is a measure of the ability of a circuit to increase the power or amplitude of a signal from the input to the output. It is usually defined as the mean ratio of the signal output of a system to the signal input of the same system. It may also be defined on a logarithmic scale,...
in Feedback Amplifiers published in 1953, Mason stated in his introduction,
He wanted to find a metric to characterize and measure the quality of transistors since up until then, no such measure existed. Little did Mason know, however, that he would discover an equation that is still used more than 50 years later and does much more than measure the quality of a transistor.
"A vacuum tubeVacuum tubeIn electronics, a vacuum tube, electron tube , or thermionic valve , reduced to simply "tube" or "valve" in everyday parlance, is a device that relies on the flow of electric current through a vacuum...
, very often represented as a simple transconductanceTransconductanceTransconductance, also known as mutual conductance, is a property of certain electronic components. Conductance is the reciprocal of resistance; transconductance, meanwhile, is the ratio of the current change at the output port to the voltage change at the input port. It is written as gm...
driving a passive impedanceElectrical impedanceElectrical impedance, or simply impedance, is the measure of the opposition that an electrical circuit presents to the passage of a current when a voltage is applied. In quantitative terms, it is the complex ratio of the voltage to the current in an alternating current circuit...
, may lead to relatively simple amplifierAmplifierGenerally, an amplifier or simply amp, is a device for increasing the power of a signal.In popular use, the term usually describes an electronic amplifier, in which the input "signal" is usually a voltage or a current. In audio applications, amplifiers drive the loudspeakers used in PA systems to...
designs in which the input impedance (and hence the power gainGainIn electronics, gain is a measure of the ability of a circuit to increase the power or amplitude of a signal from the input to the output. It is usually defined as the mean ratio of the signal output of a system to the signal input of the same system. It may also be defined on a logarithmic scale,...
) is effectively infinite, the voltageVoltageVoltage, otherwise known as electrical potential difference or electric tension is the difference in electric potential between two points — or the difference in electric potential energy per unit charge between two points...
gainGainIn electronics, gain is a measure of the ability of a circuit to increase the power or amplitude of a signal from the input to the output. It is usually defined as the mean ratio of the signal output of a system to the signal input of the same system. It may also be defined on a logarithmic scale,...
is the quantity of interest, and the input circuit is isolated from the load. The transistorTransistorA transistor is a semiconductor device used to amplify and switch electronic signals and power. It is composed of a semiconductor material with at least three terminals for connection to an external circuit. A voltage or current applied to one pair of the transistor's terminals changes the current...
, however, usually cannot be characterized so easily."
Derivation of U
Mason first defined the device being studied with the three constraints listed below.- The device has only two ports (at which power can be transferred between it and outside devices).
- The device is linear (in its relationships of currents and voltages at the two ports).
- The device is used in a specified manner (connected as an amplifier between a linear one-port source and a linear one-port load).
Then, according to Madhu Gupta in Power Gain in Feedback Amplifiers, a Classic Revisited, Mason defined the problem as "being the search for device properties that are invariant with respect to transformations as represented by an embedding network" that satisfy the four constraints listed below.
- The embedding network is a four-port.
- The embedding network is linear.
- The embedding network is lossless.
- The embedding network is reciprocal.
He next showed that all transformations that satisfy the above constraints can be accomplished with just three simple transformations performed sequentially. Similarly, this is the same as representing an embedding network by a set of three embedding networks nested within one another. The three mathematical expressions can be seen below.
1. Reactance padding:
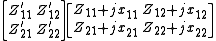
2. Real Transformations:
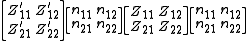
3. Inversion:
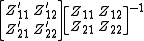
Mason then considered which quantities remained invariant under each of these three transformations. His conclusions, listed respectively to the transformations above, are shown below. Each transformation left the values below unchanged.
1. Reactance padding:
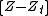
and
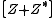
2. Real transformations:
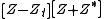
and
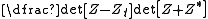
3. Inversion:
The magnitudes of the two determinants and the sign of the denominator in the above fraction remain unchanged in the inversion transformation. Consequently, the quantity invariant under all three conditions is:
-
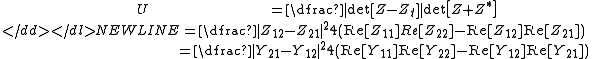
Importance
Mason's Invariant, or U, is the only device characteristic that is invariant under lossless, reciprocal embeddings. In other words, U can be used as a figure of merit to compare any three-terminal, active device. For example, a factory producing BJTs can calculate U of the transistors it is producing and compare their quality to the other BJTs on the market. Furthermore, U can be used as an indicator of activity. If U is greater than one, the two-port device is active; otherwise, that device is passive. This is especially useful in the microwaveMicrowaveMicrowaves, a subset of radio waves, have wavelengths ranging from as long as one meter to as short as one millimeter, or equivalently, with frequencies between 300 MHz and 300 GHz. This broad definition includes both UHF and EHF , and various sources use different boundaries...
engineering community. Though originally published in a circuit theory journal, Mason's paper becomes especially relevant to microwaveMicrowaveMicrowaves, a subset of radio waves, have wavelengths ranging from as long as one meter to as short as one millimeter, or equivalently, with frequencies between 300 MHz and 300 GHz. This broad definition includes both UHF and EHF , and various sources use different boundaries...
engineers since U is usually slightly greater to or equal to one in the microwaveMicrowaveMicrowaves, a subset of radio waves, have wavelengths ranging from as long as one meter to as short as one millimeter, or equivalently, with frequencies between 300 MHz and 300 GHz. This broad definition includes both UHF and EHF , and various sources use different boundaries...
frequency range. When U is smaller than or considerably larger than one, it becomes relatively useless.
While Mason's Invariant can be used as a figure of merit across all operating frequencies, its value at ƒmax is especially useful. Fmax is the maximum oscillationOscillationOscillation is the repetitive variation, typically in time, of some measure about a central value or between two or more different states. Familiar examples include a swinging pendulum and AC power. The term vibration is sometimes used more narrowly to mean a mechanical oscillation but sometimes...
frequency of a device, and it is discovered when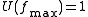
. This frequency is also the frequency at which the maximum stable gainGainIn electronics, gain is a measure of the ability of a circuit to increase the power or amplitude of a signal from the input to the output. It is usually defined as the mean ratio of the signal output of a system to the signal input of the same system. It may also be defined on a logarithmic scale,...
Gms and the maximum available gainGainIn electronics, gain is a measure of the ability of a circuit to increase the power or amplitude of a signal from the input to the output. It is usually defined as the mean ratio of the signal output of a system to the signal input of the same system. It may also be defined on a logarithmic scale,...
Gma of the device become one. Consequently, ƒmax is a characteristic of the device, and it has the significance that it is the maximum frequency of oscillationOscillationOscillation is the repetitive variation, typically in time, of some measure about a central value or between two or more different states. Familiar examples include a swinging pendulum and AC power. The term vibration is sometimes used more narrowly to mean a mechanical oscillation but sometimes...
in a circuit where only one active device is present, the device is embedded in a passive network, and only single sinusoidal signals are of interest.
Conclusion
In his revisit of Mason's paper, Gupta states, "Perhaps the most convincing evidence of the utility of the concept of a unilateral power gainGainIn electronics, gain is a measure of the ability of a circuit to increase the power or amplitude of a signal from the input to the output. It is usually defined as the mean ratio of the signal output of a system to the signal input of the same system. It may also be defined on a logarithmic scale,...
as a device figure of merit is the fact that for the last three decades, practically every new, active, two-port device developed for high frequency use has been carefully scrutinized for the achievable value of U..." This assumption is appropriate because "Umax" or "maximum unilateral gainGainIn electronics, gain is a measure of the ability of a circuit to increase the power or amplitude of a signal from the input to the output. It is usually defined as the mean ratio of the signal output of a system to the signal input of the same system. It may also be defined on a logarithmic scale,...
" is still listed on transistorTransistorA transistor is a semiconductor device used to amplify and switch electronic signals and power. It is composed of a semiconductor material with at least three terminals for connection to an external circuit. A voltage or current applied to one pair of the transistor's terminals changes the current...
specification sheets, and Mason's Invariant is still taught in some undergraduate electrical engineering curricula—ones that Mason, no doubt, had a hand in innovating. Though now it has been over five decades, Mason's finding of an invariant device characteristic still plays a significant role in transistorTransistorA transistor is a semiconductor device used to amplify and switch electronic signals and power. It is composed of a semiconductor material with at least three terminals for connection to an external circuit. A voltage or current applied to one pair of the transistor's terminals changes the current...
design.