
Tijdeman's theorem
Encyclopedia
In number theory, Tijdeman's theorem states that there are at most a finite number of consecutive powers. Stated another way, the set of solutions in integers x, y, n, m of the exponential diophantine equation
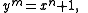
for exponents n and m greater than one, is finite.
The theorem was proven by Dutch number theorist Robert Tijdeman
in 1976, and provided a strong impetus towards the eventual proof of Catalan's conjecture by Preda Mihăilescu
. Mihăilescu's theorem
states that there is only one member to the set of consecutive power pairs, namely 9=8+1.
That the powers are consecutive is essential to Tijdeman's proof; if we replace a difference of one by any other difference k and ask for the number of solutions
of
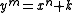
with n and m greater than one we have an unsolved problem. It is conjectured that this set also will be finite; its finiteness would follow, for instance, from the abc conjecture
.
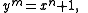
for exponents n and m greater than one, is finite.
The theorem was proven by Dutch number theorist Robert Tijdeman
Robert Tijdeman
Robert Tijdeman is a Dutch mathematician. Specializing in number theory, he is best known for his Tijdeman's theorem. He is a professor of mathematics at the Leiden University since 1975, and was chairman of the department of mathematics and computer science at Leiden from 1991 to 1993...
in 1976, and provided a strong impetus towards the eventual proof of Catalan's conjecture by Preda Mihăilescu
Preda Mihailescu
Preda V. Mihăilescu is a Romanian mathematician, best known for his proof of Catalan's conjecture.Born in Bucharest, he is the brother of Vintilă Mihăilescu. After leaving Romania in 1973, he settled in Switzerland. He studied mathematics and computer science in Zürich, receiving his Ph.D. from...
. Mihăilescu's theorem
Mihailescu's theorem
Catalan's conjecture is a theorem in number theory that was conjectured by the mathematician Eugène Charles Catalan in 1844 and proven in 2002 by Preda Mihăilescu....
states that there is only one member to the set of consecutive power pairs, namely 9=8+1.
That the powers are consecutive is essential to Tijdeman's proof; if we replace a difference of one by any other difference k and ask for the number of solutions
of
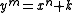
with n and m greater than one we have an unsolved problem. It is conjectured that this set also will be finite; its finiteness would follow, for instance, from the abc conjecture
Abc conjecture
The abc conjecture is a conjecture in number theory, first proposed by Joseph Oesterlé and David Masser in 1985. The conjecture is stated in terms of three positive integers, a, b and c , which have no common factor and satisfy a + b = c...
.

