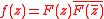Ahiezer's theorem
Encyclopedia
In the mathematical
field of complex analysis
, Akhiezer's theorem is a result about entire function
s proved by Naum Akhiezer.
of exponential type , with for real . Then the following are equivalent:
Mathematics
Mathematics is the study of quantity, space, structure, and change. Mathematicians seek out patterns and formulate new conjectures. Mathematicians resolve the truth or falsity of conjectures by mathematical proofs, which are arguments sufficient to convince other mathematicians of their validity...
field of complex analysis
Complex analysis
Complex analysis, traditionally known as the theory of functions of a complex variable, is the branch of mathematical analysis that investigates functions of complex numbers. It is useful in many branches of mathematics, including number theory and applied mathematics; as well as in physics,...
, Akhiezer's theorem is a result about entire function
Entire function
In complex analysis, an entire function, also called an integral function, is a complex-valued function that is holomorphic over the whole complex plane...
s proved by Naum Akhiezer.
Statement
Let be an entire functionEntire function
In complex analysis, an entire function, also called an integral function, is a complex-valued function that is holomorphic over the whole complex plane...
of exponential type , with for real . Then the following are equivalent:
- There exists an entire functionEntire functionIn complex analysis, an entire function, also called an integral function, is a complex-valued function that is holomorphic over the whole complex plane...
, of exponential type , having all its zeros in the (closed) upper half plane, such that
- One has:
-

where are the zeros of .