Cahn–Hilliard equation
Encyclopedia
The Cahn–Hilliard equation (after John W. Cahn
and John E. Hilliard) is an equation
of mathematical physics
which describes the process of phase
separation, by which the two components of a binary fluid spontaneously separate and form domains pure in each component. If
is the concentration of the fluid, with 
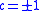indicating domains, then the equation is written as
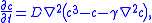
where
is a diffusion
coefficient with units of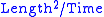
and 
gives the length of the transition regions between the domains. Here 
is the partial time derivative and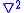 
is the Laplacian in 
dimensions. Additionally, the quantity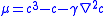 
is identified as a chemical potential.
al. Specifically, if we identify
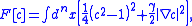
as a free energy functional, then
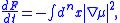
so that the free energy decays to zero. This also indicates segregation into domains is the asymptotic outcome of the evolution of this equation.
In real experiments, the segregation of an initially mixed binary fluid into domains is observed. The segregation is characterized by the following facts.
The Cahn–Hilliard equations finds applications in diverse fields: in interfacial fluid flow, polymer science and in industrial applications. The solution of the Cahn-Hilliard equation for a binary mixture demonstrated to coincide well with the solution of a Stefan problem
and the model of Thomas and Windle. Of interest to researchers at present is the coupling of the phase separation of the Cahn–Hilliard equation to the Navier–Stokes equations of fluid flow.
John W. Cahn
John Werner Cahn is an American scientist and winner of the 1998 National Medal of Science. He was a professor in the department of Materials Science at MIT from 1964-1978. Since 1977, he has held a position at the National Institute of Standards and Technology . Dr...
and John E. Hilliard) is an equation
Equation
An equation is a mathematical statement that asserts the equality of two expressions. In modern notation, this is written by placing the expressions on either side of an equals sign , for examplex + 3 = 5\,asserts that x+3 is equal to 5...
of mathematical physics
Mathematical physics
Mathematical physics refers to development of mathematical methods for application to problems in physics. The Journal of Mathematical Physics defines this area as: "the application of mathematics to problems in physics and the development of mathematical methods suitable for such applications and...
which describes the process of phase
Phase (matter)
In the physical sciences, a phase is a region of space , throughout which all physical properties of a material are essentially uniform. Examples of physical properties include density, index of refraction, and chemical composition...
separation, by which the two components of a binary fluid spontaneously separate and form domains pure in each component. If
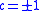is the concentration of the fluid, with indicating domains, then the equation is written as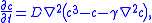where
is a diffusionDiffusion
Molecular diffusion, often called simply diffusion, is the thermal motion of all particles at temperatures above absolute zero. The rate of this movement is a function of temperature, viscosity of the fluid and the size of the particles...
coefficient with units of
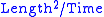and gives the length of the transition regions between the domains. Here is the partial time derivative and 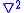is the Laplacian in dimensions. Additionally, the quantity 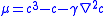is identified as a chemical potential.Features and applications
Of interest to mathematicians is the existence of a unique solution to the Cahn–Hilliard equation, given smooth initial data. The proof relies essentially on the existence of a Lyapunov functionLyapunov function
In the theory of ordinary differential equations , Lyapunov functions are scalar functions that may be used to prove the stability of an equilibrium of an ODE. Named after the Russian mathematician Aleksandr Mikhailovich Lyapunov, Lyapunov functions are important to stability theory and control...
al. Specifically, if we identify
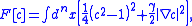
as a free energy functional, then
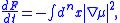
so that the free energy decays to zero. This also indicates segregation into domains is the asymptotic outcome of the evolution of this equation.
In real experiments, the segregation of an initially mixed binary fluid into domains is observed. The segregation is characterized by the following facts.
- There is a transition layer between the segregated domains, with a profile given by the function
and hence a typical width 
because this function is an equilibrium solution of the Cahn–Hilliard equation.
- Of interest also is the fact that the segregated domains grow in time as a power law. That is, if
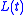is a typical domain size, then 
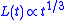. This is the Lifshitz–Slyozov law, and has been proved rigorously for the Cahn–Hilliard equation and observed in numerical simulations and real experiments on binary fluids.
- The Cahn–Hilliard equation has the form of a conservation law,
with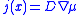 
. Thus the phase separation process conserves the total concentration 
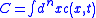, so that 
.
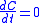- When one phase is significantly more abundant, the Cahn–Hilliard equation can show the phenomena known as Ostwald ripeningOstwald ripeningright|thumb|300px|Basic schematic of the Ostwald ripening process Ostwald ripening is an observed phenomenon in solid solutions or liquid sols which describes the change of an inhomogeneous structure over time...
, where the minority phase forms spherical droplets, and the smaller droplets are absorbed through diffusion into the larger ones.
The Cahn–Hilliard equations finds applications in diverse fields: in interfacial fluid flow, polymer science and in industrial applications. The solution of the Cahn-Hilliard equation for a binary mixture demonstrated to coincide well with the solution of a Stefan problem
Stefan problem
In mathematics and its applications, particularly to phase transitions in matter, a Stefan problem is a particular kind of boundary value problem for a partial differential equation , adapted to the case in which a phase boundary can move with time...
and the model of Thomas and Windle. Of interest to researchers at present is the coupling of the phase separation of the Cahn–Hilliard equation to the Navier–Stokes equations of fluid flow.