Cylindrical multipole moments
Encyclopedia
Cylindrical multipole moments are the coefficients in a series expansion
of a potential
that varies logarithmically with the distance to a source, i.e., as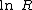 . Such potentials arise in the electric potential
. Such potentials arise in the electric potential
of long line charges, and the analogous sources for the magnetic potential
and gravitational potential.
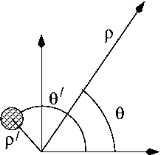
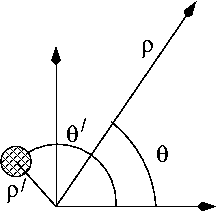
For clarity, we illustrate the expansion for a single line charge, then generalize to an arbitrary distribution of line charges. Through this article, the primed coordinates such
as refer to the position of the line charge(s), whereas the unprimed coordinates such as
refer to the position of the line charge(s), whereas the unprimed coordinates such as  refer to the point at which the potential is being observed. We use cylindrical coordinates throughout, e.g., an arbitrary vector
refer to the point at which the potential is being observed. We use cylindrical coordinates throughout, e.g., an arbitrary vector  has coordinates
has coordinates 
where is the radius from the
is the radius from the  axis,
axis, 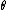 is the azimuthal angle and
is the azimuthal angle and  is the normal Cartesian coordinate. By assumption, the line charges are infinitely long and aligned with the
is the normal Cartesian coordinate. By assumption, the line charges are infinitely long and aligned with the  axis.
axis.
 The electric potential
The electric potential
of a line charge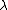 located at
located at  is given by
is given by
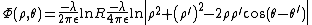
where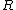 is the shortest distance between the line charge and the observation point.
is the shortest distance between the line charge and the observation point.
By symmetry, the electric potential of an infinite linecharge has no -dependence. The line charge
-dependence. The line charge  is the charge per unit length in the
is the charge per unit length in the
 -direction, and has units of (charge/length). If the radius
-direction, and has units of (charge/length). If the radius 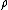 of the observation point is greater than the radius
of the observation point is greater than the radius 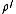 of the line charge, we may factor out
of the line charge, we may factor out 

and expand the logarithm
s in powers of
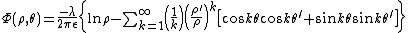
which may be written as
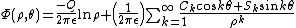
where the multipole moments are defined as
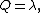

and
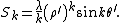
Conversely, if the radius of the observation point is less than the radius
of the observation point is less than the radius 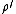 of the line charge, we may factor out
of the line charge, we may factor out  and expand the logarithms in powers of
and expand the logarithms in powers of 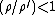
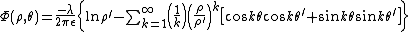
which may be written as

where the interior multipole moments are defined as
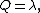
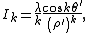
and
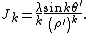
 is straightforward. The functional form is the same
is straightforward. The functional form is the same
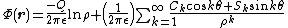
and the moments can be written
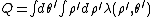

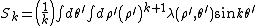
Note that the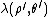 represents the line charge per unit area in the
represents the line charge per unit area in the  plane.
plane.
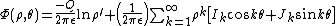
where the moments are defined

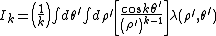

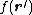 be the second charge density, and define
be the second charge density, and define 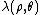 as its integral over z
as its integral over z

The electrostatic energy is given by the integral of the charge multiplied by the potential due to the cylindrical multipoles

If the cylindrical multipoles are exterior, this equation becomes
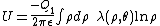

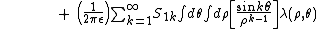
where ,
,  and
and  are the cylindrical multipole moments of charge distribution 1. This energy formula can be reduced to a remarkably simple form
are the cylindrical multipole moments of charge distribution 1. This energy formula can be reduced to a remarkably simple form
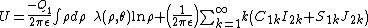
where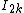 and
and  are the interior cylindrical multipoles of the second charge density.
are the interior cylindrical multipoles of the second charge density.
The analogous formula holds if charge density 1 is composed of interior cylindrical multipoles
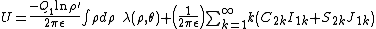
where and
and 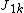 are the interior cylindrical multipole moments of charge distribution 1, and
are the interior cylindrical multipole moments of charge distribution 1, and 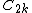 and
and  are the
are the
exterior cylindrical multipoles of the second charge density.
As an example, these formulae could be used to determine the interaction energy of a small protein
in the electrostatic field of a double-stranded DNA
molecule; the latter is relatively straight and bears a constant linear charge density due to the phosphate
groups of its backbone.
Series expansion
In mathematics, a series expansion is a method for calculating a function that cannot be expressed by just elementary operators . The resulting so-called series often can be limited to a finite number of terms, thus yielding an approximation of the function...
of a potential
Potential
*In linguistics, the potential mood*The mathematical study of potentials is known as potential theory; it is the study of harmonic functions on manifolds...
that varies logarithmically with the distance to a source, i.e., as
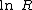 . Such potentials arise in the electric potential
. Such potentials arise in the electric potentialElectric potential
In classical electromagnetism, the electric potential at a point within a defined space is equal to the electric potential energy at that location divided by the charge there...
of long line charges, and the analogous sources for the magnetic potential
Magnetic potential
The term magnetic potential can be used for either of two quantities in classical electromagnetism: the magnetic vector potential, A, and the magnetic scalar potential, ψ...
and gravitational potential.
For clarity, we illustrate the expansion for a single line charge, then generalize to an arbitrary distribution of line charges. Through this article, the primed coordinates such
as
 refer to the position of the line charge(s), whereas the unprimed coordinates such as
refer to the position of the line charge(s), whereas the unprimed coordinates such as 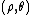 refer to the point at which the potential is being observed. We use cylindrical coordinates throughout, e.g., an arbitrary vector
refer to the point at which the potential is being observed. We use cylindrical coordinates throughout, e.g., an arbitrary vector  has coordinates
has coordinates 
where
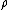 is the radius from the
is the radius from the  axis,
axis, 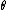 is the azimuthal angle and
is the azimuthal angle and  is the normal Cartesian coordinate. By assumption, the line charges are infinitely long and aligned with the
is the normal Cartesian coordinate. By assumption, the line charges are infinitely long and aligned with the  axis.
axis.Cylindrical multipole moments of a line charge

Electric potential
In classical electromagnetism, the electric potential at a point within a defined space is equal to the electric potential energy at that location divided by the charge there...
of a line charge
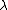 located at
located at  is given by
is given by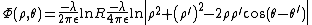
where
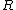 is the shortest distance between the line charge and the observation point.
is the shortest distance between the line charge and the observation point.By symmetry, the electric potential of an infinite linecharge has no
 -dependence. The line charge
-dependence. The line charge  is the charge per unit length in the
is the charge per unit length in the -direction, and has units of (charge/length). If the radius
-direction, and has units of (charge/length). If the radius 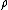 of the observation point is greater than the radius
of the observation point is greater than the radius 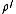 of the line charge, we may factor out
of the line charge, we may factor out 

and expand the logarithm
Logarithm
The logarithm of a number is the exponent by which another fixed value, the base, has to be raised to produce that number. For example, the logarithm of 1000 to base 10 is 3, because 1000 is 10 to the power 3: More generally, if x = by, then y is the logarithm of x to base b, and is written...
s in powers of

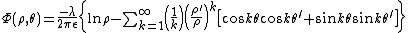
which may be written as
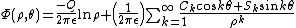
where the multipole moments are defined as
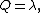

and
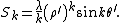
Conversely, if the radius
 of the observation point is less than the radius
of the observation point is less than the radius 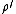 of the line charge, we may factor out
of the line charge, we may factor out  and expand the logarithms in powers of
and expand the logarithms in powers of 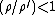
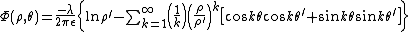
which may be written as

where the interior multipole moments are defined as
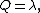
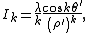
and
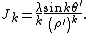
General cylindrical multipole moments
The generalization to an arbitrary distribution of line charges is straightforward. The functional form is the same
is straightforward. The functional form is the same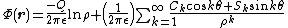
and the moments can be written
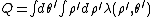

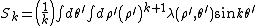
Note that the
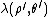 represents the line charge per unit area in the
represents the line charge per unit area in the  plane.
plane.Interior cylindrical multipole moments
Similarly, the interior cylindrical multipole expansion has the functional form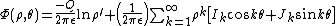
where the moments are defined

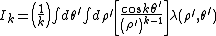

Interaction energies of cylindrical multipoles
A simple formula for the interaction energy of cylindrical multipoles (charge density 1) with a second charge density can be derived. Let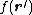 be the second charge density, and define
be the second charge density, and define 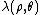 as its integral over z
as its integral over z
The electrostatic energy is given by the integral of the charge multiplied by the potential due to the cylindrical multipoles

If the cylindrical multipoles are exterior, this equation becomes
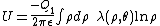

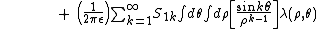
where
 ,
,  and
and  are the cylindrical multipole moments of charge distribution 1. This energy formula can be reduced to a remarkably simple form
are the cylindrical multipole moments of charge distribution 1. This energy formula can be reduced to a remarkably simple form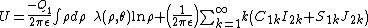
where
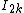 and
and  are the interior cylindrical multipoles of the second charge density.
are the interior cylindrical multipoles of the second charge density.The analogous formula holds if charge density 1 is composed of interior cylindrical multipoles
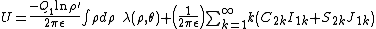
where
 and
and 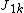 are the interior cylindrical multipole moments of charge distribution 1, and
are the interior cylindrical multipole moments of charge distribution 1, and 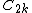 and
and  are the
are theexterior cylindrical multipoles of the second charge density.
As an example, these formulae could be used to determine the interaction energy of a small protein
Protein
Proteins are biochemical compounds consisting of one or more polypeptides typically folded into a globular or fibrous form, facilitating a biological function. A polypeptide is a single linear polymer chain of amino acids bonded together by peptide bonds between the carboxyl and amino groups of...
in the electrostatic field of a double-stranded DNA
DNA
Deoxyribonucleic acid is a nucleic acid that contains the genetic instructions used in the development and functioning of all known living organisms . The DNA segments that carry this genetic information are called genes, but other DNA sequences have structural purposes, or are involved in...
molecule; the latter is relatively straight and bears a constant linear charge density due to the phosphate
Phosphate
A phosphate, an inorganic chemical, is a salt of phosphoric acid. In organic chemistry, a phosphate, or organophosphate, is an ester of phosphoric acid. Organic phosphates are important in biochemistry and biogeochemistry or ecology. Inorganic phosphates are mined to obtain phosphorus for use in...
groups of its backbone.
See also
- Potential theoryPotential theoryIn mathematics and mathematical physics, potential theory may be defined as the study of harmonic functions.- Definition and comments :The term "potential theory" was coined in 19th-century physics, when it was realized that the fundamental forces of nature could be modeled using potentials which...
- Multipole momentsMultipole momentsIn mathematics, especially as applied to physics, multipole moments are the coefficients of a series expansion of a potential due to continuous or discrete sources . A multipole moment usually involves powers of the distance to the origin, as well as some angular dependence...
- Multipole expansionMultipole expansionA multipole expansion is a mathematical series representing a function that depends on angles — usually the two angles on a sphere. These series are useful because they can often be truncated, meaning that only the first few terms need to be retained for a good approximation to the original...
- Axial multipole momentsAxial multipole momentsAxial multipole moments are a series expansionof the electric potential of acharge distribution localized close tothe origin along oneCartesian axis,denoted here as the z-axis...
- Spherical multipole momentsSpherical multipole momentsSpherical multipole moments are the coefficients in a series expansionof a potential that varies inversely with the distance R to a source, i.e., as 1/R...

