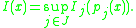Dawson-Gärtner theorem
Encyclopedia
In mathematics
, the Dawson–Gärtner theorem is a result in large deviations theory
. Heuristically speaking, the Dawson–Gärtner theorem allows one to transport a large deviation principle on a “smaller” topological space
to a “larger” one.
with maps pij : Yj → Yi. Let X be the projective limit (also known as the inverse limit) of the system (Yj, pij)i,j∈J, i.e.
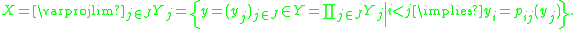
Let (με)ε>0 be a family of probability measure
s on X. Assume that, for each j ∈ J, the push-forward measures (pj∗με)ε>0 on Yj satisfy the large deviation principle with good rate function Ij : Yj → R ∪ {+∞}. Then the family (με)ε>0 satisfies the large deviation principle on X with good rate function I : X → R ∪ {+∞} given by
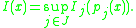
Mathematics
Mathematics is the study of quantity, space, structure, and change. Mathematicians seek out patterns and formulate new conjectures. Mathematicians resolve the truth or falsity of conjectures by mathematical proofs, which are arguments sufficient to convince other mathematicians of their validity...
, the Dawson–Gärtner theorem is a result in large deviations theory
Large deviations theory
In probability theory, the theory of large deviations concerns the asymptotic behaviour of remote tails of sequences of probability distributions. Some basic ideas of the theory can be tracked back to Laplace and Cramér, although a clear unified formal definition was introduced in 1966 by Varadhan...
. Heuristically speaking, the Dawson–Gärtner theorem allows one to transport a large deviation principle on a “smaller” topological space
Topological space
Topological spaces are mathematical structures that allow the formal definition of concepts such as convergence, connectedness, and continuity. They appear in virtually every branch of modern mathematics and are a central unifying notion...
to a “larger” one.
Statement of the theorem
Let (Yj)j∈J be a projective system of Hausdorff topological spacesHausdorff space
In topology and related branches of mathematics, a Hausdorff space, separated space or T2 space is a topological space in which distinct points have disjoint neighbourhoods. Of the many separation axioms that can be imposed on a topological space, the "Hausdorff condition" is the most frequently...
with maps pij : Yj → Yi. Let X be the projective limit (also known as the inverse limit) of the system (Yj, pij)i,j∈J, i.e.
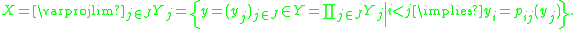
Let (με)ε>0 be a family of probability measure
Probability measure
In mathematics, a probability measure is a real-valued function defined on a set of events in a probability space that satisfies measure properties such as countable additivity...
s on X. Assume that, for each j ∈ J, the push-forward measures (pj∗με)ε>0 on Yj satisfy the large deviation principle with good rate function Ij : Yj → R ∪ {+∞}. Then the family (με)ε>0 satisfies the large deviation principle on X with good rate function I : X → R ∪ {+∞} given by