Dirichlet principle
Encyclopedia
In mathematics
, Dirichlet's principle in potential theory
states that, if the function u(x) is the solution to Poisson's equation
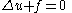
on a domain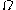
of 
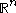with boundary condition
then u can be obtained as the minimizer of the Dirichlet's energy
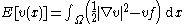
amongst all twice differentiable functions
such that 
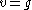on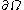 
(provided that there exists at least one function making the Dirichlet's integral finite). This concept is named after the German mathematician Lejeune Dirichlet.
Since the Dirichlet's integral is bounded from below, the existence of an infimum
is guaranteed. That this infimum is attained was taken for granted by Riemann (who coined the term Dirichlet's principle) and others until Weierstraß gave an example of a functional that does not attain its minimum. Hilbert
later justified Riemann's use of Dirichlet's principle.
Mathematics
Mathematics is the study of quantity, space, structure, and change. Mathematicians seek out patterns and formulate new conjectures. Mathematicians resolve the truth or falsity of conjectures by mathematical proofs, which are arguments sufficient to convince other mathematicians of their validity...
, Dirichlet's principle in potential theory
Potential theory
In mathematics and mathematical physics, potential theory may be defined as the study of harmonic functions.- Definition and comments :The term "potential theory" was coined in 19th-century physics, when it was realized that the fundamental forces of nature could be modeled using potentials which...
states that, if the function u(x) is the solution to Poisson's equation
Poisson's equation
In mathematics, Poisson's equation is a partial differential equation of elliptic type with broad utility in electrostatics, mechanical engineering and theoretical physics...
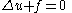
on a domain
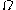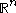of with boundary conditionthen u can be obtained as the minimizer of the Dirichlet's energy
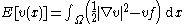
amongst all twice differentiable functions
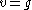such that on 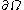(provided that there exists at least one function making the Dirichlet's integral finite). This concept is named after the German mathematician Lejeune Dirichlet.Since the Dirichlet's integral is bounded from below, the existence of an infimum
Infimum
In mathematics, the infimum of a subset S of some partially ordered set T is the greatest element of T that is less than or equal to all elements of S. Consequently the term greatest lower bound is also commonly used...
is guaranteed. That this infimum is attained was taken for granted by Riemann (who coined the term Dirichlet's principle) and others until Weierstraß gave an example of a functional that does not attain its minimum. Hilbert
David Hilbert
David Hilbert was a German mathematician. He is recognized as one of the most influential and universal mathematicians of the 19th and early 20th centuries. Hilbert discovered and developed a broad range of fundamental ideas in many areas, including invariant theory and the axiomatization of...
later justified Riemann's use of Dirichlet's principle.
See also
- Plateau's problemPlateau's problemIn mathematics, Plateau's problem is to show the existence of a minimal surface with a given boundary, a problem raised by Joseph-Louis Lagrange in 1760. However, it is named after Joseph Plateau who was interested in soap films. The problem is considered part of the calculus of variations...
- Green's first identity