In number theory

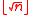Number theory

Number theory is a branch of pure mathematics devoted primarily to the study of the integers. Number theorists study prime numbers as well...

, a Durfee square is an attribute of an integer partition

Partition (number theory)

In number theory and combinatorics, a partition of a positive integer n, also called an integer partition, is a way of writing n as a sum of positive integers. Two sums that differ only in the order of their summands are considered to be the same partition; if order matters then the sum becomes a...

. A partition of n has a Durfee square of side s if s is the largest number such that the partition contains at least s parts with values ≥ s. An equivalent, but more visual, definition is that the Durfee square is the largest square that is contained within a partition's Ferrers diagram.

For example, the partition 4 + 3 + 3 + 2 + 1 + 1:


has a Durfee square of side 3 (in red) because it contains 3 parts that are ≥ 3, but does not contain 4 parts that are ≥ 4.

History

Durfee squares are named after William Durfee, a pupil of English mathematician James Joseph Sylvester

James Joseph Sylvester

James Joseph Sylvester was an English mathematician. He made fundamental contributions to matrix theory, invariant theory, number theory, partition theory and combinatorics...

. In a letter to Arthur Cayley

Arthur Cayley

Arthur Cayley F.R.S. was a British mathematician. He helped found the modern British school of pure mathematics....

in 1883, Sylvester wrote:

Properties

It is clear from the visual definition that the Durfee square of a partition and its conjugate partition have the same size. The partitions of an integer n contain Durfee squares with sides up to and including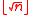 .