
Erdos–Turan conjecture
Encyclopedia
Erdős' conjecture on arithmetic progressions, often incorrectly referred to as the Erdős–Turán conjecture
(see also Erdős–Turán conjecture on additive bases
), is a conjecture
in additive combinatorics due to Paul Erdős
. It states that if the sum of the reciprocals of the members of a set A of positive integers diverges, then A contains arbitrarily long arithmetic progression
s.
Formally, if
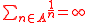
(i.e. A is a large set) then A contains arithmetic progressions of any given length.
If true, the theorem would generalize Szemerédi's theorem
.
Erdős offered a prize of US$3000 for a proof of this conjecture at the time. The problem is currently worth US$5000.
The Green–Tao theorem on arithmetic progressions in the primes
is a special case of this conjecture.
Erdos–Turan conjecture
Erdős' conjecture on arithmetic progressions, often incorrectly referred to as the Erdős–Turán conjecture , is a conjecture in additive combinatorics due to Paul Erdős...
(see also Erdős–Turán conjecture on additive bases
Erdős–Turán conjecture on additive bases
The Erdős–Turán conjecture is an old unsolved problem in additive number theory posed by Paul Erdős and Pál Turán in 1941.-History:...
), is a conjecture
Conjecture
A conjecture is a proposition that is unproven but is thought to be true and has not been disproven. Karl Popper pioneered the use of the term "conjecture" in scientific philosophy. Conjecture is contrasted by hypothesis , which is a testable statement based on accepted grounds...
in additive combinatorics due to Paul Erdős
Paul Erdos
Paul Erdős was a Hungarian mathematician. Erdős published more papers than any other mathematician in history, working with hundreds of collaborators. He worked on problems in combinatorics, graph theory, number theory, classical analysis, approximation theory, set theory, and probability theory...
. It states that if the sum of the reciprocals of the members of a set A of positive integers diverges, then A contains arbitrarily long arithmetic progression
Arithmetic progression
In mathematics, an arithmetic progression or arithmetic sequence is a sequence of numbers such that the difference between the consecutive terms is constant...
s.
Formally, if
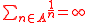
(i.e. A is a large set) then A contains arithmetic progressions of any given length.
If true, the theorem would generalize Szemerédi's theorem
Szemerédi's theorem
In number theory, Szemerédi's theorem is a result that was formerly the Erdős–Turán conjecture...
.
Erdős offered a prize of US$3000 for a proof of this conjecture at the time. The problem is currently worth US$5000.
The Green–Tao theorem on arithmetic progressions in the primes
Prime number
A prime number is a natural number greater than 1 that has no positive divisors other than 1 and itself. A natural number greater than 1 that is not a prime number is called a composite number. For example 5 is prime, as only 1 and 5 divide it, whereas 6 is composite, since it has the divisors 2...
is a special case of this conjecture.

