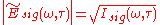
Frequency-resolved optical gating
Encyclopedia
In optics, frequency-resolved optical gating (FROG) is a derivative of autocorrelation
, but is far superior in its ability to measure ultrafast optical pulse
shapes. Further, it can determine the phase of the pulse.
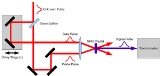
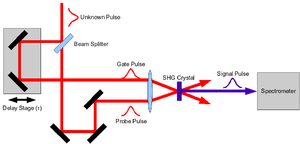
In the most common configuration, FROG is simply a background-free autocorrelator followed by a spectrometer.
It is the two-dimensional nature of the FROG trace that allows the extraction of the actual pulse shape and phase from the data.
Mathematically the FROG trace is simply a spectrogram
but with an unknown gate function:
where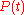
is the "probe" pulse and 
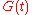is the "gate" pulse.
The probe and gate pulses are determined by the nonlinear interaction used, and
it is the form of the probe and gate that distinguishes the different types of FROG from each other.
Some of the most common are:
For example second-harmonic generation FROG (SHG FROG) would be:
and PG FROG would be:
Traditional inversion algorithms for spectrograms requires perfect knowledge of the gate function (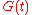
), however, FROG does not have this luxury.
Instead an iterative alogrithm is used.
The algorithm uses both the data (FROG trace) and form of the nonlinearity to achieve a best match between the real FROG trace and the "retrieved" FROG trace.
The retrieved FROG trace is created synthetically from the best guess for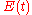
.
The FROG algorithm is all about phase retrieval.
The FROG trace measured in the lab is the exact intensity of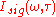
; however, it is missing the phase information.
To start off with define a signal field:
and further defining:
given this the FROG trace becomes:
inverting this:
so the amplitude of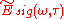
is known, but not its phase.
If this phase is found, then the pulse (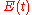
) can be retrieved.
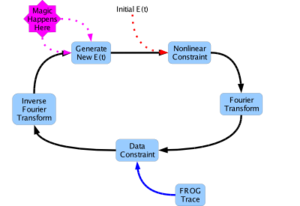
An iterative algorithm is used to determine this unknown phase.
A reply to L. Xu, D. J. Kane, and R. Trebino, "Amplitude ambiguities in second-harmonic-generation frequency-resolved optical gating: comment," Opt. Lett. 34, 2602-2602 (2009) is given in B. Yellampalle, K. Kim, and A. J. Taylor, "Amplitude ambiguities in second-harmonic-generation frequency-resolved optical gating: reply to comment," Opt. Lett. 34, 2603-2603 (2009). In their reply
Yellampalle et al. insist in their concern and remind the readership that the "FROG algorithms do not produce correct results nearly 20% of the time for complex or multiple pulses."
Also, researchers are actively working on simpler more direct methods to determine the pulse shape of femtosecond and attosecond lasers, see for example the work of J. Gagnon and V. S. Yakolev, Appl. Phys. B 103, 303-309 (2011).
Optical autocorrelation
In optics, various autocorrelation functions can be experimentally realized. The field autocorrelation may be used to calculate the spectrum of a source of light, while the intensity autocorrelation and the interferometric autocorrelation are commonly used to estimate the duration of ultrashort...
, but is far superior in its ability to measure ultrafast optical pulse
Ultrashort pulse
In optics, an ultrashort pulse of light is an electromagnetic pulse whose time duration is of the order of a femtosecond . Such pulses have a broadband optical spectrum, and can be created by mode-locked oscillators...
shapes. Further, it can determine the phase of the pulse.
In the most common configuration, FROG is simply a background-free autocorrelator followed by a spectrometer.
It is the two-dimensional nature of the FROG trace that allows the extraction of the actual pulse shape and phase from the data.
The basics
Frequency-Resolved refers to the fact that the final signal is a spectrum. Before explaining Optical Gating, it helps to recognize that the pulse is really interacting with itself. In most configurations, the pulse is split and recombined, as in an interferometer. However, in this case, the recombination does not occur on a beam-splitter, but rather in a nonlinear medium, which allows the two beams to interact with each other. It is this interaction that allows the pulses to "gate" the spectral information of the other pulse. So Optical Gating refers to the fact that the measured spectrum is really from a time-slice of the pulse, and that time-slice is determined by the pulse nonlinear interaction. The gate function depends on the type of nonlinear interaction allowed.Mathematically the FROG trace is simply a spectrogram
Spectrogram
A spectrogram is a time-varying spectral representation that shows how the spectral density of a signal varies with time. Also known as spectral waterfalls, sonograms, voiceprints, or voicegrams, spectrograms are used to identify phonetic sounds, to analyse the cries of animals; they were also...
but with an unknown gate function:
where
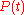is the "probe" pulse and 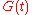is the "gate" pulse.The probe and gate pulses are determined by the nonlinear interaction used, and
it is the form of the probe and gate that distinguishes the different types of FROG from each other.
Some of the most common are:
 |
 |
: Second-harmonic generation FROG |
 |
: Polarization gating FROG | |
 |
 |
: Self-diffraction FROG |
 |
 |
: Third-harmonic generation FROG |
and PG FROG would be:
Traditional inversion algorithms for spectrograms requires perfect knowledge of the gate function (
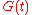), however, FROG does not have this luxury.Instead an iterative alogrithm is used.
The algorithm uses both the data (FROG trace) and form of the nonlinearity to achieve a best match between the real FROG trace and the "retrieved" FROG trace.
The retrieved FROG trace is created synthetically from the best guess for
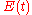.FROG algorithm
The FROG trace measured in the lab is the exact intensity of
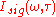; however, it is missing the phase information.To start off with define a signal field:
and further defining:
given this the FROG trace becomes:
inverting this:
so the amplitude of
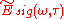is known, but not its phase.If this phase is found, then the pulse (
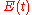) can be retrieved.An iterative algorithm is used to determine this unknown phase.
- Start with an initial guess for
. - Synthetically create
from 
. - Fourier transformFourier transformIn mathematics, Fourier analysis is a subject area which grew from the study of Fourier series. The subject began with the study of the way general functions may be represented by sums of simpler trigonometric functions...
the time axis to the frequency domain, yielding
. - Compare
to 
(generally the rmsRoot mean squareIn mathematics, the root mean square , also known as the quadratic mean, is a statistical measure of the magnitude of a varying quantity. It is especially useful when variates are positive and negative, e.g., sinusoids...
difference). If this error (termed the "G" error) is sufficiently small, exit the loop. - Replace the amplitude (preserving the phase) of
with the amplitude measured in the lab (
). Call this 
. - Take
and inverse Fourier transformFourier transformIn mathematics, Fourier analysis is a subject area which grew from the study of Fourier series. The subject began with the study of the way general functions may be represented by sums of simpler trigonometric functions...
it back into the time domain (
). - Extract the best
from 
. - GOTO 2.
FROG techniques
- Grating-eliminated no-nonsense observation of ultrafast incident laser light e-fields (GRENOUILLE), a simplified version of FROG
Competing techniques
- Spectral phase interferometry for direct electric-field reconstructionSpectral phase interferometry for direct electric-field reconstructionIn ultrafast optics, spectral phase interferometry for direct electric-field reconstruction is an ultrashort pulse measurement technique.-The basics:...
(SPIDER) - Streak cameraStreak cameraA streak camera is an instrument for measuring the variation in a pulse of light's intensity with time. They are used to measure the pulse duration of some ultrafast laser systems, and for applications such as time-resolved spectroscopy and LIDAR....
— not a significant competitor. Streak cameras have picosecond response times. - Multiphoton intrapulse interference phase scanMultiphoton intrapulse interference phase scanMultiphoton intrapulse interference phase scan is a method used in ultrashort laser technology that simultaneously measures , and compensates femtosecond laser pulses using an adaptive pulse shaper....
(MIIPS), a method to characterize and manipulate the ultrashort pulse. - Frequency-resolved electro-absorption gating (FREAG)
Controversy
In their 2007 paper, "Amplitude ambiguities in second-harmonic generation frequency-resolved optical gating", B. Yellampalle, K. Y. Kim, and A. J. Taylor, in Optics Letters, Vol. 32, Issue 24, pp. 3558–3560. Yellampalle et al. raised concerns (shown later to be in error) about FROG. Trebino found a calculation error that was a fatal flaw in their paper, and sought to have his clarification published as a comment in the journal. However, Trebino had some challenges in getting his response to the paper published as a comment, a saga he relates with much endurance and some humor in "How to Publish a Scientific Comment in 123 Easy Steps" http://www.physics.gatech.edu/frog/How%20to%20Publish%20a%20Comment%20w%20suggestions.pdfA reply to L. Xu, D. J. Kane, and R. Trebino, "Amplitude ambiguities in second-harmonic-generation frequency-resolved optical gating: comment," Opt. Lett. 34, 2602-2602 (2009) is given in B. Yellampalle, K. Kim, and A. J. Taylor, "Amplitude ambiguities in second-harmonic-generation frequency-resolved optical gating: reply to comment," Opt. Lett. 34, 2603-2603 (2009). In their reply
Yellampalle et al. insist in their concern and remind the readership that the "FROG algorithms do not produce correct results nearly 20% of the time for complex or multiple pulses."
Also, researchers are actively working on simpler more direct methods to determine the pulse shape of femtosecond and attosecond lasers, see for example the work of J. Gagnon and V. S. Yakolev, Appl. Phys. B 103, 303-309 (2011).
External links
- FROG Page by Rick Trebino (co-inventor of FROG)