Harry Kesten
Encyclopedia
Harry Kesten is an American mathematician
best known for his work in probability
, most notably on random walks and percolation theory
.
, where he moved with his parents in 1933 to escape the Nazis. He received his Ph.D. in 1956 at Cornell University
under supervision of Mark Kac
. He was an instructor at Princeton University
and the Hebrew University before returning to Cornell where he is now professor emeritus
of mathematics.
Kesten was a Guggenheim fellow
, Alfred P. Sloan Fellow
, received the Brouwer Medal, the
Steele Prize for Lifetime Achievement from the AMS
, and the George Pólya Prize
from the SIAM
. He is also a member of the National Academy of Sciences
and a correspondent member of the Royal Dutch Academy of Sciences.
Mathematician
A mathematician is a person whose primary area of study is the field of mathematics. Mathematicians are concerned with quantity, structure, space, and change....
best known for his work in probability
Probability
Probability is ordinarily used to describe an attitude of mind towards some proposition of whose truth we arenot certain. The proposition of interest is usually of the form "Will a specific event occur?" The attitude of mind is of the form "How certain are we that the event will occur?" The...
, most notably on random walks and percolation theory
Percolation theory
In mathematics, percolation theory describes the behavior of connected clusters in a random graph. The applications of percolation theory to materials science and other domains are discussed in the article percolation.-Introduction:...
.
Biography
Kesten grew up in the NetherlandsNetherlands
The Netherlands is a constituent country of the Kingdom of the Netherlands, located mainly in North-West Europe and with several islands in the Caribbean. Mainland Netherlands borders the North Sea to the north and west, Belgium to the south, and Germany to the east, and shares maritime borders...
, where he moved with his parents in 1933 to escape the Nazis. He received his Ph.D. in 1956 at Cornell University
Cornell University
Cornell University is an Ivy League university located in Ithaca, New York, United States. It is a private land-grant university, receiving annual funding from the State of New York for certain educational missions...
under supervision of Mark Kac
Mark Kac
Mark Kac was a Polish mathematician. His main interest was probability theory. His question, "Can one hear the shape of a drum?" set off research into spectral theory, with the idea of understanding the extent to which the spectrum allows one to read back the geometry. Kac completed his Ph.D...
. He was an instructor at Princeton University
Princeton University
Princeton University is a private research university located in Princeton, New Jersey, United States. The school is one of the eight universities of the Ivy League, and is one of the nine Colonial Colleges founded before the American Revolution....
and the Hebrew University before returning to Cornell where he is now professor emeritus
Emeritus
Emeritus is a post-positive adjective that is used to designate a retired professor, bishop, or other professional or as a title. The female equivalent emerita is also sometimes used.-History:...
of mathematics.
Kesten was a Guggenheim fellow
Guggenheim Fellowship
Guggenheim Fellowships are American grants that have been awarded annually since 1925 by the John Simon Guggenheim Memorial Foundation to those "who have demonstrated exceptional capacity for productive scholarship or exceptional creative ability in the arts." Each year, the foundation makes...
, Alfred P. Sloan Fellow
Sloan Fellowship
The Sloan Research Fellowships are awarded annually by the Alfred P. Sloan Foundation since 1955 to "provide support and recognition to early-career scientists and scholars". This is distinct from the Sloan Fellows in business....
, received the Brouwer Medal, the
Steele Prize for Lifetime Achievement from the AMS
American Mathematical Society
The American Mathematical Society is an association of professional mathematicians dedicated to the interests of mathematical research and scholarship, which it does with various publications and conferences as well as annual monetary awards and prizes to mathematicians.The society is one of the...
, and the George Pólya Prize
Pólya Prize (SIAM)
The Pólya Prize is a prize in mathematics, awarded by the Society for Industrial and Applied Mathematics. First given in 1969, the prize is named after Hungarian mathematician George Pólya...
from the SIAM
Society for Industrial and Applied Mathematics
The Society for Industrial and Applied Mathematics was founded by a small group of mathematicians from academia and industry who met in Philadelphia in 1951 to start an organization whose members would meet periodically to exchange ideas about the uses of mathematics in industry. This meeting led...
. He is also a member of the National Academy of Sciences
United States National Academy of Sciences
The National Academy of Sciences is a corporation in the United States whose members serve pro bono as "advisers to the nation on science, engineering, and medicine." As a national academy, new members of the organization are elected annually by current members, based on their distinguished and...
and a correspondent member of the Royal Dutch Academy of Sciences.
Mathematical work
Kesten's work includes a number of fundamental contributions, including the following.- Showing that amenability is equivalent to zero spectral gap and that a regular tree of degree
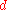has spectral radius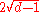 
- His work with Hillel Furstenberg on products of random matrices
- Enumerations of self-avoiding walkSelf-avoiding walkIn mathematics, a self-avoiding walk is a sequence of moves on a lattice that does not visit the same point more than once. A self-avoiding polygon is a closed self-avoiding walk on a lattice...
s and a "pattern theorem". - Resolving a conjecture of ErdősPaul ErdosPaul Erdős was a Hungarian mathematician. Erdős published more papers than any other mathematician in history, working with hundreds of collaborators. He worked on problems in combinatorics, graph theory, number theory, classical analysis, approximation theory, set theory, and probability theory...
and Szusz on the discrepancy of irrational rotations - Kesten did much work on percolationPercolationIn physics, chemistry and materials science, percolation concerns the movement and filtering of fluids through porous materials...
including proving that the critical probability for 2 dimensional percolationPercolationIn physics, chemistry and materials science, percolation concerns the movement and filtering of fluids through porous materials...
is one-half, demonstrating the so-called scaling relations and writing the first book on percolation directed towards mathematicians. - Giving an upper bound for the growth of arms in diffusion-limited aggregationDiffusion-limited aggregationDiffusion-limited aggregation is the process whereby particles undergoing a random walk due to Brownian motion cluster together to form aggregates of such particles. This theory, proposed by Witten and Sander in 1981, is applicable to aggregation in any system where diffusion is the primary means...
- His work with Vladas Sidoravicius on the spread of an infection in a moving population