
Hilbert modular form
Encyclopedia
In mathematics
, a Hilbert modular form is a generalization of modular form
s to functions of two or more variables.
It is a (complex) analytic function
on the m-fold product of upper half-planes
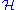 satisfying a certain kind of functional equation
satisfying a certain kind of functional equation
.
Let F be a totally real number field
of degree m over rational field. Let

be the real embeddings of F. Through them
we have a map
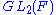 →
→ 
Let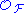 be the ring of integers
be the ring of integers
of F. The group
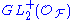 is called the full Hilbert modular group.
is called the full Hilbert modular group.
For every element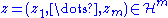 ,
,
there is a group action of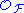 defined by
defined by
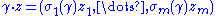
For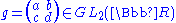 , define
, define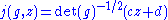
A Hilbert modular form of weight is an analytic function on
is an analytic function on
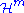 such that for every
such that for every 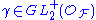
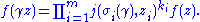
Unlike the modular form case, no extra condition is needed for the cusps because of Koecher's principle.
. There he mentions that David Hilbert
had considered them initially in work from 1893-4, which remained unpublished. Blumenthal's work was published in 1903. For this reason Hilbert modular forms are now often called Hilbert-Blumenthal modular forms.
The theory remained dormant for some decades; Erich Hecke
appealed to it in his early work, but major interest in Hilbert modular forms awaited the development of complex manifold
theory.
Mathematics
Mathematics is the study of quantity, space, structure, and change. Mathematicians seek out patterns and formulate new conjectures. Mathematicians resolve the truth or falsity of conjectures by mathematical proofs, which are arguments sufficient to convince other mathematicians of their validity...
, a Hilbert modular form is a generalization of modular form
Modular form
In mathematics, a modular form is a analytic function on the upper half-plane satisfying a certain kind of functional equation and growth condition. The theory of modular forms therefore belongs to complex analysis but the main importance of the theory has traditionally been in its connections...
s to functions of two or more variables.
It is a (complex) analytic function
Analytic function
In mathematics, an analytic function is a function that is locally given by a convergent power series. There exist both real analytic functions and complex analytic functions, categories that are similar in some ways, but different in others...
on the m-fold product of upper half-planes
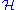 satisfying a certain kind of functional equation
satisfying a certain kind of functional equationFunctional equation
In mathematics, a functional equation is any equation that specifies a function in implicit form.Often, the equation relates the value of a function at some point with its values at other points. For instance, properties of functions can be determined by considering the types of functional...
.
Let F be a totally real number field
Totally real number field
In number theory, a number field K is called totally real if for each embedding of K into the complex numbers the image lies inside the real numbers...
of degree m over rational field. Let

be the real embeddings of F. Through them
we have a map
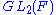 →
→ 
Let
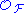 be the ring of integers
be the ring of integersRing of integers
In mathematics, the ring of integers is the set of integers making an algebraic structure Z with the operations of integer addition, negation, and multiplication...
of F. The group
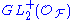 is called the full Hilbert modular group.
is called the full Hilbert modular group.For every element
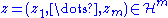 ,
,there is a group action of
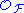 defined by
defined by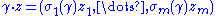
For
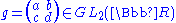 , define
, define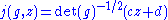
A Hilbert modular form of weight
 is an analytic function on
is an analytic function on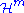 such that for every
such that for every 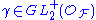
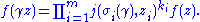
Unlike the modular form case, no extra condition is needed for the cusps because of Koecher's principle.
History
These modular forms, for real quadratic fields, were first treated in the 1901 Göttingen University Habilitationssschrift of Otto BlumenthalOtto Blumenthal
Ludwig Otto Blumenthal was a German mathematician and professor at RWTH Aachen University. He was born in Frankfurt, Prussia...
. There he mentions that David Hilbert
David Hilbert
David Hilbert was a German mathematician. He is recognized as one of the most influential and universal mathematicians of the 19th and early 20th centuries. Hilbert discovered and developed a broad range of fundamental ideas in many areas, including invariant theory and the axiomatization of...
had considered them initially in work from 1893-4, which remained unpublished. Blumenthal's work was published in 1903. For this reason Hilbert modular forms are now often called Hilbert-Blumenthal modular forms.
The theory remained dormant for some decades; Erich Hecke
Erich Hecke
Erich Hecke was a German mathematician. He obtained his doctorate in Göttingen under the supervision of David Hilbert. Kurt Reidemeister and Heinrich Behnke were among his students....
appealed to it in his early work, but major interest in Hilbert modular forms awaited the development of complex manifold
Complex manifold
In differential geometry, a complex manifold is a manifold with an atlas of charts to the open unit disk in Cn, such that the transition maps are holomorphic....
theory.

