Hodrick-Prescott filter
Encyclopedia
The Hodrick–Prescott filter is a mathematical tool used in macroeconomics
, especially in real business cycle theory to separate the cyclical component of a time series from raw data. It is used to obtain a smoothed-curve representation of a time series
, one that is more sensitive to long-term than to short-term fluctuations. The adjustment of the sensitivity of the trend to short-term fluctuations is achieved by modifying a multiplier
. The filter was first applied by economists Robert J. Hodrick
and Nobel Prize(2004) winner Edward C. Prescott
. Though Hodrick and Prescott popularized the filter in the field of economics, it was first proposed by E. T. Whittaker (Whittaker, E. T. (1923). On a new method of graduation, Proceedings of the Edinburgh Mathematical Association, 78, 81-89.)
for 
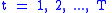denote the logarithms of a time series variable. The series 
is made up of a trend component, denoted by 
and a cyclical component, denoted by 
such that 
. Given an adequately chosen, positive value of 
, there is a trend component that will minimize
The first term of the equation is the sum of the squared deviations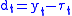
which penalizes the cyclical component. The second term is a multiple 
of the sum of the squares of the trend component's second differences. This second term penalizes variations in the growth rate of the trend component. The larger the value of 
, the higher is the penalty. Hodrick and Prescott advise that, for quarterly data, a value of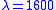 
is reasonable.
Macroeconomics
Macroeconomics is a branch of economics dealing with the performance, structure, behavior, and decision-making of the whole economy. This includes a national, regional, or global economy...
, especially in real business cycle theory to separate the cyclical component of a time series from raw data. It is used to obtain a smoothed-curve representation of a time series
Time series
In statistics, signal processing, econometrics and mathematical finance, a time series is a sequence of data points, measured typically at successive times spaced at uniform time intervals. Examples of time series are the daily closing value of the Dow Jones index or the annual flow volume of the...
, one that is more sensitive to long-term than to short-term fluctuations. The adjustment of the sensitivity of the trend to short-term fluctuations is achieved by modifying a multiplier
. The filter was first applied by economists Robert J. HodrickRobert J. Hodrick
Robert James Hodrick , is a U.S. economistspecialized in International Finance. AB, Princeton, 1972; PhD, University of Chicago, 1976....
and Nobel Prize(2004) winner Edward C. Prescott
Edward C. Prescott
Edward Christian Prescott is an American economist. He received the Nobel Memorial Prize in Economics in 2004, sharing the award with Finn E. Kydland, "for their contributions to dynamic macroeconomics: the time consistency of economic policy and the driving forces behind business cycles"...
. Though Hodrick and Prescott popularized the filter in the field of economics, it was first proposed by E. T. Whittaker (Whittaker, E. T. (1923). On a new method of graduation, Proceedings of the Edinburgh Mathematical Association, 78, 81-89.)
The equation
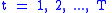The reasoning for the methodology uses ideas related to the decomposition of time series. Let for denote the logarithms of a time series variable. The series is made up of a trend component, denoted by and a cyclical component, denoted by such that . Given an adequately chosen, positive value of , there is a trend component that will minimize- min
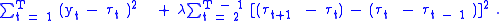
The first term of the equation is the sum of the squared deviations
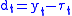which penalizes the cyclical component. The second term is a multiple of the sum of the squares of the trend component's second differences. This second term penalizes variations in the growth rate of the trend component. The larger the value of , the higher is the penalty. Hodrick and Prescott advise that, for quarterly data, a value of 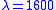is reasonable.Drawbacks to the H–P filter
The Hodrick–Prescott filter will only be optimal when:- Data exists in a I(2) trend.
- If one-time permanent shocks or split growth rates occur, the filter will generate shifts in the trend that do not actually exist.
- Noise in data is approximately normally distributed.
- Analysis is purely historical and static (closed domain). The filter has misleading predictive outcome when used dynamically since the algorithm changes (during iteration for minimization) the past state (unlike a moving average) of the time series to adjust for the current state regardless of the size of
used.