Johnson–Nyquist noise
Encyclopedia
Johnson–Nyquist noise is the electronic
noise generated by the thermal agitation of the charge carriers (usually the electron
s) inside an electrical conductor
at equilibrium, which happens regardless of any applied voltage
. The generic, statistical physical derivation of this noise is called the fluctuation-dissipation theorem, where generalized impedance
or generalized susceptibility
is used to characterize the medium.
Thermal noise in an idealistic resistor is approximately white
, meaning that the power spectral density
is nearly constant throughout the frequency spectrum
(however see the section below on extremely high frequencies). Additionally, the amplitude of the signal has very nearly a Gaussian probability density function
.
at Bell Labs
in 1926. He described his findings to Harry Nyquist
, also at Bell Labs, who was able to explain the results.
, which consists of additional current fluctuations that occur when a voltage is applied and a macroscopic current starts to flow. For the general case, the above definition applies to charge carriers in any type of conducting medium
(e.g. ion
s in an electrolyte
), not just resistor
s. It can be modeled by a voltage source representing the noise of the non-ideal resistor in series with an ideal noise free resistor.
The one-sided power spectral density
, or voltage variance (mean square) per hertz
of bandwidth, is given by
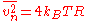
where kB is Boltzmann's constant in joule
s per kelvin
, T is the resistor's absolute temperature
in kelvins, and R is the resistor value in ohm
s (Ω).
Use this equation for quick calculation, at room temperature:
For example, a 1 kΩ resistor at a temperature of 300 K has
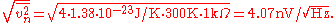
For a given bandwidth, the root mean square
(RMS) of the voltage,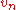
, is given by
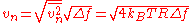
where Δf is the bandwidth in hertz over which the noise is measured. For a 1 kΩ resistor at room temperature and a 10 kHz bandwidth, the RMS noise voltage is 400 nV. A useful rule of thumb to remember is that 50 Ω at 1 Hz bandwidth correspond to 1 nV noise at room temperature.
A resistor in a short circuit dissipates a noise power of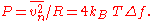
The noise generated at the resistor can transfer to the remaining circuit; the maximum noise power transfer happens with impedance matching
when the Thévenin equivalent resistance of the remaining circuit is equal to the noise generating resistance. In this case each one of the two participating resistors dissipates noise in both itself and in the other resistor. Since only half of the source voltage drops across any one of these resistors, the resulting noise power is given by
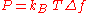
where P is the thermal noise power in watts. Notice that this is independent of the noise generating resistance.
value of the current source as:
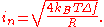
Thermal noise is intrinsic to all resistors and is not a sign of poor design or manufacture, although resistors may also have excess noise.
(decibels relative to 1 milliwatt). From the equation above, noise power in a resistor at room temperature
, in dBm, is then:
where the factor of 1000 is present because the power is given in milliwatts, rather than watts. This equation can be simplified by separating the constant parts from the bandwidth:
which is more commonly seen approximated as:
Noise power at different bandwidths is then simple to calculate:
! Bandwidth
!! Thermal noise power !! Notes
|-
| 1 Hz || −174 dBm ||
|-
| 10 Hz || −164 dBm
|-
| 100 Hz || −154 dBm
|-
| 1 kHz || −144 dBm
|-
| 10 kHz || −134 dBm || FM
channel of 2-way radio
|-
| 100 kHz || −124 dBm
|-
| 180 kHz || −121.45 dBm || One LTE
resource block
|-
| 200 kHz || −120.98 dBm || One GSM channel (ARFCN)
|-
| 1 MHz || −114 dBm
|-
| 2 MHz || −111 dBm || Commercial GPS
channel
|-
| 6 MHz || −106 dBm || Analog television
channel
|-
| 20 MHz || −101 dBm || WLAN 802.11
channel
|}
has an unusually simple expression, as the value of the resistance
(R) drops out of the equation. This is because higher R contributes to more filtering as well as to more noise. The noise bandwidth of the RC circuit is 1/(4RC), which can substituted into the above formula to eliminate R. The mean-square and RMS noise voltage generated in such a filter are:
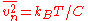
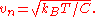
Thermal noise accounts for 100% of kTC noise, whether it is attributed to the resistance or to the capacitance
.
In the extreme case of the reset noise left on a capacitor by opening an ideal switch, the resistance is infinite, yet the formula still applies; however, now the RMS must be interpreted not as a time average, but as an average over many such reset events, since the voltage is constant when the bandwidth is zero. In this sense, the Johnson noise of an RC circuit can be seen to be inherent, an effect of the thermodynamic distribution of the number of electrons on the capacitor, even without the involvement of a resistor.
The noise is not caused by the capacitor itself, but by the thermodynamic equilibrium
of the amount of charge on the capacitor. Once the capacitor is disconnected from a conducting circuit, the thermodynamic fluctuation is frozen at a random value with standard deviation
as given above.
The reset noise of capacitive sensors is often a limiting noise source, for example in image sensor
s. As an alternative to the voltage noise, the reset noise on the capacitor can also be quantified as the electrical charge standard deviation, as
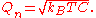
Since the charge variance is
, this noise is often called kTC noise.
Any system in thermal equilibrium
has state variable
s with a mean energy
of kT/2 per degree of freedom
. Using the formula for energy on a capacitor (E = ½CV2), mean noise energy on a capacitor can be seen to also be ½C(kT/C), or also kT/2. Thermal noise on a capacitor can be derived from this relationship, without consideration of resistance.
The kTC noise is the dominant noise source at small capacitors.
|+ Noise of capacitors at 300 K
! Capacitance !!
!! Electrons
|-
| 1 fF || 2 mV || 12.5 e–
|-
| 10 fF || 640 µV || 40 e–
|-
| 100 fF || 200 µV || 125 e–
|-
| 1 pF || 64 µV || 400 e–
|-
| 10 pF || 20 µV || 1250 e–
|-
| 100 pF || 6.4 µV || 4000 e–
|-
| 1 nF || 2 µV || 12500 e–
|}
of the voltage across the resistor R, in V2/Hz is given by:
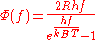
where f is the frequency, h Planck's constant
, kB Boltzmann constant and T the temperature in kelvins.
If the frequency is low enough, that means:
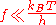
(this assumption is valid until few terahertz at room temperature) then the exponential can be expressed in terms of its Taylor series
. The relationship then becomes:
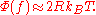
In general, both R and T depend on frequency. In order to know the total noise it is enough to integrate over all the bandwidth. Since the signal is real, it is possible to integrate over only the positive frequencies, then multiply by 2.
Assuming that R and T are constants over all the bandwidth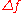
, then the root mean square
(RMS) value of the voltage across a resistor due to thermal noise is given by
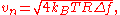
that is, the same formula as above.
Electronic noise
Electronic noise is a random fluctuation in an electrical signal, a characteristic of all electronic circuits. Noise generated by electronic devices varies greatly, as it can be produced by several different effects...
noise generated by the thermal agitation of the charge carriers (usually the electron
Electron
The electron is a subatomic particle with a negative elementary electric charge. It has no known components or substructure; in other words, it is generally thought to be an elementary particle. An electron has a mass that is approximately 1/1836 that of the proton...
s) inside an electrical conductor
Electrical conductor
In physics and electrical engineering, a conductor is a material which contains movable electric charges. In metallic conductors such as copper or aluminum, the movable charged particles are electrons...
at equilibrium, which happens regardless of any applied voltage
Voltage
Voltage, otherwise known as electrical potential difference or electric tension is the difference in electric potential between two points — or the difference in electric potential energy per unit charge between two points...
. The generic, statistical physical derivation of this noise is called the fluctuation-dissipation theorem, where generalized impedance
Impedance
Impedance may refer to:*Electrical impedance, the ratio of the voltage phasor to the electric current phasor, a measure of the opposition to time-varying electric current in an electric circuit**Characteristic impedance of a transmission line...
or generalized susceptibility
Susceptibility
*In physics, the susceptibility of a material or substance describes its response to an applied field. There are many kinds of susceptibilities, for example:These two susceptibilities are particular examples of a linear response function;...
is used to characterize the medium.
Thermal noise in an idealistic resistor is approximately white
White noise
White noise is a random signal with a flat power spectral density. In other words, the signal contains equal power within a fixed bandwidth at any center frequency...
, meaning that the power spectral density
Spectral density
In statistical signal processing and physics, the spectral density, power spectral density , or energy spectral density , is a positive real function of a frequency variable associated with a stationary stochastic process, or a deterministic function of time, which has dimensions of power per hertz...
is nearly constant throughout the frequency spectrum
Frequency spectrum
The frequency spectrum of a time-domain signal is a representation of that signal in the frequency domain. The frequency spectrum can be generated via a Fourier transform of the signal, and the resulting values are usually presented as amplitude and phase, both plotted versus frequency.Any signal...
(however see the section below on extremely high frequencies). Additionally, the amplitude of the signal has very nearly a Gaussian probability density function
Probability density function
In probability theory, a probability density function , or density of a continuous random variable is a function that describes the relative likelihood for this random variable to occur at a given point. The probability for the random variable to fall within a particular region is given by the...
.
History
This type of noise was first measured by John B. JohnsonJohn B. Johnson
John Bertrand "Bert" Johnson was a Swedish-born American electrical engineer and physicist...
at Bell Labs
Bell Labs
Bell Laboratories is the research and development subsidiary of the French-owned Alcatel-Lucent and previously of the American Telephone & Telegraph Company , half-owned through its Western Electric manufacturing subsidiary.Bell Laboratories operates its...
in 1926. He described his findings to Harry Nyquist
Harry Nyquist
Harry Nyquist was an important contributor to information theory.-Personal life:...
, also at Bell Labs, who was able to explain the results.
Noise voltage and power
Thermal noise is distinct from shot noiseShot noise
Shot noise is a type of electronic noise that may be dominant when the finite number of particles that carry energy is sufficiently small so that uncertainties due to the Poisson distribution, which describes the occurrence of independent random events, are of significance...
, which consists of additional current fluctuations that occur when a voltage is applied and a macroscopic current starts to flow. For the general case, the above definition applies to charge carriers in any type of conducting medium
Transmission medium
A transmission medium is a material substance that can propagate energy waves...
(e.g. ion
Ion
An ion is an atom or molecule in which the total number of electrons is not equal to the total number of protons, giving it a net positive or negative electrical charge. The name was given by physicist Michael Faraday for the substances that allow a current to pass between electrodes in a...
s in an electrolyte
Electrolyte
In chemistry, an electrolyte is any substance containing free ions that make the substance electrically conductive. The most typical electrolyte is an ionic solution, but molten electrolytes and solid electrolytes are also possible....
), not just resistor
Resistor
A linear resistor is a linear, passive two-terminal electrical component that implements electrical resistance as a circuit element.The current through a resistor is in direct proportion to the voltage across the resistor's terminals. Thus, the ratio of the voltage applied across a resistor's...
s. It can be modeled by a voltage source representing the noise of the non-ideal resistor in series with an ideal noise free resistor.
The one-sided power spectral density
Spectral density
In statistical signal processing and physics, the spectral density, power spectral density , or energy spectral density , is a positive real function of a frequency variable associated with a stationary stochastic process, or a deterministic function of time, which has dimensions of power per hertz...
, or voltage variance (mean square) per hertz
Hertz
The hertz is the SI unit of frequency defined as the number of cycles per second of a periodic phenomenon. One of its most common uses is the description of the sine wave, particularly those used in radio and audio applications....
of bandwidth, is given by
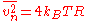
where kB is Boltzmann's constant in joule
Joule
The joule ; symbol J) is a derived unit of energy or work in the International System of Units. It is equal to the energy expended in applying a force of one newton through a distance of one metre , or in passing an electric current of one ampere through a resistance of one ohm for one second...
s per kelvin
Kelvin
The kelvin is a unit of measurement for temperature. It is one of the seven base units in the International System of Units and is assigned the unit symbol K. The Kelvin scale is an absolute, thermodynamic temperature scale using as its null point absolute zero, the temperature at which all...
, T is the resistor's absolute temperature
Temperature
Temperature is a physical property of matter that quantitatively expresses the common notions of hot and cold. Objects of low temperature are cold, while various degrees of higher temperatures are referred to as warm or hot...
in kelvins, and R is the resistor value in ohm
Ohm
The ohm is the SI unit of electrical resistance, named after German physicist Georg Simon Ohm.- Definition :The ohm is defined as a resistance between two points of a conductor when a constant potential difference of 1 volt, applied to these points, produces in the conductor a current of 1 ampere,...
s (Ω).
Use this equation for quick calculation, at room temperature:
For example, a 1 kΩ resistor at a temperature of 300 K has
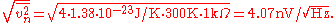
For a given bandwidth, the root mean square
Root mean square
In mathematics, the root mean square , also known as the quadratic mean, is a statistical measure of the magnitude of a varying quantity. It is especially useful when variates are positive and negative, e.g., sinusoids...
(RMS) of the voltage,
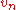, is given by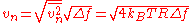where Δf is the bandwidth in hertz over which the noise is measured. For a 1 kΩ resistor at room temperature and a 10 kHz bandwidth, the RMS noise voltage is 400 nV. A useful rule of thumb to remember is that 50 Ω at 1 Hz bandwidth correspond to 1 nV noise at room temperature.
A resistor in a short circuit dissipates a noise power of
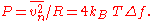
The noise generated at the resistor can transfer to the remaining circuit; the maximum noise power transfer happens with impedance matching
Impedance matching
In electronics, impedance matching is the practice of designing the input impedance of an electrical load to maximize the power transfer and/or minimize reflections from the load....
when the Thévenin equivalent resistance of the remaining circuit is equal to the noise generating resistance. In this case each one of the two participating resistors dissipates noise in both itself and in the other resistor. Since only half of the source voltage drops across any one of these resistors, the resulting noise power is given by
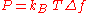
where P is the thermal noise power in watts. Notice that this is independent of the noise generating resistance.
Noise current
The noise source can also be modeled by a current source in parallel with the resistor by taking the Norton equivalent that corresponds simply to divide by R. This gives the root mean squareRoot mean square
In mathematics, the root mean square , also known as the quadratic mean, is a statistical measure of the magnitude of a varying quantity. It is especially useful when variates are positive and negative, e.g., sinusoids...
value of the current source as:
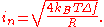
Thermal noise is intrinsic to all resistors and is not a sign of poor design or manufacture, although resistors may also have excess noise.
Noise power in decibels
Signal power is often measured in dBmDBm
dBm is an abbreviation for the power ratio in decibels of the measured power referenced to one milliwatt . It is used in radio, microwave and fiber optic networks as a convenient measure of absolute power because of its capability to express both very large and very small values in a short form...
(decibels relative to 1 milliwatt). From the equation above, noise power in a resistor at room temperature
Room temperature
-Comfort levels:The American Society of Heating, Refrigerating and Air-Conditioning Engineers has listings for suggested temperatures and air flow rates in different types of buildings and different environmental circumstances. For example, a single office in a building has an occupancy ratio per...
, in dBm, is then:
where the factor of 1000 is present because the power is given in milliwatts, rather than watts. This equation can be simplified by separating the constant parts from the bandwidth:
which is more commonly seen approximated as:
Noise power at different bandwidths is then simple to calculate:
-
- {| class="wikitable"
! Bandwidth
!! Thermal noise power !! Notes|-
| 1 Hz || −174 dBm ||
|-
| 10 Hz || −164 dBm
|-
| 100 Hz || −154 dBm
|-
| 1 kHz || −144 dBm
|-
| 10 kHz || −134 dBm || FM
Frequency modulation
In telecommunications and signal processing, frequency modulation conveys information over a carrier wave by varying its instantaneous frequency. This contrasts with amplitude modulation, in which the amplitude of the carrier is varied while its frequency remains constant...
channel of 2-way radio
Walkie-talkie
A walkie-talkie is a hand-held, portable, two-way radio transceiver. Its development during the Second World War has been variously credited to Donald L. Hings, radio engineer Alfred J. Gross, and engineering teams at Motorola...
|-
| 100 kHz || −124 dBm
|-
| 180 kHz || −121.45 dBm || One LTE
3GPP Long Term Evolution
3GPP Long Term Evolution, usually referred to as LTE, is a standard for wireless communication of high-speed data for mobile phones and data terminals. It is based on the GSM/EDGE and UMTS/HSPA network technologies, increasing the capacity and speed using new modulation techniques...
resource block
|-
| 200 kHz || −120.98 dBm || One GSM channel (ARFCN)
|-
| 1 MHz || −114 dBm
|-
| 2 MHz || −111 dBm || Commercial GPS
Global Positioning System
The Global Positioning System is a space-based global navigation satellite system that provides location and time information in all weather, anywhere on or near the Earth, where there is an unobstructed line of sight to four or more GPS satellites...
channel
|-
| 6 MHz || −106 dBm || Analog television
Analog television
Analog television is the analog transmission that involves the broadcasting of encoded analog audio and analog video signal: one in which the message conveyed by the broadcast signal is a function of deliberate variations in the amplitude and/or frequency of the signal...
channel
|-
| 20 MHz || −101 dBm || WLAN 802.11
IEEE 802.11
IEEE 802.11 is a set of standards for implementing wireless local area network computer communication in the 2.4, 3.6 and 5 GHz frequency bands. They are created and maintained by the IEEE LAN/MAN Standards Committee . The base version of the standard IEEE 802.11-2007 has had subsequent...
channel
|}
Thermal noise on capacitors
Thermal noise on capacitors is referred to as kTC noise. Thermal noise in an RC circuitRC circuit
A resistor–capacitor circuit ', or RC filter or RC network, is an electric circuit composed of resistors and capacitors driven by a voltage or current source...
has an unusually simple expression, as the value of the resistance
Electrical resistance
The electrical resistance of an electrical element is the opposition to the passage of an electric current through that element; the inverse quantity is electrical conductance, the ease at which an electric current passes. Electrical resistance shares some conceptual parallels with the mechanical...
(R) drops out of the equation. This is because higher R contributes to more filtering as well as to more noise. The noise bandwidth of the RC circuit is 1/(4RC), which can substituted into the above formula to eliminate R. The mean-square and RMS noise voltage generated in such a filter are:
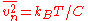
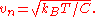
Thermal noise accounts for 100% of kTC noise, whether it is attributed to the resistance or to the capacitance
Capacitance
In electromagnetism and electronics, capacitance is the ability of a capacitor to store energy in an electric field. Capacitance is also a measure of the amount of electric potential energy stored for a given electric potential. A common form of energy storage device is a parallel-plate capacitor...
.
In the extreme case of the reset noise left on a capacitor by opening an ideal switch, the resistance is infinite, yet the formula still applies; however, now the RMS must be interpreted not as a time average, but as an average over many such reset events, since the voltage is constant when the bandwidth is zero. In this sense, the Johnson noise of an RC circuit can be seen to be inherent, an effect of the thermodynamic distribution of the number of electrons on the capacitor, even without the involvement of a resistor.
The noise is not caused by the capacitor itself, but by the thermodynamic equilibrium
Thermodynamic equilibrium
In thermodynamics, a thermodynamic system is said to be in thermodynamic equilibrium when it is in thermal equilibrium, mechanical equilibrium, radiative equilibrium, and chemical equilibrium. The word equilibrium means a state of balance...
of the amount of charge on the capacitor. Once the capacitor is disconnected from a conducting circuit, the thermodynamic fluctuation is frozen at a random value with standard deviation
Standard deviation
Standard deviation is a widely used measure of variability or diversity used in statistics and probability theory. It shows how much variation or "dispersion" there is from the average...
as given above.
The reset noise of capacitive sensors is often a limiting noise source, for example in image sensor
Image sensor
An image sensor is a device that converts an optical image into an electronic signal. It is used mostly in digital cameras and other imaging devices...
s. As an alternative to the voltage noise, the reset noise on the capacitor can also be quantified as the electrical charge standard deviation, as
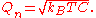
Since the charge variance is
, this noise is often called kTC noise.Any system in thermal equilibrium
Thermal equilibrium
Thermal equilibrium is a theoretical physical concept, used especially in theoretical texts, that means that all temperatures of interest are unchanging in time and uniform in space...
has state variable
State variable
A state variable is one of the set of variables that describe the "state" of a dynamical system. Intuitively, the state of a system describes enough about the system to determine its future behaviour...
s with a mean energy
Energy
In physics, energy is an indirectly observed quantity. It is often understood as the ability a physical system has to do work on other physical systems...
of kT/2 per degree of freedom
Degrees of freedom (physics and chemistry)
A degree of freedom is an independent physical parameter, often called a dimension, in the formal description of the state of a physical system...
. Using the formula for energy on a capacitor (E = ½CV2), mean noise energy on a capacitor can be seen to also be ½C(kT/C), or also kT/2. Thermal noise on a capacitor can be derived from this relationship, without consideration of resistance.
The kTC noise is the dominant noise source at small capacitors.
-
- {| class="wikitable"
|+ Noise of capacitors at 300 K
! Capacitance !!
!! Electrons|-
| 1 fF || 2 mV || 12.5 e–
|-
| 10 fF || 640 µV || 40 e–
|-
| 100 fF || 200 µV || 125 e–
|-
| 1 pF || 64 µV || 400 e–
|-
| 10 pF || 20 µV || 1250 e–
|-
| 100 pF || 6.4 µV || 4000 e–
|-
| 1 nF || 2 µV || 12500 e–
|}
Noise at very high frequencies
The above equations are good approximations at any practical radio frequency in use (i.e. frequencies below about 80 gigahertz). In the most general case, which includes up to optical frequencies, the power spectral densitySpectral density
In statistical signal processing and physics, the spectral density, power spectral density , or energy spectral density , is a positive real function of a frequency variable associated with a stationary stochastic process, or a deterministic function of time, which has dimensions of power per hertz...
of the voltage across the resistor R, in V2/Hz is given by:
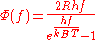
where f is the frequency, h Planck's constant
Planck constant
The Planck constant , also called Planck's constant, is a physical constant reflecting the sizes of energy quanta in quantum mechanics. It is named after Max Planck, one of the founders of quantum theory, who discovered it in 1899...
, kB Boltzmann constant and T the temperature in kelvins.
If the frequency is low enough, that means:
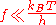
(this assumption is valid until few terahertz at room temperature) then the exponential can be expressed in terms of its Taylor series
Taylor series
In mathematics, a Taylor series is a representation of a function as an infinite sum of terms that are calculated from the values of the function's derivatives at a single point....
. The relationship then becomes:
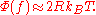
In general, both R and T depend on frequency. In order to know the total noise it is enough to integrate over all the bandwidth. Since the signal is real, it is possible to integrate over only the positive frequencies, then multiply by 2.
Assuming that R and T are constants over all the bandwidth
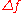, then the root mean squareRoot mean square
In mathematics, the root mean square , also known as the quadratic mean, is a statistical measure of the magnitude of a varying quantity. It is especially useful when variates are positive and negative, e.g., sinusoids...
(RMS) value of the voltage across a resistor due to thermal noise is given by
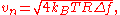
that is, the same formula as above.
See also
- Fluctuation-dissipation theorem
- Shot noiseShot noiseShot noise is a type of electronic noise that may be dominant when the finite number of particles that carry energy is sufficiently small so that uncertainties due to the Poisson distribution, which describes the occurrence of independent random events, are of significance...
- 1/f noise
- Langevin equationLangevin equationIn statistical physics, a Langevin equation is a stochastic differential equation describing the time evolution of a subset of the degrees of freedom. These degrees of freedom typically are collective variables changing only slowly in comparison to the other variables of the system...
External links
- Amplifier noise in RF systems
- Thermal noise (undergraduate) with detailed math
- Johnson-Nyquist noise or thermal noise calculator — volts and dB
- Thoughts about Image Calibration for low dark current and Amateur CCD Cameras to increase Signal-To-Noise Ratio
- Derivation of the Nyquist relation using a random electric field, H. Sonoda