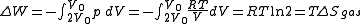The Joule expansion is an irreversible process in thermodynamics

Thermodynamics

Thermodynamics is a physical science that studies the effects on material bodies, and on radiation in regions of space, of transfer of heat and of work done on or by the bodies or radiation...

 in which a volume of gas is kept in one side of a thermally isolated container (via a small partition), with the other side of the container being evacuated; the partition between the two parts of the container is then opened, and the gas fills the whole container. This process is a useful exercise in classical thermodynamics, as it is easy to work out the resulting increase in entropy

Entropy

Entropy is a thermodynamic property that can be used to determine the energy available for useful work in a thermodynamic process, such as in energy conversion devices, engines, or machines. Such devices can only be driven by convertible energy, and have a theoretical maximum efficiency when...

.

Description

One mole

Mole (unit)

The mole is a unit of measurement used in chemistry to express amounts of a chemical substance, defined as an amount of a substance that contains as many elementary entities as there are atoms in 12 grams of pure carbon-12 , the isotope of carbon with atomic weight 12. This corresponds to a value...

 of ideal gas (at pressure 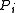and temperature 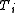) is confined to the left-hand side (as drawn) of a thermally isolated container, and occupies a volume 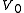. The right hand side of the container, also containing a volume , is evacuated. The tap (solid line) between the two halves of the container is then suddenly opened and the gas fills the entire container, of volume . We propose that both the previous and new temperature and pressure (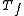, 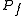) follow the Ideal Gas Law

Ideal gas law

The ideal gas law is the equation of state of a hypothetical ideal gas. It is a good approximation to the behavior of many gases under many conditions, although it has several limitations. It was first stated by Émile Clapeyron in 1834 as a combination of Boyle's law and Charles's law...

, so that initially we have 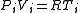and then, when the tap is opened, we have 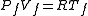, where is the ideal gas constant.

As the system is thermally isolated, it cannot exchange heat with its surroundings. Also, since the system's volume is kept constant, the system does not do work on its surroundings. As a result, the change in internal energy

Internal energy

In thermodynamics, the internal energy is the total energy contained by a thermodynamic system. It is the energy needed to create the system, but excludes the energy to displace the system's surroundings, any energy associated with a move as a whole, or due to external force fields. Internal...

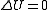 , and because the internal energy is a function of temperature only for the ideal gas, we know that 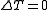also, and so 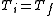. This implies that 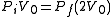, and thus the pressure halves; i.e. .

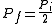Entropy changes

It is awkward to calculate the change in entropy in this process directly, because the route that the expansion takes in the time between the partition being opened and the equilibrium state being reached involves states that are far from thermal equilibrium and would require specifying the complete velocity distribution of the molecules to be defined. However, entropy is a function of state, and therefore the entropy change can be computed directly from the knowledge of the final and initial equilibrium states. For an ideal monatomic gas

Monatomic gas

In physics and chemistry, monatomic is a combination of the words "mono" and "atomic," and means "single atom." It is usually applied to gases: a monatomic gas is one in which atoms are not bound to each other....

, the entropy as a function of the internal energy, volume and number of particles is given by the Sackur–Tetrode equation:


It is seen that a doubling of the volume at constant internal energy will lead to an entropy increase of:


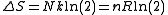
This result is also valid if the gas is not monatomic, as the volume dependence of an ideal gas in the dilute limit (in which classical statistical mechanics correctly describes the translational degrees of freedom) is the same as for a monatomic gas.

One can also evaluate the entropy change using purely thermodynamic methods. It is necessary to then take another route from the initial state to the final state, such that all the intermediary states are in thermal equilibrium. This means that such a route can only be realized in the limit where the changes happen infinitely slowly. Such routes are also referred to as quasistatic routes. In some books one demands that a quasistatic route has to be reversible, here we don't add this extra condition. The net entropy change from the initial state to the final state is independent of the particular choice of the quasistatic route, as the entropy is a function of state.

Suppose that instead of letting the gas undergo a free expansion in which the volume is doubled, a free expansion in allowed which the volume expands by a very small amount dV. After thermal equilibrium is reached, we then let the gas undergo another free expansion by dV and wait until thermal equilibrium is reached. We repeat this until the volume has been doubled. In the limit dV to zero, this becomes an ideal quasistatic process, albeit an irreversible one. Now, according to the fundamental thermodynamic relation, we have:


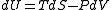
As this equation relates changes in thermodynamic state variables, it is valid for any quasistatic change, regardless of whether it is irreversible or reversible. For the above defined path we have that dU = 0 and thus 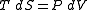, and hence the change in entropy for the Joule expansion is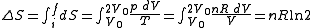


Another path that can be chosen is to let the system undergo a reversible adiabatic expansion in which the volume is doubled. The system will then perform work, so we'll have to supply energy to the system equal to the work performed to make sure it ends up in the same final state as in case of Joule expansion. During the reversible adiabatic expansion, we have 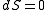, hence
. For an ideal gas we also have 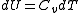. The temperature after the doubling of the volume is thus given as: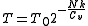


Heating the gas up to the initial temperature of 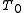quasistatically will increase the entropy by: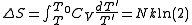

Universal changes

We have calculated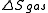 above; we can proceed to infer 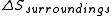and 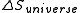as follows: the system is thermally isolated, so the surroundings cannot exchange heat with the gas, and therefore 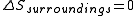. Hence .

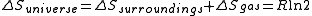We might ask what the entropy would be if, once the Joule expansion has occurred, the gas is put back into the left-hand side by compressing it. The best method (i.e. the method involving the least work) is that of a reversible isothermal compression, which would take work (for one mole) given by