Loop group
Encyclopedia
In mathematics
, a loop group is a group
of loops
in a topological group
G with multiplication defined pointwise. Specifically, let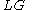
denote the space
of continuous maps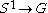
equipped with the compact-open topology
. An element of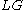
is called a loop in G. Pointwise multiplication of such loops gives 
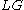the structure of a topological group. The space 
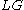is called the free loop group on 
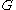. A loop group is any subgroup
of the free loop group
.
An important example of a loop group is the group
of based loops on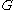
. It is defined to be the kernel of the evaluation map
,
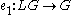and hence is a closed normal subgroup of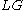
. (Here, 
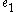is the map that sends a loop to its value at 
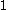.) Note that we may embed 
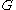into 
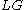as the subgroup of constant loops. Consequently, we arrive at a split exact sequence
.
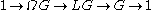The space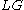
splits as a semi-direct product,
.
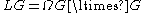We may also think of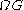
as the loop space on 
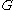. From this point of view, 
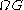is an H-space
with respect to concatenation of loops. On the face of it, this seems to provide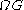
with two very different product maps. However, it can be shown that concatenation and pointwise multiplication are homotopic
. Thus, in terms of the homotopy theory of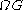
, these maps are interchangeable.
Loop groups were used to explain the phenomenon of Bäcklund transform
s in soliton
equations by Chuu-Lian Terng
and Karen Uhlenbeck
.
Mathematics
Mathematics is the study of quantity, space, structure, and change. Mathematicians seek out patterns and formulate new conjectures. Mathematicians resolve the truth or falsity of conjectures by mathematical proofs, which are arguments sufficient to convince other mathematicians of their validity...
, a loop group is a group
Group (mathematics)
In mathematics, a group is an algebraic structure consisting of a set together with an operation that combines any two of its elements to form a third element. To qualify as a group, the set and the operation must satisfy a few conditions called group axioms, namely closure, associativity, identity...
of loops
Loop (topology)
In mathematics, a loop in a topological space X is a path f from the unit interval I = [0,1] to X such that f = f...
in a topological group
Topological group
In mathematics, a topological group is a group G together with a topology on G such that the group's binary operation and the group's inverse function are continuous functions with respect to the topology. A topological group is a mathematical object with both an algebraic structure and a...
G with multiplication defined pointwise. Specifically, let
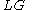
denote the space
Topological space
Topological spaces are mathematical structures that allow the formal definition of concepts such as convergence, connectedness, and continuity. They appear in virtually every branch of modern mathematics and are a central unifying notion...
of continuous maps
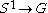
equipped with the compact-open topology
Compact-open topology
In mathematics, the compact-open topology is a topology defined on the set of continuous maps between two topological spaces. The compact-open topology is one of the commonly-used topologies on function spaces, and is applied in homotopy theory and functional analysis...
. An element of
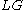is called a loop in G. Pointwise multiplication of such loops gives 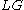the structure of a topological group. The space 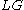is called the free loop group on 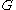. A loop group is any subgroupSubgroup
In group theory, given a group G under a binary operation *, a subset H of G is called a subgroup of G if H also forms a group under the operation *. More precisely, H is a subgroup of G if the restriction of * to H x H is a group operation on H...
of the free loop group
.An important example of a loop group is the group
of based loops on
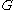. It is defined to be the kernel of the evaluation map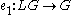,and hence is a closed normal subgroup of
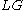. (Here, 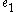is the map that sends a loop to its value at 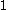.) Note that we may embed 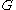into 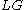as the subgroup of constant loops. Consequently, we arrive at a split exact sequence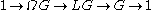.The space
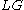splits as a semi-direct product,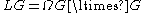.We may also think of
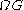as the loop space on 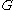. From this point of view, 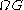is an H-spaceH-space
In mathematics, an H-space is a topological space X together with a continuous map μ : X × X → X with an identity element e so that μ = μ = x for all x in X...
with respect to concatenation of loops. On the face of it, this seems to provide
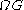with two very different product maps. However, it can be shown that concatenation and pointwise multiplication are homotopicHomotopy
In topology, two continuous functions from one topological space to another are called homotopic if one can be "continuously deformed" into the other, such a deformation being called a homotopy between the two functions...
. Thus, in terms of the homotopy theory of
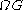, these maps are interchangeable.Loop groups were used to explain the phenomenon of Bäcklund transform
Bäcklund transform
In mathematics, Bäcklund transforms or Bäcklund transformations relate partial differential equations and their solutions. They are an important tool in soliton theory and integrable systems...
s in soliton
Soliton
In mathematics and physics, a soliton is a self-reinforcing solitary wave that maintains its shape while it travels at constant speed. Solitons are caused by a cancellation of nonlinear and dispersive effects in the medium...
equations by Chuu-Lian Terng
Chuu-Lian Terng
Chuu-Lian Terng is a mathematician. She received her B.S. from National Taiwan University in 1971 and her Ph.D. from Brandeis University in 1976 under the supervision of Richard Palais, whom she later married. She is currently a professor at University of California at Irvine.She was a professor...
and Karen Uhlenbeck
Karen Uhlenbeck
Karen Keskulla Uhlenbeck is a professor and Sid W. Richardson Regents Chairholder in the Department of Mathematics at The University of Texas in Austin. In 1998 she was selected to be a Noether Lecturer. In 2000, she became a recipient of the National Medal of Science...
.