
Luminosity distance
Encyclopedia
Luminosity distance DL is defined in terms of the relationship between the absolute magnitude
M and apparent magnitude
m of an astronomical object.
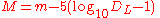
which gives:
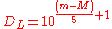
where DL is measured in parsec
s. For nearby objects (say, in the Milky Way
) the luminosity distance gives a good approximation to the natural notion of distance in Euclidean space
.
The relation is less clear for distant objects like quasar
s far beyond the Milky Way
since the apparent magnitude is affected by spacetime
curvature
, redshift
, and time dilation
. Calculating the relation between the apparent and actual luminosity of an object requires taking all of these factors into account. The object's actual luminosity is determined using the inverse-square law and the proportions of the object's apparent distance and luminosity distance.
Another way to express the luminosity distance is through the flux-luminosity relationship. Since,

where F is flux (W·cm−2), and L is luminosity (W), or where F is flux (erg·s−1·cm−2), and L is luminosity (erg·s−1). From this the luminosity distance can be expressed as:
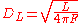
The luminosity distance is related to the "comoving transverse distance" by the equation:
by the equation:

where z is the redshift
.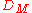 is a factor that allows you to calculate the comoving distance
is a factor that allows you to calculate the comoving distance
between two objects with the same redshift but at different positions of the sky; if the two objects are separated by an angle , the comoving distance between them would be
, the comoving distance between them would be 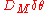 . In a spatially flat universe, the comoving transverse distance
. In a spatially flat universe, the comoving transverse distance  is exactly equal to the radial comoving distance
is exactly equal to the radial comoving distance  , i.e. the comoving distance from ourselves to the object.
, i.e. the comoving distance from ourselves to the object.
Absolute magnitude
Absolute magnitude is the measure of a celestial object's intrinsic brightness. it is also the apparent magnitude a star would have if it were 32.6 light years away from Earth...
M and apparent magnitude
Apparent magnitude
The apparent magnitude of a celestial body is a measure of its brightness as seen by an observer on Earth, adjusted to the value it would have in the absence of the atmosphere...
m of an astronomical object.
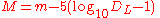
which gives:
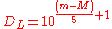
where DL is measured in parsec
Parsec
The parsec is a unit of length used in astronomy. It is about 3.26 light-years, or just under 31 trillion kilometres ....
s. For nearby objects (say, in the Milky Way
Milky Way
The Milky Way is the galaxy that contains the Solar System. This name derives from its appearance as a dim un-resolved "milky" glowing band arching across the night sky...
) the luminosity distance gives a good approximation to the natural notion of distance in Euclidean space
Euclidean space
In mathematics, Euclidean space is the Euclidean plane and three-dimensional space of Euclidean geometry, as well as the generalizations of these notions to higher dimensions...
.
The relation is less clear for distant objects like quasar
Quasar
A quasi-stellar radio source is a very energetic and distant active galactic nucleus. Quasars are extremely luminous and were first identified as being high redshift sources of electromagnetic energy, including radio waves and visible light, that were point-like, similar to stars, rather than...
s far beyond the Milky Way
Milky Way
The Milky Way is the galaxy that contains the Solar System. This name derives from its appearance as a dim un-resolved "milky" glowing band arching across the night sky...
since the apparent magnitude is affected by spacetime
Spacetime
In physics, spacetime is any mathematical model that combines space and time into a single continuum. Spacetime is usually interpreted with space as being three-dimensional and time playing the role of a fourth dimension that is of a different sort from the spatial dimensions...
curvature
Curvature
In mathematics, curvature refers to any of a number of loosely related concepts in different areas of geometry. Intuitively, curvature is the amount by which a geometric object deviates from being flat, or straight in the case of a line, but this is defined in different ways depending on the context...
, redshift
Redshift
In physics , redshift happens when light seen coming from an object is proportionally increased in wavelength, or shifted to the red end of the spectrum...
, and time dilation
Time dilation
In the theory of relativity, time dilation is an observed difference of elapsed time between two events as measured by observers either moving relative to each other or differently situated from gravitational masses. An accurate clock at rest with respect to one observer may be measured to tick at...
. Calculating the relation between the apparent and actual luminosity of an object requires taking all of these factors into account. The object's actual luminosity is determined using the inverse-square law and the proportions of the object's apparent distance and luminosity distance.
Another way to express the luminosity distance is through the flux-luminosity relationship. Since,

where F is flux (W·cm−2), and L is luminosity (W), or where F is flux (erg·s−1·cm−2), and L is luminosity (erg·s−1). From this the luminosity distance can be expressed as:
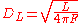
The luminosity distance is related to the "comoving transverse distance"
 by the equation:
by the equation:
where z is the redshift
Redshift
In physics , redshift happens when light seen coming from an object is proportionally increased in wavelength, or shifted to the red end of the spectrum...
.
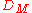 is a factor that allows you to calculate the comoving distance
is a factor that allows you to calculate the comoving distanceComoving distance
In standard cosmology, comoving distance and proper distance are two closely related distance measures used by cosmologists to define distances between objects...
between two objects with the same redshift but at different positions of the sky; if the two objects are separated by an angle
 , the comoving distance between them would be
, the comoving distance between them would be  . In a spatially flat universe, the comoving transverse distance
. In a spatially flat universe, the comoving transverse distance  is exactly equal to the radial comoving distance
is exactly equal to the radial comoving distance  , i.e. the comoving distance from ourselves to the object.
, i.e. the comoving distance from ourselves to the object.
