Nonsqueezing theorem
Encyclopedia
The nonsqueezing theorem is one of the most important theorems in symplectic geometry. It was first proven by Mikhail Gromov in 1985. The theorem states that one cannot embed a sphere into a cylinder via a symplectic map unless the radius of the sphere is less than or equal to the radius of the cylinder. The importance of this theorem is as follows: very little was known about the geometry behind symplectic transformations. One easy consequence of a transformation being symplectic is that it preserves volume. Since one can easily embed a ball of any radius into a cylinder of any other radius by a volume-preserving transformation: just picture squeezing the ball into the cylinder (hence, the name nonsqueezing theorem). Thus, the nonsqueezing theorem tells us that, although symplectic transformations are volume preserving, it is much more restrictive for a transformation to be symplectic than it is to be volume preserving.
the ball of radius R:
and the cylinder of radius r: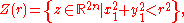
each endowed with the symplectic form
The nonsqueezing theorem tells us that if we can find a symplectic embedding φ : B(R) → Z(r) then R ≤ r.
Background and statement
We start by considering the symplectic spacesthe ball of radius R:

and the cylinder of radius r:
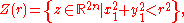
each endowed with the symplectic form
The nonsqueezing theorem tells us that if we can find a symplectic embedding φ : B(R) → Z(r) then R ≤ r.