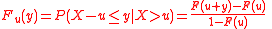Pickands–Balkema–de Haan theorem
Encyclopedia
The Pickands–Balkema–de Haan theorem is often called the second theorem in extreme value theory
. It gives the asymptotic tail distribution of a random variable
X, when the true distribution F of X is unknown. Unlike the first theorem (the Fisher–Tippett–Gnedenko theorem) in extreme value theory, the interest here is the values above a threshold.
 of a random variable
of a random variable  , we are interested in estimating the distribution function
, we are interested in estimating the distribution function  of variable
of variable  above a certain threshold
above a certain threshold  . The distribution function Fu is so called the conditional excess distribution function and is defined as
. The distribution function Fu is so called the conditional excess distribution function and is defined as
for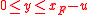 , where
, where  is either the finite or infinite right endpoint of the underlying distribution
is either the finite or infinite right endpoint of the underlying distribution  . The function
. The function  describes the distribution of the excess value over the threshold
describes the distribution of the excess value over the threshold  , given that
, given that  is exceeded.
is exceeded.
 be a sequence of independent and identically-distributed random variables, let
be a sequence of independent and identically-distributed random variables, let 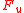 be the conditional excess distribution function. Pickands (1975), Balkema and de Haan (1974) posed that for a large class of underlying distribution function F, for u large, Fu is well approximated by the generalized Pareto distribution. That is:
be the conditional excess distribution function. Pickands (1975), Balkema and de Haan (1974) posed that for a large class of underlying distribution function F, for u large, Fu is well approximated by the generalized Pareto distribution. That is:
where
Here σ > 0, and y ≥ 0 when k ≥ 0 and 0 ≤ y ≤ −σ/k when k < 0.
Extreme value theory
Extreme value theory is a branch of statistics dealing with the extreme deviations from the median of probability distributions. The general theory sets out to assess the type of probability distributions generated by processes...
. It gives the asymptotic tail distribution of a random variable
Random variable
In probability and statistics, a random variable or stochastic variable is, roughly speaking, a variable whose value results from a measurement on some type of random process. Formally, it is a function from a probability space, typically to the real numbers, which is measurable functionmeasurable...
X, when the true distribution F of X is unknown. Unlike the first theorem (the Fisher–Tippett–Gnedenko theorem) in extreme value theory, the interest here is the values above a threshold.
Conditional excess distribution function
If we consider an unknown distribution function of a random variable
of a random variable  , we are interested in estimating the distribution function
, we are interested in estimating the distribution function 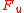 of variable
of variable  above a certain threshold
above a certain threshold  . The distribution function Fu is so called the conditional excess distribution function and is defined as
. The distribution function Fu is so called the conditional excess distribution function and is defined asfor
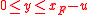 , where
, where 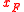 is either the finite or infinite right endpoint of the underlying distribution
is either the finite or infinite right endpoint of the underlying distribution  . The function
. The function  describes the distribution of the excess value over the threshold
describes the distribution of the excess value over the threshold  , given that
, given that  is exceeded.
is exceeded.Statement
Let be a sequence of independent and identically-distributed random variables, let
be a sequence of independent and identically-distributed random variables, let 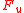 be the conditional excess distribution function. Pickands (1975), Balkema and de Haan (1974) posed that for a large class of underlying distribution function F, for u large, Fu is well approximated by the generalized Pareto distribution. That is:
be the conditional excess distribution function. Pickands (1975), Balkema and de Haan (1974) posed that for a large class of underlying distribution function F, for u large, Fu is well approximated by the generalized Pareto distribution. That is:where
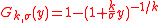 , if
, if 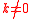
 , if
, if 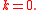
Here σ > 0, and y ≥ 0 when k ≥ 0 and 0 ≤ y ≤ −σ/k when k < 0.
Special cases of generalized Pareto distribution
- Exponential distributionExponential distributionIn probability theory and statistics, the exponential distribution is a family of continuous probability distributions. It describes the time between events in a Poisson process, i.e...
with meanExpected valueIn probability theory, the expected value of a random variable is the weighted average of all possible values that this random variable can take on...
 , if k = 0.
, if k = 0. - Triangular distribution, if k = 1/2.
- Uniform distribution on
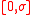 , if k = 1.
, if k = 1. - Pareto distribution, if k < 0.