Quantum tic-tac-toe is a "quantum

Quantum mechanics

Quantum mechanics, also known as quantum physics or quantum theory, is a branch of physics providing a mathematical description of much of the dual particle-like and wave-like behavior and interactions of energy and matter. It departs from classical mechanics primarily at the atomic and subatomic...

 generalization" of tic-tac-toe

Tic-tac-toe

Tic-tac-toe, also called wick wack woe and noughts and crosses , is a pencil-and-paper game for two players, X and O, who take turns marking the spaces in a 3×3 grid. The X player usually goes first...

 in which the players' moves are "superpositions" of plays in the classical game. The game was invented by Allan Goff of Novatia Labs.

Quote

Quantum tic tac toe offers a way of introducing quantum physics without advanced mathematics, provides a conceptual foundation for understanding the meaning of quantum mechanics, and is fun to play. —Allan Goff

The motivation

The rules of quantum tic-tac-toe are not particularly hard, but their unfamiliarity initially makes learning them somewhat more challenging than for conventional games. It may help, therefore, to consider why this game was invented.

The motivation to invent quantum tic-tac-toe was to explore what it means to be in two places at once. In classical physics, a single object cannot be in two places at once. In quantum physics, however, the mathematics used to describe quantum systems seems to imply that, when not being observed, quantum particles can be in multiple places at once. (The textbook example of this is the double-slit experiment

Double-slit experiment

The double-slit experiment, sometimes called Young's experiment, is a demonstration that matter and energy can display characteristics of both waves and particles...

.) How the universe can be like this is a bit mysterious. There is a disconnect between the mathematics and our mental images of reality, a disconnect that is absent in classical physics. This is why quantum mechanics supports multiple "interpretations." An interpretation is a formal effort to explain what a theory means, to articulate a model

Mathematical model

A mathematical model is a description of a system using mathematical concepts and language. The process of developing a mathematical model is termed mathematical modeling. Mathematical models are used not only in the natural sciences and engineering disciplines A mathematical model is a...

. By definition, interpretations are not testable. Testability implies a theory or at least a hypothesis. The success of quantum mechanics is not in dispute, and it has been validated over an incredibly wide range of phenomena and to astonishingly high levels of precision. Yet the problem of multiple mutually incompatible interpretations remains a bit of a professional embarrassment.

The researchers who invented quantum tic-tac-toe were studying abstract quantum systems, formal systems whose axiomatic foundation included only a few of the axioms of quantum mechanics. Quantum tic-tac-toe became the most thoroughly studied abstract quantum system and offered insights that spawned new research. It also turned out to be a fun and engaging game, a game which also provides good pedagogy in the classroom.

The rules of quantum tic-tac-toe attempt to capture several phenomena of quantum systems. These phenomena are superposition, entanglement

Quantum entanglement

Quantum entanglement occurs when electrons, molecules even as large as "buckyballs", photons, etc., interact physically and then become separated; the type of interaction is such that each resulting member of a pair is properly described by the same quantum mechanical description , which is...

 and collapse. Superposition is the ability of quantum objects to be in two places at once. Entanglement is the phenomenon where distant parts of a quantum system display correlations that cannot be explained by either timelike causality or common cause. Collapse is the phenomenon where the quantum states of a system are reduced to classical states. Collapses occur when a measurement happens, but the mathematics of the current formulation of quantum mechanics is silent on the measurement process. Many of the interpretations of quantum mechanics derive from different efforts to deal with the measurement problem.

The rules

Quantum tic-tac-toe captures the three quantum phenomena discussed above by modifying one basic rule of classical tic-tac-toe: the number of marks allowed in each square. Additional rules specify when and how a set of marks "collapses" into classical moves.

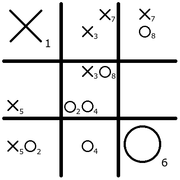
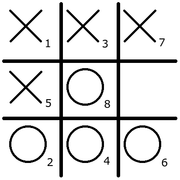
On each move, the current player marks two squares with their letter (X or O), instead of one, and each letter (X or O) is subscripted with the number of the move (beginning counting with 1). The pair of marks are called spooky marks. (Because X always moves first, the subscripts on X are always odd and the subscripts on O are always even.)

For example, player 1's first move might be to place "X₁" in both the upper left and lower right squares. The two squares thus marked are called entangled. During the game, there may be as many as eight spooky marks in a single square (if the square is entangled with all eight other squares).

The phenomenon of collapse is captured by specifying that a "cyclic entanglement" causes a "measurement". A cyclic entanglement is a cycle

Cycle (graph theory)

In graph theory, the term cycle may refer to a closed path. If repeated vertices are allowed, it is more often called a closed walk. If the path is a simple path, with no repeated vertices or edges other than the starting and ending vertices, it may also be called a simple cycle, circuit, circle,...

in the entanglement graph; for example, if square 1 is entangled via move X₁ with square 4, which is entangled via move X₃ with square 8, which is in turn entangled via move O₄ with square 1, then these three squares form a cyclic entanglement. At the end of the turn on which the cyclic entanglement was created, the player whose turn it is not — that is, the player who did not create the cycle — chooses one of two ways to "measure" the cycle and thus cause all the entangled squares to "collapse" into classical tic-tac-toe moves. In the preceding example, since player 2 created the cycle, player 1 decides how to "measure" it. Player 1's two options are:

1. X₁ collapses into square 1. This forces O₄ to collapse into square 8 and X₃ to collapse into square 4.
2. X₁ collapses into square 4. This forces X₃ to collapse into square 8 and O₄ to collapse into square 1.

Any other chains of entanglements hanging off the cycle would also collapse at this time; for example, if square 1 were also entangled via O₂ with square 5, then either measurement above would force O₂ to collapse into square 5. (Note that it is impossible for two or more cyclic entanglements to be created in a single turn.)

When a move collapses into a single square, that square is permanently marked (in larger print) with the letter and subscript of the collapsed move — a classical mark. A square containing a classical mark is fixed for the rest of the game; no more spooky marks may be placed in it.

The first player to achieve a tic-tac-toe (three in a row horizontally, vertically, or diagonally) consisting entirely of classical marks is declared the winner. Since it is possible for a single measurement to collapse the entire board and give classical tic-tac-toes to both players simultaneously, the rules declare that the player whose tic-tac-toe has the lower maximum subscript earns one point, and the player whose tic-tac-toe has the higher maximum subscript earns only one-half point.

External links

• Play quantum tic-tac-toe against a bot (HTML5)
• Play quantum tic-tac-toe (Java applet)
• Quantum tic-tac-toe iPhone App