Quasi-Lie algebra
Encyclopedia
In mathematics
, a quasi-Lie algebra in abstract algebra
is just like a Lie algebra
, but with the usual axiom
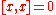
replaced by
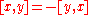(anti-symmetry).
In characteristic
other than 2, these are equivalent (in the presence of bilinearity
), so this distinction doesn't arise when considering real or complex Lie algebras. It can however become important, when considering Lie algebras over the integers.
In a quasi-Lie algebra,
Therefore the bracket of any element with itself is 2-torsion, if it does not actually vanish.
Mathematics
Mathematics is the study of quantity, space, structure, and change. Mathematicians seek out patterns and formulate new conjectures. Mathematicians resolve the truth or falsity of conjectures by mathematical proofs, which are arguments sufficient to convince other mathematicians of their validity...
, a quasi-Lie algebra in abstract algebra
Abstract algebra
Abstract algebra is the subject area of mathematics that studies algebraic structures, such as groups, rings, fields, modules, vector spaces, and algebras...
is just like a Lie algebra
Lie algebra
In mathematics, a Lie algebra is an algebraic structure whose main use is in studying geometric objects such as Lie groups and differentiable manifolds. Lie algebras were introduced to study the concept of infinitesimal transformations. The term "Lie algebra" was introduced by Hermann Weyl in the...
, but with the usual axiom
Axiom
In traditional logic, an axiom or postulate is a proposition that is not proven or demonstrated but considered either to be self-evident or to define and delimit the realm of analysis. In other words, an axiom is a logical statement that is assumed to be true...
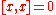
replaced by
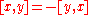(anti-symmetry).In characteristic
Characteristic (algebra)
In mathematics, the characteristic of a ring R, often denoted char, is defined to be the smallest number of times one must use the ring's multiplicative identity element in a sum to get the additive identity element ; the ring is said to have characteristic zero if this repeated sum never reaches...
other than 2, these are equivalent (in the presence of bilinearity
Bilinear operator
In mathematics, a bilinear operator is a function combining elements of two vector spaces to yield an element of a third vector space that is linear in each of its arguments. Matrix multiplication is an example.-Definition:...
), so this distinction doesn't arise when considering real or complex Lie algebras. It can however become important, when considering Lie algebras over the integers.
In a quasi-Lie algebra,
Therefore the bracket of any element with itself is 2-torsion, if it does not actually vanish.