Quasi-triangular Quasi-Hopf algebra
Encyclopedia
A quasi-triangular quasi-Hopf algebra is a specialized form of a quasi-Hopf algebra defined by the Ukrainian
mathematician Vladimir Drinfeld in 1989. It is also a generalized form of a quasi-triangular Hopf algebra.
A quasi-triangular quasi-Hopf algebra is a set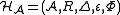
where 
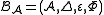is a quasi-Hopf algebra and 
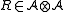known as the R-matrix, is an invertible element such that
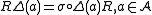
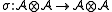
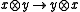
so that
is the switch map and
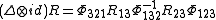
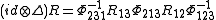
where
and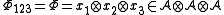 
.
The quasi-Hopf algebra becomes triangular if in addition,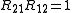
.
The twisting of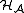
by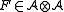 
is the same as for a quasi-Hopf algebra, with the additional definition of the twisted R-matrix
A quasi-triangular (resp. triangular) quasi-Hopf algebra with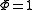
is a quasi-triangular (resp. triangular) Hopf algebra as the latter two conditions in the definition reduce the conditions of quasi-triangularity of a Hopf algebra .
Similarly to the twisting properties of the quasi-Hopf algebra, the property of being quasi-triangular or triangular quasi-Hopf algebra is preserved by twisting.
Ukraine
Ukraine is a country in Eastern Europe. It has an area of 603,628 km², making it the second largest contiguous country on the European continent, after Russia...
mathematician Vladimir Drinfeld in 1989. It is also a generalized form of a quasi-triangular Hopf algebra.
A quasi-triangular quasi-Hopf algebra is a set
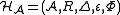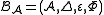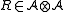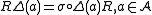
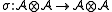
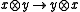
where is a quasi-Hopf algebra and known as the R-matrix, is an invertible element such thatso that
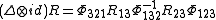
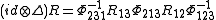
is the switch map andwhere
and 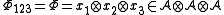.The quasi-Hopf algebra becomes triangular if in addition,
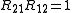.The twisting of
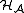by 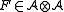is the same as for a quasi-Hopf algebra, with the additional definition of the twisted R-matrixA quasi-triangular (resp. triangular) quasi-Hopf algebra with
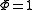is a quasi-triangular (resp. triangular) Hopf algebra as the latter two conditions in the definition reduce the conditions of quasi-triangularity of a Hopf algebra .Similarly to the twisting properties of the quasi-Hopf algebra, the property of being quasi-triangular or triangular quasi-Hopf algebra is preserved by twisting.