Rational sequence topology
Encyclopedia
In mathematics
, more specifically general topology
, the rational sequence topology is an example of a topology given to the set of real number
s, denoted R.
To give R a topology means to say which subset
s of R are "open", and to do so in a way that the following axiom
s are met:
(cf. rational number
). Take a sequence
of rational numbers {xk} with the property that {xk} converge
s, with respect to the Euclidean topology
, towards x as k tends towards infinity. Informally, this means that each of the numbers in the sequence get closer and closer to x as we progress further and further along the sequence.
The rational sequence topology is given by defining both the whole set R and the empty set ∅ to be open, defining each rational number singleton to be open, and using as a basis for the irrational number x, the sets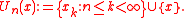
Mathematics
Mathematics is the study of quantity, space, structure, and change. Mathematicians seek out patterns and formulate new conjectures. Mathematicians resolve the truth or falsity of conjectures by mathematical proofs, which are arguments sufficient to convince other mathematicians of their validity...
, more specifically general topology
General topology
In mathematics, general topology or point-set topology is the branch of topology which studies properties of topological spaces and structures defined on them...
, the rational sequence topology is an example of a topology given to the set of real number
Real number
In mathematics, a real number is a value that represents a quantity along a continuum, such as -5 , 4/3 , 8.6 , √2 and π...
s, denoted R.
To give R a topology means to say which subset
Subset
In mathematics, especially in set theory, a set A is a subset of a set B if A is "contained" inside B. A and B may coincide. The relationship of one set being a subset of another is called inclusion or sometimes containment...
s of R are "open", and to do so in a way that the following axiom
Axiom
In traditional logic, an axiom or postulate is a proposition that is not proven or demonstrated but considered either to be self-evident or to define and delimit the realm of analysis. In other words, an axiom is a logical statement that is assumed to be true...
s are met:
- The union of open sets is an open set.
- The finite intersection of open sets is an open set.
- R and the empty setEmpty setIn mathematics, and more specifically set theory, the empty set is the unique set having no elements; its size or cardinality is zero. Some axiomatic set theories assure that the empty set exists by including an axiom of empty set; in other theories, its existence can be deduced...
∅ are open sets.
Construction
Let x be an irrational numberIrrational number
In mathematics, an irrational number is any real number that cannot be expressed as a ratio a/b, where a and b are integers, with b non-zero, and is therefore not a rational number....
(cf. rational number
Rational number
In mathematics, a rational number is any number that can be expressed as the quotient or fraction a/b of two integers, with the denominator b not equal to zero. Since b may be equal to 1, every integer is a rational number...
). Take a sequence
Sequence
In mathematics, a sequence is an ordered list of objects . Like a set, it contains members , and the number of terms is called the length of the sequence. Unlike a set, order matters, and exactly the same elements can appear multiple times at different positions in the sequence...
of rational numbers {xk} with the property that {xk} converge
Limit of a sequence
The limit of a sequence is, intuitively, the unique number or point L such that the terms of the sequence become arbitrarily close to L for "large" values of n...
s, with respect to the Euclidean topology
Euclidean topology
In mathematics, and especially general topology, the Euclidean topology is an example of a topology given to the set of real numbers, denoted by R...
, towards x as k tends towards infinity. Informally, this means that each of the numbers in the sequence get closer and closer to x as we progress further and further along the sequence.
The rational sequence topology is given by defining both the whole set R and the empty set ∅ to be open, defining each rational number singleton to be open, and using as a basis for the irrational number x, the sets