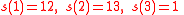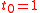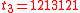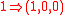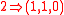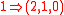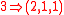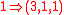Rauzy fractal
Encyclopedia
In mathematics, the Rauzy fractal is a fractal
set associated to the Tribonacci substitution
It has been studied in 1981 by Gérard Rauzy, with the idea of generalizing the dynamic properties of the Fibonacci morphism
.
That fractal set can be generalized to other maps on a 3 letter alphabet, generating other fractal sets with interesting properties (periodic tiling of the plane, self-similarity
in three homothetic parts..)
 ,
,  ,
, 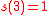 .
.
Starting from 1, the Tribonacci words are :
We can show that, for ,
,  , hence the name Tribonacci".
, hence the name Tribonacci".
Let's consider, now, the space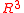 with cartesian coordinates (x,y,z).
with cartesian coordinates (x,y,z).
The Rauzy fractal is constructed this way:
1) Interpret the sequence of letters of the infinite Tribonacci word as a sequence of unitary vectors
of the space, with the following rules (1 = direction x, 2 = direction y, 3 = direction z).
2) Then, build a "stair" by tracing the points reached by this sequence of vectors (see figure). For example, the first points are:
etc...Every point can be coloured according to the corresponding letter, to stress the self-similarity property.
3) Then, project those points on the contracting plane (plane orthogonal to the main direction of propagation of the points, none of those projected points escape to infinity).
and generate, for the examples below, a periodic tiling of the plane.
Fractal
A fractal has been defined as "a rough or fragmented geometric shape that can be split into parts, each of which is a reduced-size copy of the whole," a property called self-similarity...
set associated to the Tribonacci substitution
It has been studied in 1981 by Gérard Rauzy, with the idea of generalizing the dynamic properties of the Fibonacci morphism
Fibonacci word
thumb|350px|Characterization by a [[cutting sequence]] with a line of slope 1/\varphi or \varphi-1, with \varphi the [[golden ratio]].A Fibonacci word is a specific sequence of binary digits...
.
That fractal set can be generalized to other maps on a 3 letter alphabet, generating other fractal sets with interesting properties (periodic tiling of the plane, self-similarity
Self-similarity
In mathematics, a self-similar object is exactly or approximately similar to a part of itself . Many objects in the real world, such as coastlines, are statistically self-similar: parts of them show the same statistical properties at many scales...
in three homothetic parts..)
Definitions
The infinite tribonacci word is a word constructed by applying iteratively the Tribonacci map : ,
,  ,
, 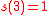 .
.Starting from 1, the Tribonacci words are :
We can show that, for
 ,
,  , hence the name Tribonacci".
, hence the name Tribonacci".Let's consider, now, the space
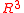 with cartesian coordinates (x,y,z).
with cartesian coordinates (x,y,z).The Rauzy fractal is constructed this way:
1) Interpret the sequence of letters of the infinite Tribonacci word as a sequence of unitary vectors
Vector (mathematics and physics)
In mathematics and physics, a vector is an element of a vector space. If n is a non negative integer and K is either the field of the real numbers or the field of the complex number, then K^n is naturally endowed with a structure of vector space, where K^n is the set of the ordered sequences of n...
of the space, with the following rules (1 = direction x, 2 = direction y, 3 = direction z).
2) Then, build a "stair" by tracing the points reached by this sequence of vectors (see figure). For example, the first points are:
etc...Every point can be coloured according to the corresponding letter, to stress the self-similarity property.
3) Then, project those points on the contracting plane (plane orthogonal to the main direction of propagation of the points, none of those projected points escape to infinity).
Properties
- Can be tiledTessellationA tessellation or tiling of the plane is a pattern of plane figures that fills the plane with no overlaps and no gaps. One may also speak of tessellations of parts of the plane or of other surfaces. Generalizations to higher dimensions are also possible. Tessellations frequently appeared in the art...
by three copies of itself, reduced by factors ,
,  et
et 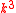 with
with  solution of
solution of  :
:  .
. - Stable by exchanging pieces. We can obtain the same set by exchanging the place of the pieces.
- ConnectedConnected spaceIn topology and related branches of mathematics, a connected space is a topological space that cannot be represented as the union of two or more disjoint nonempty open subsets. Connectedness is one of the principal topological properties that is used to distinguish topological spaces...
and simply connected. Has no hole. - Tiles the plane periodically, by translation.
- The matrix of the Tribonacci map has for characteristic polynomialCharacteristic polynomialIn linear algebra, one associates a polynomial to every square matrix: its characteristic polynomial. This polynomial encodes several important properties of the matrix, most notably its eigenvalues, its determinant and its trace....
 , its eigenvalues are a real number
, its eigenvalues are a real number 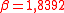 , called Tribonacci constant, a Pisot number, and two complex conjugated numbers
, called Tribonacci constant, a Pisot number, and two complex conjugated numbers  and
and  with
with  .
. - Its boundary is fractal, and the Hausdorff dimensionHausdorff dimensionthumb|450px|Estimating the Hausdorff dimension of the coast of Great BritainIn mathematics, the Hausdorff dimension is an extended non-negative real number associated with any metric space. The Hausdorff dimension generalizes the notion of the dimension of a real vector space...
of this boundary equals 1.0933. (Solution of ) .
) .
Variants and generalization
For any unimodular substitution of Pisot type, which verifies a coïncidence condition (apparently always verified), one can construct a similar set called "Rauzy fractal of the map". They all display self-similaritySelf-similarity
In mathematics, a self-similar object is exactly or approximately similar to a part of itself . Many objects in the real world, such as coastlines, are statistically self-similar: parts of them show the same statistical properties at many scales...
and generate, for the examples below, a periodic tiling of the plane.