
Reciprocal rule
Encyclopedia
In calculus
, the reciprocal rule is a shorthand method of finding the derivative
of a function
that is the reciprocal
of a differentiable function, without using the quotient rule or chain rule
.
The reciprocal rule states that the derivative of is given by
is given by
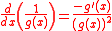
where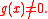
 . Then,
. Then,
, by a process very much like that of the derivation of the quotient rule. One thinks of
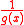 as being the function
as being the function  composed with the function
composed with the function 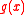 . The result then follows by application of the chain rule.
. The result then follows by application of the chain rule.
 is:
is:
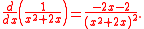
The derivative of (when
(when 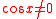 ) is:
) is:
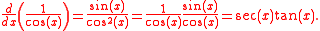
For more general examples, see the derivative
article.
Calculus
Calculus is a branch of mathematics focused on limits, functions, derivatives, integrals, and infinite series. This subject constitutes a major part of modern mathematics education. It has two major branches, differential calculus and integral calculus, which are related by the fundamental theorem...
, the reciprocal rule is a shorthand method of finding the derivative
Derivative
In calculus, a branch of mathematics, the derivative is a measure of how a function changes as its input changes. Loosely speaking, a derivative can be thought of as how much one quantity is changing in response to changes in some other quantity; for example, the derivative of the position of a...
of a function
Function (mathematics)
In mathematics, a function associates one quantity, the argument of the function, also known as the input, with another quantity, the value of the function, also known as the output. A function assigns exactly one output to each input. The argument and the value may be real numbers, but they can...
that is the reciprocal
Multiplicative inverse
In mathematics, a multiplicative inverse or reciprocal for a number x, denoted by 1/x or x−1, is a number which when multiplied by x yields the multiplicative identity, 1. The multiplicative inverse of a fraction a/b is b/a. For the multiplicative inverse of a real number, divide 1 by the...
of a differentiable function, without using the quotient rule or chain rule
Chain rule
In calculus, the chain rule is a formula for computing the derivative of the composition of two or more functions. That is, if f is a function and g is a function, then the chain rule expresses the derivative of the composite function in terms of the derivatives of f and g.In integration, the...
.
The reciprocal rule states that the derivative of
 is given by
is given by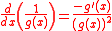
where
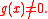
From the quotient rule
The reciprocal rule is derived from the quotient rule, with the numerator . Then,
. Then,
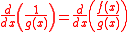 |
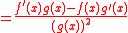 |
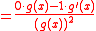 |
|
 |
From the chain rule
It is also possible to derive the reciprocal rule from the chain ruleChain rule
In calculus, the chain rule is a formula for computing the derivative of the composition of two or more functions. That is, if f is a function and g is a function, then the chain rule expresses the derivative of the composite function in terms of the derivatives of f and g.In integration, the...
, by a process very much like that of the derivation of the quotient rule. One thinks of
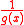 as being the function
as being the function  composed with the function
composed with the function 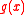 . The result then follows by application of the chain rule.
. The result then follows by application of the chain rule.Examples
The derivative of is:
is: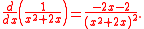
The derivative of
 (when
(when 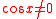 ) is:
) is: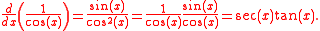
For more general examples, see the derivative
Derivative
In calculus, a branch of mathematics, the derivative is a measure of how a function changes as its input changes. Loosely speaking, a derivative can be thought of as how much one quantity is changing in response to changes in some other quantity; for example, the derivative of the position of a...
article.

