Relative Utilitarianism
Encyclopedia
Relative Utilitarianism
Let
be a set of possible `states of the world' or `alternatives'; society wishes to chose some state from 
. Let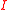 
be a finite set, representing a collection of people. For each 
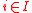, let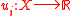 
be a utility function
. A social choice rule
(or voting system
) is a mechanism which uses the data
to select some element(s) from 
which are `best' for society. (The basic problem of social choice theory
is to disambiguate the word `best'.)
The classic utilitarian
social choice rule selects the element
which maximizes the utilitarian sum
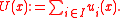
However, for this formula to make sense, we must assume that the utility functions
are both cardinal
, and interpersonally comparable at a cardinal level.
The notion that individuals have cardinal utility functions is not that problematic. Cardinal utility has been implicitly assumed in decision theory
ever since Daniel Bernoulli
's analysis of the Saint Petersburg Paradox. Rigorous mathematical theories of cardinal utility (with application to risky decision making) were developed by Frank P. Ramsey
, Bruno de Finetti
,
von Neumann and Morgenstern
, and Leonard Savage
. However, in these theories, a person's utility function is only well-defined up to an `affine rescaling'. Thus, if the utility function
is valid description of her preferences, and if 
are two constants with 
, then the `rescaled' utility function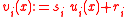 
is an equally valid description of her preferences. If we define a new package of utility functions 
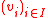using possibly different 
and 
for all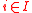 
, and we then consider the utilitarian sum
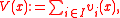
then in general, the maximizer of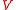
will not be the same as the maximizer of 
. Thus, in a sense, classic utilitarian social choice is not well-defined within the standard model of cardinal utility used in decision theory, unless we specify some mechanism to `calibrate' the utility functions of the different individuals.
Relative utilitarianism proposes a natural calibration mechanism. For every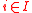
, suppose that the values
are well-defined. (For example, this will always be true if
is finite, or if 
is a compact space and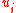 
is a continuous function.) Then define
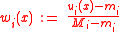
for all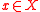
. Thus, 
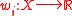is a `rescaled' utility function which has a minimum value of 0 and a maximum value of 1. The Relative Utilitarian social choice rule selects the element in 
which maximizes the utilitarian sum
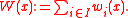
As an abstract social choice function, relative utilitarianism has been analyzed by Cao (1982), Dhillon (1998), Karni (1998), Dhillon and Mertens (1999), Segal (2000), Sobel (2001) and Pivato (2008). (Cao (1982) refers to it as the `modified Thomson solution'.) When interpreted as a `voting rule', it is equivalent to Range voting
.
Let
be a set of possible `states of the world' or `alternatives'; society wishes to chose some state from . Let 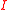be a finite set, representing a collection of people. For each 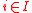, let 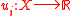be a utility functionUtility
In economics, utility is a measure of customer satisfaction, referring to the total satisfaction received by a consumer from consuming a good or service....
. A social choice rule
Social choice theory
Social choice theory is a theoretical framework for measuring individual interests, values, or welfares as an aggregate towards collective decision. A non-theoretical example of a collective decision is passing a set of laws under a constitution. Social choice theory dates from Condorcet's...
(or voting system
Voting system
A voting system or electoral system is a method by which voters make a choice between options, often in an election or on a policy referendum....
) is a mechanism which uses the data
to select some element(s) from which are `best' for society. (The basic problem of social choice theorySocial choice theory
Social choice theory is a theoretical framework for measuring individual interests, values, or welfares as an aggregate towards collective decision. A non-theoretical example of a collective decision is passing a set of laws under a constitution. Social choice theory dates from Condorcet's...
is to disambiguate the word `best'.)
The classic utilitarian
Utilitarianism
Utilitarianism is an ethical theory holding that the proper course of action is the one that maximizes the overall "happiness", by whatever means necessary. It is thus a form of consequentialism, meaning that the moral worth of an action is determined only by its resulting outcome, and that one can...
social choice rule selects the element
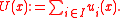
which maximizes the utilitarian sumHowever, for this formula to make sense, we must assume that the utility functions
are both cardinalCardinal utility
In economics, cardinal utility refers to a property of mathematical indices that preserve preference orderings uniquely up to positive linear transformations...
, and interpersonally comparable at a cardinal level.
The notion that individuals have cardinal utility functions is not that problematic. Cardinal utility has been implicitly assumed in decision theory
Decision theory
Decision theory in economics, psychology, philosophy, mathematics, and statistics is concerned with identifying the values, uncertainties and other issues relevant in a given decision, its rationality, and the resulting optimal decision...
ever since Daniel Bernoulli
Daniel Bernoulli
Daniel Bernoulli was a Dutch-Swiss mathematician and was one of the many prominent mathematicians in the Bernoulli family. He is particularly remembered for his applications of mathematics to mechanics, especially fluid mechanics, and for his pioneering work in probability and statistics...
's analysis of the Saint Petersburg Paradox. Rigorous mathematical theories of cardinal utility (with application to risky decision making) were developed by Frank P. Ramsey
Frank P. Ramsey
Frank Plumpton Ramsey was a British mathematician who, in addition to mathematics, made significant and precocious contributions in philosophy and economics before his death at the age of 26...
, Bruno de Finetti
Coherence (philosophical gambling strategy)
In a thought experiment proposed by the Italian probabilist Bruno de Finetti in order to justify Bayesian probability, an array of wagers is coherent precisely if it does not expose the wagerer to certain loss regardless of the outcomes of events on which he is wagering, even if his opponent makes...
,
von Neumann and Morgenstern
Von Neumann–Morgenstern utility theorem
In 1947, John von Neumann and Oskar Morgenstern exhibited four relatively modest axioms of "rationality" such that any agent satisfying the axioms has a utility function...
, and Leonard Savage
Subjective expected utility
Subjective expected utility is a method in decision theory in the presence of risk, promoted by L. J. Savage in 1954 following previous work by Ramsey and von Neumann...
. However, in these theories, a person's utility function is only well-defined up to an `affine rescaling'. Thus, if the utility function
is valid description of her preferences, and if are two constants with , then the `rescaled' utility function 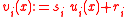is an equally valid description of her preferences. If we define a new package of utility functions 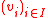using possibly different and for all 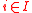, and we then consider the utilitarian sum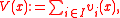then in general, the maximizer of
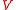will not be the same as the maximizer of . Thus, in a sense, classic utilitarian social choice is not well-defined within the standard model of cardinal utility used in decision theory, unless we specify some mechanism to `calibrate' the utility functions of the different individuals.Relative utilitarianism proposes a natural calibration mechanism. For every
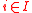, suppose that the valuesare well-defined. (For example, this will always be true if
is finite, or if is a compact space and 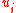is a continuous function.) Then define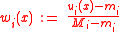for all
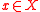. Thus, 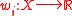is a `rescaled' utility function which has a minimum value of 0 and a maximum value of 1. The Relative Utilitarian social choice rule selects the element in which maximizes the utilitarian sum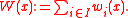As an abstract social choice function, relative utilitarianism has been analyzed by Cao (1982), Dhillon (1998), Karni (1998), Dhillon and Mertens (1999), Segal (2000), Sobel (2001) and Pivato (2008). (Cao (1982) refers to it as the `modified Thomson solution'.) When interpreted as a `voting rule', it is equivalent to Range voting
Range voting
Range voting is a voting system for one-seat elections under which voters score each candidate, the scores are added up, and the candidate with the highest score wins.A form of range voting was apparently used in...
.