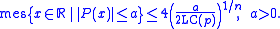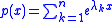Remez inequality
Encyclopedia
In mathematics, the Remez inequality, discovered by the Ukrainian mathematician Evgeny Yakovlevich Remez
, gives a bound on the sup norms of certain polynomials, the bound being attained by the Chebyshev polynomials
.
on some set of measure ≥ 2 contained in the closed interval [−1, 1+σ]. Then the Remez inequality states that
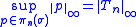
where Tn(x) is the Chebyshev polynomial of degree n, and the supremum norm is taken over the interval [−1, 1+σ].
Observe that Tn is increasing on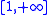
, hence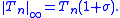
The R.i., combined with an estimate on Chebyshev polynomials, implies the following
corollary: If J ⊂ R is a finite interval, and E ⊂ J is an arbitrary measurable set, then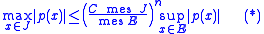
for any polynomial p of degree n. Here C>0 is a numerical constant.
Let
be an exponential sum
(with arbitrary λk ∈C), and let J ⊂ R be a finite interval, E ⊂ J — an arbitrary measurable set. Then
where C>0 is a numerical constant.
In the special case when λk are pure imaginary and integer, and the subset E is itself an interval, the inequality was proved by Pál Turán
and is known as Turán's lemma.
, and states that the Lebesgue measure of a sub-level set of a polynomial p of degree n is bounded in terms of the leading coefficient LC(p) as follows:
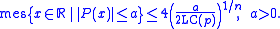
Evgeny Yakovlevich Remez
Evgeny Yakovlevich Remez Evgeny Yakovlevich Remez Evgeny Yakovlevich Remez (sometimes spelled as Evgenii Yakovlevich Remez, ; (born 1896 in Mstislavl, now Belarus; died 1975 in Kiev) was a Soviet mathematician...
, gives a bound on the sup norms of certain polynomials, the bound being attained by the Chebyshev polynomials
Chebyshev polynomials
In mathematics the Chebyshev polynomials, named after Pafnuty Chebyshev, are a sequence of orthogonal polynomials which are related to de Moivre's formula and which can be defined recursively. One usually distinguishes between Chebyshev polynomials of the first kind which are denoted Tn and...
.
The inequality
Let σ be an arbitrary fixed positive number. Define the class of polynomials πn(σ) to be those polynomials p of the nth degree for whichon some set of measure ≥ 2 contained in the closed interval [−1, 1+σ]. Then the Remez inequality states that
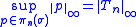
where Tn(x) is the Chebyshev polynomial of degree n, and the supremum norm is taken over the interval [−1, 1+σ].
Observe that Tn is increasing on
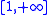, hence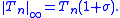The R.i., combined with an estimate on Chebyshev polynomials, implies the following
corollary: If J ⊂ R is a finite interval, and E ⊂ J is an arbitrary measurable set, then
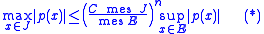
for any polynomial p of degree n. Here C>0 is a numerical constant.
Extensions
Inequalities similar to (*) have been proved for different classes of functions, and are known as Remez-type inequalities. One important example is Nazarov's inequality for exponential sums :Let
be an exponential sum
Exponential sum
In mathematics, an exponential sum may be a finite Fourier series , or other finite sum formed using the exponential function, usually expressed by means of the functione = \exp.\,...
(with arbitrary λk ∈C), and let J ⊂ R be a finite interval, E ⊂ J — an arbitrary measurable set. Then
where C>0 is a numerical constant.
In the special case when λk are pure imaginary and integer, and the subset E is itself an interval, the inequality was proved by Pál Turán
Pál Turán
Paul Turán was a Hungarian mathematician who worked primarily in number theory. He had a long collaboration with fellow Hungarian mathematician Paul Erdős, lasting 46 years and resulting in 28 joint papers.- Life and education :...
and is known as Turán's lemma.
Pólya inequality
One of the corollaries of the R.i. is the Pólya inequality, which was proved by George PólyaGeorge Pólya
George Pólya was a Hungarian mathematician. He was a professor of mathematics from 1914 to 1940 at ETH Zürich and from 1940 to 1953 at Stanford University. He made fundamental contributions to combinatorics, number theory, numerical analysis and probability theory...
, and states that the Lebesgue measure of a sub-level set of a polynomial p of degree n is bounded in terms of the leading coefficient LC(p) as follows: