Mathematics

In mathematics

Mathematics

Mathematics is the study of quantity, space, structure, and change. Mathematicians seek out patterns and formulate new conjectures. Mathematicians resolve the truth or falsity of conjectures by mathematical proofs, which are arguments sufficient to convince other mathematicians of their validity...

, a dynamical system

Dynamical system

A dynamical system is a concept in mathematics where a fixed rule describes the time dependence of a point in a geometrical space. Examples include the mathematical models that describe the swinging of a clock pendulum, the flow of water in a pipe, and the number of fish each springtime in a...

 is invertible if the forward evolution is one-to-one, not many-to-one; so that for every state there exists a well-defined reverse-time evolution operator.

The dynamics are time-reversible if there exists a transformation (an involution) π which gives a one-to-one mapping between the time-reversed evolution of any one state, and the forward-time evolution of another corresponding state, given by the operator equation:


Any time-independent structures (for example critical points

Critical point (mathematics)

In calculus, a critical point of a function of a real variable is any value in the domain where either the function is not differentiable or its derivative is 0. The value of the function at a critical point is a critical value of the function...

, or attractor

Attractor

An attractor is a set towards which a dynamical system evolves over time. That is, points that get close enough to the attractor remain close even if slightly disturbed...

s) which the dynamics gives rise to must therefore either be self-symmetrical or have symmetrical images under the involution π.

Physics

In physics

Physics

Physics is a natural science that involves the study of matter and its motion through spacetime, along with related concepts such as energy and force. More broadly, it is the general analysis of nature, conducted in order to understand how the universe behaves.Physics is one of the oldest academic...

, the laws of motion

Laws of motion

In physics, a number of noted theories of the motion of objects have developed. Among the best-known are:* Classical mechanics** Newton's laws of motion**Euler's laws**Cauchy's equations of motion** Kepler's laws of planetary motion ** General relativity...

 of classical mechanics

Classical mechanics

In physics, classical mechanics is one of the two major sub-fields of mechanics, which is concerned with the set of physical laws describing the motion of bodies under the action of a system of forces...

 have the above property, if the operator π reverses the conjugate momenta of all the particles of the system, p -> -p . (T-symmetry

T-symmetry

T Symmetry is the symmetry of physical laws under a time reversal transformation: T: t \mapsto -t.Although in restricted contexts one may find this symmetry, the observable universe itself does not show symmetry under time reversal, primarily due to the second law of thermodynamics.Time asymmetries...

).

In quantum mechanical

Quantum mechanics

Quantum mechanics, also known as quantum physics or quantum theory, is a branch of physics providing a mathematical description of much of the dual particle-like and wave-like behavior and interactions of energy and matter. It departs from classical mechanics primarily at the atomic and subatomic...

 systems, it turns out that the weak nuclear force is not invariant under T-symmetry alone. If weak interactions are present, reversible dynamics are still possible, but only if the operator π also reverses the signs of all the charges

Charge (physics)

In physics, a charge may refer to one of many different quantities, such as the electric charge in electromagnetism or the color charge in quantum chromodynamics. Charges are associated with conserved quantum numbers.-Formal definition:...

, and the parity

Parity (physics)

In physics, a parity transformation is the flip in the sign of one spatial coordinate. In three dimensions, it is also commonly described by the simultaneous flip in the sign of all three spatial coordinates:...

 of the spatial co-ordinates (C-symmetry

C-symmetry

In physics, C-symmetry means the symmetry of physical laws under a charge-conjugation transformation. Electromagnetism, gravity and the strong interaction all obey C-symmetry, but weak interactions violate C-symmetry.-Charge reversal in electromagnetism:...

 and P-symmetry).

Stochastic processes

A stochastic

Stochastic

Stochastic refers to systems whose behaviour is intrinsically non-deterministic. A stochastic process is one whose behavior is non-deterministic, in that a system's subsequent state is determined both by the process's predictable actions and by a random element. However, according to M. Kac and E...

 process

Stochastic process

In probability theory, a stochastic process , or sometimes random process, is the counterpart to a deterministic process...

 is reversible if the statistical properties of the process are the same as the statistical properties for time-reversed data from the same process. More formally, for all sets of time increments { τ_s }, where s = 1..k for any k, the joint probabilities


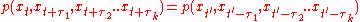
A simple consequence for Markov process

Markov process

In probability theory and statistics, a Markov process, named after the Russian mathematician Andrey Markov, is a time-varying random phenomenon for which a specific property holds...

es is that they can only be reversible if their stationary distributions have the property
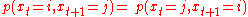
This is called the property of detailed balance

Detailed balance

The principle of detailed balance is formulated for kinetic systems which are decomposed into elementary processes : At equilibrium, each elementary process should be equilibrated by its reverse process....

.