Secular equilibrium
Encyclopedia
In nuclear physics
, secular equilibrium is a situation in which the quantity of a radioactive isotope
remains constant because its production rate (due, e.g., to decay of a parent isotope) is equal to its decay rate.
of the daughter radionuclide B is much shorter than the half-life of the parent radionuclide A. In such a situation, the decay rate of A, and hence the production rate of B, is approximately constant, because the half-life of A is very long compared to the timescales being considered. The quantity of radionuclide B builds up until the number of B atoms decaying per unit time becomes equal to the number being produced per unit time; the quantity of radionuclide B then reaches a constant, equilibrium value. Assuming the initial concentration of radionuclide B is zero, full equilibrium usually takes several half-lives of radionuclide B to establish.
The quantity of radionuclide B when secular equilibrium is reached is determined by the quantity of its parent A and the half-lives of the two radionuclide. This can be seen from the time rate of change of the number of atoms of radionuclide B:
,
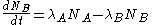where λA and λB are the decay constants of radionuclide A and B, related to their half-lives t1/2 by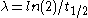
, and NA and NB are the number of atoms of A and B at a given time.
Secular equilibrium occurs when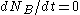
, or
.
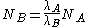Over long enough times, comparable to the half-life of radionuclide A, the secular equilibrium is only approximate; NA decays away according to
,
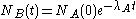and the "equilibrium" quantity of radionuclide B declines in turn. For times short compared to the half-life of A,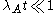
and the exponential can be approximated as 1.
Nuclear physics
Nuclear physics is the field of physics that studies the building blocks and interactions of atomic nuclei. The most commonly known applications of nuclear physics are nuclear power generation and nuclear weapons technology, but the research has provided application in many fields, including those...
, secular equilibrium is a situation in which the quantity of a radioactive isotope
Isotope
Isotopes are variants of atoms of a particular chemical element, which have differing numbers of neutrons. Atoms of a particular element by definition must contain the same number of protons but may have a distinct number of neutrons which differs from atom to atom, without changing the designation...
remains constant because its production rate (due, e.g., to decay of a parent isotope) is equal to its decay rate.
Secular equilibrium in radioactive decay
Secular equilibrium can only occur in a radioactive decay chain if the half-lifeHalf-life
Half-life, abbreviated t½, is the period of time it takes for the amount of a substance undergoing decay to decrease by half. The name was originally used to describe a characteristic of unstable atoms , but it may apply to any quantity which follows a set-rate decay.The original term, dating to...
of the daughter radionuclide B is much shorter than the half-life of the parent radionuclide A. In such a situation, the decay rate of A, and hence the production rate of B, is approximately constant, because the half-life of A is very long compared to the timescales being considered. The quantity of radionuclide B builds up until the number of B atoms decaying per unit time becomes equal to the number being produced per unit time; the quantity of radionuclide B then reaches a constant, equilibrium value. Assuming the initial concentration of radionuclide B is zero, full equilibrium usually takes several half-lives of radionuclide B to establish.
The quantity of radionuclide B when secular equilibrium is reached is determined by the quantity of its parent A and the half-lives of the two radionuclide. This can be seen from the time rate of change of the number of atoms of radionuclide B:
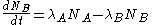,where λA and λB are the decay constants of radionuclide A and B, related to their half-lives t1/2 by
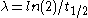, and NA and NB are the number of atoms of A and B at a given time.Secular equilibrium occurs when
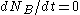, or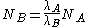 .Over long enough times, comparable to the half-life of radionuclide A, the secular equilibrium is only approximate; NA decays away according to
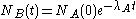,and the "equilibrium" quantity of radionuclide B declines in turn. For times short compared to the half-life of A,
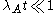and the exponential can be approximated as 1.