
Self number
Encyclopedia
A self number, Colombian number or Devlali number is an integer
which, in a given base
, cannot be generated by any other integer added to the sum of that other integer's digits. For example, 21 is not a self number, since it can be generated by the sum of 15 and the digits comprising 15, that is, 21 = 15 + 1 + 5. No such sum will generate the integer 20, hence it is a self number. These numbers were first described in 1949 by the India
n mathematician
D. R. Kaprekar
.
The first few base 10 self numbers are:
In general, for even bases, all odd
numbers below the base number are self numbers, since any number below such an odd number would have to also be a 1-digit number which when added to its digit would result in an even number. For odd bases, all odd numbers are self numbers.
A search for self numbers can turn up self-descriptive number
s, which are similar to self numbers in being base-dependent, but quite different in definition and much fewer in frequency.
generates some base 10
self numbers:
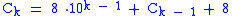
(with C1 = 9)
And for binary
numbers:

(where j stands for the number of digits) we can generalize a recurrence relation to generate self numbers in any base b:
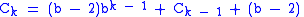
in which C1 = b − 1 for even bases and C1 = b − 2 for odd bases.
The existence of these recurrence relations shows that for any base there are infinitely many self numbers.
. The first few self primes are
In October 2006 Luke Pebody
demonstrated that the largest known Mersenne prime
that is at the same time a self number is 224036583−1. This is then the largest known self prime .
a) In general, n is self if and only if
m = R(n)+SOD(R(n))-SOD(n) is self
Where:
R(n) is the smallest rightmost digits of n, greater than 9.d(n)
d(n) is the number of digits in n
SOD(x) is the sum of digits of x, the function S10(x) from above.
b) If n = a.10^b+c, c<10^b, then n is self if and only if both {m1 & m2} are negative or self
Where:
m1 = c - SOD(a)
m2 = SOD(a-1)+9.b-(c+1)
c) For the simple case of a=1 & c=0 in the previous model (i.e. n=10^b), then n is self if and only if (9.b-1) is self
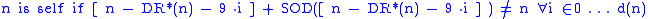
Where:
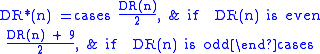

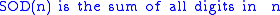
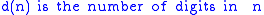
Integer
The integers are formed by the natural numbers together with the negatives of the non-zero natural numbers .They are known as Positive and Negative Integers respectively...
which, in a given base
Radix
In mathematical numeral systems, the base or radix for the simplest case is the number of unique digits, including zero, that a positional numeral system uses to represent numbers. For example, for the decimal system the radix is ten, because it uses the ten digits from 0 through 9.In any numeral...
, cannot be generated by any other integer added to the sum of that other integer's digits. For example, 21 is not a self number, since it can be generated by the sum of 15 and the digits comprising 15, that is, 21 = 15 + 1 + 5. No such sum will generate the integer 20, hence it is a self number. These numbers were first described in 1949 by the India
India
India , officially the Republic of India , is a country in South Asia. It is the seventh-largest country by geographical area, the second-most populous country with over 1.2 billion people, and the most populous democracy in the world...
n mathematician
Mathematician
A mathematician is a person whose primary area of study is the field of mathematics. Mathematicians are concerned with quantity, structure, space, and change....
D. R. Kaprekar
D. R. Kaprekar
Dattaraya Ramchandra Kaprekar was an Indian mathematician who discovered several results in number theory, including a class of numbers and a constant named after him...
.
The first few base 10 self numbers are:
- 1, 3, 5, 7, 9, 2020 (number)20 is the natural number following 19 and preceding 21. A group of twenty units may also be referred to as a score.-In mathematics:*20 is the basis for vigesimal number systems....
, 3131 (number)31 is the natural number following 30 and preceding 32.- In mathematics :Thirty-one is the third Mersenne prime as well as the fourth primorial prime, and together with twenty-nine, another primorial prime, it comprises a twin prime. As a Mersenne prime, 31 is related to the perfect number 496,...
, 4242 (number)42 is the natural number immediately following 41 and directly preceding 43. The number has received considerable attention in popular culture as a result of its central appearance in The Hitchhiker's Guide to the Galaxy as the "Answer to the Ultimate Question of Life, the Universe, and...
, 5353 (number)53 is the natural number following 52 and preceding 54.-In mathematics:Fifty-three is the 16th prime number. It is also an Eisenstein prime....
, 6464 (number)64 is the natural number following 63 and preceding 65.-In mathematics:Sixty-four is the square of 8, the cube of 4, and the sixth power of 2. It is the smallest number with exactly seven divisors. It is the lowest positive power of two that is adjacent to neither a Mersenne prime nor a Fermat...
, 7575 (number)75 is the natural number following 74 and preceding 76.-In mathematics:As the sum of the first five pentagonal numbers, seventy-five is a pentagonal pyramidal number. It is also an enneagonal number....
, 8686 (number)86 is the natural number following 85 and preceding 87.-In mathematics:86 is the 25th distinct semiprime and the 13th of the form...
, 9797 (number)97 is the natural number following 96 and preceding 98.-In mathematics:97 is the 25th prime number , following 89 and preceding 101. 97 is a Proth prime as it is 3 × 25 + 1.The numbers 97, 907, 9007, 90007 and 900007 are happy primes...
, 108108 (number)108 is the natural number following 107 and preceding 109.- In mathematics :One hundred [and] eight is an abundant number and a semiperfect number...
, 110110 (number)110 is the natural number following 109 and preceding 111.It is also known as "eleventy", a term made famous by linguist and author J. R. R...
, 121121 (number)121 is the natural number following 120 and preceding 122.-In mathematics:One hundred [and] twenty-one is a square and is the sum of three consecutive primes . There are no squares besides 121 known to be of the form 1 + p + p^2 + p^3 + p^4, where p is prime...
, 132132 (number)132 is the natural number following 131 and preceding 133.-In mathematics:132 is the sixth Catalan number. It is a pronic number, the product of 11 and 12. As it has 12 divisors total, 132 is a refactorable number....
, 143143 (number)143 is the natural number following 142 and preceding 144.-In mathematics:143 is the sum of three consecutive primes , as well as the sum of seven consecutive primes...
, 154154 (number)One hundred and fifty-four is the natural number following one hundred and fifty-three and preceding one hundred and fifty-five.-In mathematics:* 154 is a nonagonal number...
, 165, 176176 (number)176 is the natural number following 175 and preceding 177.-In mathematics:* 176 is an even number* 176 is an abundant number, as 196 is greater than 176* 176 is a cake number* 176 is a composite number...
, 187187 (number)187 is the natural number following 186 and preceding 188.-In mathematics:* 187 is an odd number* 187 is a composite number* 187 is a deficient number, as 29 is less than 187* 187 is a self number* 187 is a square-free number...
, 198, 209, 211211 (number)211 is the natural number between 210 and 212. It is also a prime number.-In mathematics:211 is an odd number.211 is a primorial prime, sum of three consecutive primes , Chen prime, centered decagonal prime, and self prime....
, 222222 (number)222 is the natural number following 221 and preceding 223. It is a repdigit composite number, and its factorization makes it a sphenic number. It is also a Harshad number....
, 233233 (number)233 is the natural number between 232 and 234. It is also a prime number.-In mathematics:233 is an irregular prime, a full reptend prime, a cousin prime, a Chen prime, a Fibonacci prime and a sexy prime. It is the 13th Fibonacci number. It is an Eisenstein prime of the form 3n - 1 with no...
, 244, 255255 (number)255 is the natural number following 254 and preceding 256.-In mathematics:Its factorization makes it a sphenic number. Since 255 = 28 - 1, it is a Mersenne number, and the fourth such number not to be a prime number...
, 266, 277277 (number)277 is the natural number following 276 and preceding 278.-In mathematics:277 is the fifty-ninth prime number, following 271 and preceding 281...
, 288, 299, 310, 312, 323, 334, 345, 356, 367, 378, 389, 400400 (number)400 is the natural number following 399 and preceding 401. - Mathematical properties :400 is the square of 20.A circle is divided into 400 grads, which is equal to 360 degrees and 2π radians...
, 411, 413, 424, 435, 446, 457, 468, 479, 490, 501, 512, 514, 525
In general, for even bases, all odd
Even and odd numbers
In mathematics, the parity of an object states whether it is even or odd.This concept begins with integers. An even number is an integer that is "evenly divisible" by 2, i.e., divisible by 2 without remainder; an odd number is an integer that is not evenly divisible by 2...
numbers below the base number are self numbers, since any number below such an odd number would have to also be a 1-digit number which when added to its digit would result in an even number. For odd bases, all odd numbers are self numbers.
A search for self numbers can turn up self-descriptive number
Self-descriptive number
A self-descriptive number is an integer m that in a given base b is b-digits long in which each digit d at position n counts how many instances of digit n are in m.For example, in base 10, the number 6210001000 is self-descriptive because of the following...
s, which are similar to self numbers in being base-dependent, but quite different in definition and much fewer in frequency.
Recurrent formula
The following recurrence relationRecurrence relation
In mathematics, a recurrence relation is an equation that recursively defines a sequence, once one or more initial terms are given: each further term of the sequence is defined as a function of the preceding terms....
generates some base 10
Decimal
The decimal numeral system has ten as its base. It is the numerical base most widely used by modern civilizations....
self numbers:
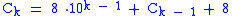
(with C1 = 9)
And for binary
Binary numeral system
The binary numeral system, or base-2 number system, represents numeric values using two symbols, 0 and 1. More specifically, the usual base-2 system is a positional notation with a radix of 2...
numbers:

(where j stands for the number of digits) we can generalize a recurrence relation to generate self numbers in any base b:
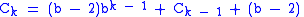
in which C1 = b − 1 for even bases and C1 = b − 2 for odd bases.
The existence of these recurrence relations shows that for any base there are infinitely many self numbers.
Self primes
A self prime is a self number that is primePrime number
A prime number is a natural number greater than 1 that has no positive divisors other than 1 and itself. A natural number greater than 1 that is not a prime number is called a composite number. For example 5 is prime, as only 1 and 5 divide it, whereas 6 is composite, since it has the divisors 2...
. The first few self primes are
- 3, 5, 7, 31, 53, 97, 211, 233, 277, 367, 389, ...
In October 2006 Luke Pebody
Luke Pebody
Luke Thomas Pebody is a mathematician who solved the necklace problem. Educated at Rugby School, and competing three times in the International Mathematical Olympiad, Luke Pebody was admitted to Cambridge University at the age of 14 to read mathematics...
demonstrated that the largest known Mersenne prime
Mersenne prime
In mathematics, a Mersenne number, named after Marin Mersenne , is a positive integer that is one less than a power of two: M_p=2^p-1.\,...
that is at the same time a self number is 224036583−1. This is then the largest known self prime .
Reduction tests
Luke Pebody showed (Oct 2006) that a link can be made between the self property of a large number n and a low-order portion of that number, adjusted for digit sums:a) In general, n is self if and only if
If and only if
In logic and related fields such as mathematics and philosophy, if and only if is a biconditional logical connective between statements....
m = R(n)+SOD(R(n))-SOD(n) is self
Where:
R(n) is the smallest rightmost digits of n, greater than 9.d(n)
d(n) is the number of digits in n
SOD(x) is the sum of digits of x, the function S10(x) from above.
b) If n = a.10^b+c, c<10^b, then n is self if and only if both {m1 & m2} are negative or self
Where:
m1 = c - SOD(a)
m2 = SOD(a-1)+9.b-(c+1)
c) For the simple case of a=1 & c=0 in the previous model (i.e. n=10^b), then n is self if and only if (9.b-1) is self
Effective test
Kaprekar demonstrated that: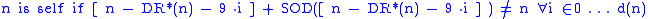
Where:
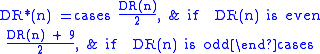

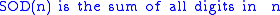
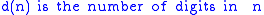
Excerpt from the table of bases where 2007 is self or Colombian
The following table was calculated in 2007.| Base | Certificate | Sum of digits |
|---|---|---|
| 40 |  | 48 |
| 41 | - | - |
| 42 | 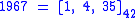 | 40 |
| 43 | - | - |
| 44 |  | 36 |
| 44 | 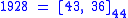 | 79 |
| 45 | - | - |
| 46 |  | 81 |
| 47 | - | - |
| 48 | - | - |
| 49 | - | - |
| 50 | 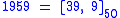 | 48 |
| 51 | - | - |
| 52 | 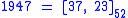 | 60 |
| 53 | - | - |
| 54 |  | 76 |
| 55 | - | - |
| 56 | 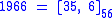 | 41 |
| 57 | - | - |
| 58 | 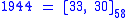 | 63 |
| 59 | - | - |
| 60 | 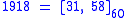 | 89 |

