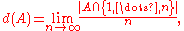Examples

• The function that assigns to each set its cardinality, i.e. the number of members of the set, is a set function.
• The function

assigning densities

Schnirelmann density

In additive number theory, the Schnirelmann density of a sequence of numbers is a way to measure how "dense" the sequence is. It is named after Russian mathematician L.G...

 to sufficiently well-behaved

Well-behaved

Mathematicians very frequently speak of whether a mathematical object — a function, a set, a space of one sort or another — is "well-behaved" or not. The term has no fixed formal definition, and is dependent on mathematical interests, fashion, and taste...

 subsets A ⊆ {1, 2, 3, ...}, is a set function.

• The Lebesgue measure
  Lebesgue measure
  In measure theory, the Lebesgue measure, named after French mathematician Henri Lebesgue, is the standard way of assigning a measure to subsets of n-dimensional Euclidean space. For n = 1, 2, or 3, it coincides with the standard measure of length, area, or volume. In general, it is also called...
  
   is a set function that assigns a non-negative real number to each set of real numbers. (Kolmogorov and Fomin 1975)
• A probability measure
  Probability measure
  In mathematics, a probability measure is a real-valued function defined on a set of events in a probability space that satisfies measure properties such as countable additivity...
  
   assigns a probability to each set in a σ-algebra
  Sigma-algebra
  In mathematics, a σ-algebra is a technical concept for a collection of sets satisfying certain properties. The main use of σ-algebras is in the definition of measures; specifically, the collection of sets over which a measure is defined is a σ-algebra...
  
  . Specifically, the probability of the empty set
  Empty set
  In mathematics, and more specifically set theory, the empty set is the unique set having no elements; its size or cardinality is zero. Some axiomatic set theories assure that the empty set exists by including an axiom of empty set; in other theories, its existence can be deduced...
  
   is zero and the probability of the sample space is 1, with other sets given probabilities between 0 and 1.
• A Possibility measure assigns a number between zero and one to each set in the powerset of some given set. See Possibility theory
  Possibility theory
  Possibility theory is a mathematical theory for dealing with certain types of uncertainty and is an alternative to probability theory. Professor Lotfi Zadeh first introduced possibility theory in 1978 as an extension of his theory of fuzzy sets and fuzzy logic. D. Dubois and H. Prade further...
  
  .
• A Random set is a set-valued random variable
  Random variable
  In probability and statistics, a random variable or stochastic variable is, roughly speaking, a variable whose value results from a measurement on some type of random process. Formally, it is a function from a probability space, typically to the real numbers, which is measurable functionmeasurable...
  
  . See Random compact set
  Random compact set
  In mathematics, a random compact set is essentially a compact set-valued random variable. Random compact sets are useful in the study of attractors for random dynamical systems.-Definition:...
  
  .