Simplicial manifold
Encyclopedia
In mathematics
, the term simplicial manifold commonly refers to either of two different types of objects, which combine attributes of a simplex
with those of a manifold
. Briefly; a simplex is a generalization of the concept of a triangle
into forms with more, or fewer, than two dimensions. Accordingly, a 3-simplex is the figure known as a tetrahedron
. A manifold is simply a space which appears to be Euclidean
(following the laws of ordinary geometry, or more generally a flat Pseudo-Riemannian
space) in a given local neighborhood, though it can be greatly more complicated overall. The combination of these concepts gives us two useful definitions.
for which the geometric realization is homeomorphic to a topological manifold
. This can mean simply that a neighborhood of each vertex (i.e. the set of simplices that contain that point as a vertex) is homeomorphic to a n-dimensional ball
.
A manifold made from simplices can be locally flat, or can approximate a smooth curve, just as a large geodesic dome
appears relatively flat over small areas, and approximates a hemisphere
over its full extent. One can generalize this concept to more dimensions and other kinds of curved surfaces which makes it useful in various kinds of simulations
.
This notion of simplicial manifold is important in Regge calculus
and Causal dynamical triangulations as a way to discretize spacetime
by triangulating
it. A simplicial manifold with a metric is called a piecewise linear space.
of manifold
s. This is a special case of a simplicial space in which, for each n , the space of n-simplices is a manifold.
For example, if G is a Lie group
, then the simplicial nerve
of G has the manifold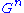
as its space of n-simplices. More generally, G can be a Lie groupoid
.
Mathematics
Mathematics is the study of quantity, space, structure, and change. Mathematicians seek out patterns and formulate new conjectures. Mathematicians resolve the truth or falsity of conjectures by mathematical proofs, which are arguments sufficient to convince other mathematicians of their validity...
, the term simplicial manifold commonly refers to either of two different types of objects, which combine attributes of a simplex
Simplex
In geometry, a simplex is a generalization of the notion of a triangle or tetrahedron to arbitrary dimension. Specifically, an n-simplex is an n-dimensional polytope which is the convex hull of its n + 1 vertices. For example, a 2-simplex is a triangle, a 3-simplex is a tetrahedron,...
with those of a manifold
Manifold
In mathematics , a manifold is a topological space that on a small enough scale resembles the Euclidean space of a specific dimension, called the dimension of the manifold....
. Briefly; a simplex is a generalization of the concept of a triangle
Triangle
A triangle is one of the basic shapes of geometry: a polygon with three corners or vertices and three sides or edges which are line segments. A triangle with vertices A, B, and C is denoted ....
into forms with more, or fewer, than two dimensions. Accordingly, a 3-simplex is the figure known as a tetrahedron
Tetrahedron
In geometry, a tetrahedron is a polyhedron composed of four triangular faces, three of which meet at each vertex. A regular tetrahedron is one in which the four triangles are regular, or "equilateral", and is one of the Platonic solids...
. A manifold is simply a space which appears to be Euclidean
Euclidean space
In mathematics, Euclidean space is the Euclidean plane and three-dimensional space of Euclidean geometry, as well as the generalizations of these notions to higher dimensions...
(following the laws of ordinary geometry, or more generally a flat Pseudo-Riemannian
Pseudo-Riemannian manifold
In differential geometry, a pseudo-Riemannian manifold is a generalization of a Riemannian manifold. It is one of many mathematical objects named after Bernhard Riemann. The key difference between a Riemannian manifold and a pseudo-Riemannian manifold is that on a pseudo-Riemannian manifold the...
space) in a given local neighborhood, though it can be greatly more complicated overall. The combination of these concepts gives us two useful definitions.
A manifold made out of simplices
A simplicial manifold is a simplicial complexSimplicial complex
In mathematics, a simplicial complex is a topological space of a certain kind, constructed by "gluing together" points, line segments, triangles, and their n-dimensional counterparts...
for which the geometric realization is homeomorphic to a topological manifold
Topological manifold
In mathematics, a topological manifold is a topological space which looks locally like Euclidean space in a sense defined below...
. This can mean simply that a neighborhood of each vertex (i.e. the set of simplices that contain that point as a vertex) is homeomorphic to a n-dimensional ball
Ball (mathematics)
In mathematics, a ball is the space inside a sphere. It may be a closed ball or an open ball ....
.
A manifold made from simplices can be locally flat, or can approximate a smooth curve, just as a large geodesic dome
Geodesic dome
A geodesic dome is a spherical or partial-spherical shell structure or lattice shell based on a network of great circles on the surface of a sphere. The geodesics intersect to form triangular elements that have local triangular rigidity and also distribute the stress across the structure. When...
appears relatively flat over small areas, and approximates a hemisphere
Sphere
A sphere is a perfectly round geometrical object in three-dimensional space, such as the shape of a round ball. Like a circle in two dimensions, a perfect sphere is completely symmetrical around its center, with all points on the surface lying the same distance r from the center point...
over its full extent. One can generalize this concept to more dimensions and other kinds of curved surfaces which makes it useful in various kinds of simulations
Computer simulation
A computer simulation, a computer model, or a computational model is a computer program, or network of computers, that attempts to simulate an abstract model of a particular system...
.
This notion of simplicial manifold is important in Regge calculus
Regge calculus
In general relativity, Regge calculus is a formalism for producing simplicial approximations of spacetimes that are solutions to the Einstein field equation. The calculus was introduced by the Italian theoretician Tullio Regge in the early 1960s....
and Causal dynamical triangulations as a way to discretize spacetime
Spacetime
In physics, spacetime is any mathematical model that combines space and time into a single continuum. Spacetime is usually interpreted with space as being three-dimensional and time playing the role of a fourth dimension that is of a different sort from the spatial dimensions...
by triangulating
Triangulation
In trigonometry and geometry, triangulation is the process of determining the location of a point by measuring angles to it from known points at either end of a fixed baseline, rather than measuring distances to the point directly...
it. A simplicial manifold with a metric is called a piecewise linear space.
A simplicial object built from manifolds
A simplicial manifold is also a simplicial object in the categoryCategory (mathematics)
In mathematics, a category is an algebraic structure that comprises "objects" that are linked by "arrows". A category has two basic properties: the ability to compose the arrows associatively and the existence of an identity arrow for each object. A simple example is the category of sets, whose...
of manifold
Manifold
In mathematics , a manifold is a topological space that on a small enough scale resembles the Euclidean space of a specific dimension, called the dimension of the manifold....
s. This is a special case of a simplicial space in which, for each n , the space of n-simplices is a manifold.
For example, if G is a Lie group
Lie group
In mathematics, a Lie group is a group which is also a differentiable manifold, with the property that the group operations are compatible with the smooth structure...
, then the simplicial nerve
Nerve (category theory)
In category theory, the nerve N of a small category C is a simplicial set constructed from the objects and morphisms of C. The geometric realization of this simplicial set is a topological space, called the classifying space of the category C...
of G has the manifold
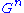as its space of n-simplices. More generally, G can be a Lie groupoidLie groupoid
In mathematics, a Lie groupoid is a groupoid where the set Ob of objects and the set Mor of morphisms are both manifolds, the source and target operationss,t : Mor \to Ob...
.