
Small number
Encyclopedia
Within a set of positive numbers, a number is small if it is close to zero. A number is smaller if it is less than another number.
Within a set of positive and negative numbers there is ambiguity, because being closer to zero does not correspond to being less, but to being less in absolute value
. Depending on context a negative number may be called "smaller" if it is closer to zero, or if it is more negative.
This article deals with positive numbers, and is also applicable to negative numbers by taking the absolute value.
Small numbers are number
s that are small compared with the numbers used in everyday life. Very small numbers often occur in fields such as chemistry
, electronics
and quantum physics.
-minutes-seconds system, it is possible to represent one second of arc, equal to
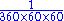
of a circle.
was created to handle very small and very large numbers.
Examples of small numbers describing everyday real-world objects are:
Other small numbers are found in particle physics
and quantum physics:
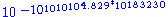
numbers. An infinitesimal is a number greater than zero yet smaller than any positive real number.
Infinitesimal numbers were originally developed to create the differential and integral calculus, but were replaced by systems using limits when they were shown to lack theoretical rigor. More recent work has restored rigor to infinitesimals, making them once more a legitimate mathematical topic (see non-standard analysis
).
Systems of infinitesimals can be generated in the same way as systems of transfinite number
s can be generated.
Some mathematical systems such as surreal number
s and hyperreal number
s generate elaborate systems of infinitesimals with amazing properties.
Within a set of positive and negative numbers there is ambiguity, because being closer to zero does not correspond to being less, but to being less in absolute value
Absolute value
In mathematics, the absolute value |a| of a real number a is the numerical value of a without regard to its sign. So, for example, the absolute value of 3 is 3, and the absolute value of -3 is also 3...
. Depending on context a negative number may be called "smaller" if it is closer to zero, or if it is more negative.
This article deals with positive numbers, and is also applicable to negative numbers by taking the absolute value.
Small numbers are number
Number
A number is a mathematical object used to count and measure. In mathematics, the definition of number has been extended over the years to include such numbers as zero, negative numbers, rational numbers, irrational numbers, and complex numbers....
s that are small compared with the numbers used in everyday life. Very small numbers often occur in fields such as chemistry
Chemistry
Chemistry is the science of matter, especially its chemical reactions, but also its composition, structure and properties. Chemistry is concerned with atoms and their interactions with other atoms, and particularly with the properties of chemical bonds....
, electronics
Electronics
Electronics is the branch of science, engineering and technology that deals with electrical circuits involving active electrical components such as vacuum tubes, transistors, diodes and integrated circuits, and associated passive interconnection technologies...
and quantum physics.
Fractions
As soon as systems of weights and measures were devised, units were subdivided into smaller units: pounds were divided into ounces, pounds into shillings and pence. Beyond the smallest units, there was a need to use vulgar fractions to represent even smaller quantities. In systems such as the degreesDegree (angle)
A degree , usually denoted by ° , is a measurement of plane angle, representing 1⁄360 of a full rotation; one degree is equivalent to π/180 radians...
-minutes-seconds system, it is possible to represent one second of arc, equal to
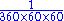
of a circle.
Tiny numbers in science
Even smaller numbers are often found in science, which are so small that they are not easily dealt with using fractions. Scientific notationScientific notation
Scientific notation is a way of writing numbers that are too large or too small to be conveniently written in standard decimal notation. Scientific notation has a number of useful properties and is commonly used in calculators and by scientists, mathematicians, doctors, and engineers.In scientific...
was created to handle very small and very large numbers.
Examples of small numbers describing everyday real-world objects are:
- size of a bit on a computer hard disk
- feature size of a structure on a microprocessorMicroprocessorA microprocessor incorporates the functions of a computer's central processing unit on a single integrated circuit, or at most a few integrated circuits. It is a multipurpose, programmable device that accepts digital data as input, processes it according to instructions stored in its memory, and...
chip - wavelength of greenGreenGreen is a color, the perception of which is evoked by light having a spectrum dominated by energy with a wavelength of roughly 520–570 nanometres. In the subtractive color system, it is not a primary color, but is created out of a mixture of yellow and blue, or yellow and cyan; it is considered...
light: 5.5 × 10-7 m - period of a 100 MHz FM radioRadioRadio is the transmission of signals through free space by modulation of electromagnetic waves with frequencies below those of visible light. Electromagnetic radiation travels by means of oscillating electromagnetic fields that pass through the air and the vacuum of space...
wave: 1 × 10-8 s - time taken by light to travel one meter: roughly 3 × 10-9 s
- radius of a hydrogenHydrogenHydrogen is the chemical element with atomic number 1. It is represented by the symbol H. With an average atomic weight of , hydrogen is the lightest and most abundant chemical element, constituting roughly 75% of the Universe's chemical elemental mass. Stars in the main sequence are mainly...
atom: 2.5 × 10-11 m - the charge on an electronElectronThe electron is a subatomic particle with a negative elementary electric charge. It has no known components or substructure; in other words, it is generally thought to be an elementary particle. An electron has a mass that is approximately 1/1836 that of the proton...
: roughly 1.6 × 10-19 C (negative)
Other small numbers are found in particle physics
Particle physics
Particle physics is a branch of physics that studies the existence and interactions of particles that are the constituents of what is usually referred to as matter or radiation. In current understanding, particles are excitations of quantum fields and interact following their dynamics...
and quantum physics:
- size of the atomic nucleusAtomic nucleusThe nucleus is the very dense region consisting of protons and neutrons at the center of an atom. It was discovered in 1911, as a result of Ernest Rutherford's interpretation of the famous 1909 Rutherford experiment performed by Hans Geiger and Ernest Marsden, under the direction of Rutherford. The...
of a lead atom: 7.1 × 10-15 m - the Planck length: 1.6 × 10-35 m
Extremely small numbers
Extremely small numbers can be described through their reciprocal, an extremely large number. The notation is similar, with a minus sign at the first level of exponents, e.g.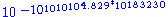
Infinitesimals
Although all these numbers above are very small, they are all still real numbers greater than zero. Some fields of mathematics define infinitesimalInfinitesimal
Infinitesimals have been used to express the idea of objects so small that there is no way to see them or to measure them. The word infinitesimal comes from a 17th century Modern Latin coinage infinitesimus, which originally referred to the "infinite-th" item in a series.In common speech, an...
numbers. An infinitesimal is a number greater than zero yet smaller than any positive real number.
Infinitesimal numbers were originally developed to create the differential and integral calculus, but were replaced by systems using limits when they were shown to lack theoretical rigor. More recent work has restored rigor to infinitesimals, making them once more a legitimate mathematical topic (see non-standard analysis
Non-standard analysis
Non-standard analysis is a branch of mathematics that formulates analysis using a rigorous notion of an infinitesimal number.Non-standard analysis was introduced in the early 1960s by the mathematician Abraham Robinson. He wrote:...
).
Systems of infinitesimals can be generated in the same way as systems of transfinite number
Transfinite number
Transfinite numbers are numbers that are "infinite" in the sense that they are larger than all finite numbers, yet not necessarily absolutely infinite. The term transfinite was coined by Georg Cantor, who wished to avoid some of the implications of the word infinite in connection with these...
s can be generated.
Some mathematical systems such as surreal number
Surreal number
In mathematics, the surreal number system is an arithmetic continuum containing the real numbers as well as infinite and infinitesimal numbers, respectively larger or smaller in absolute value than any positive real number...
s and hyperreal number
Hyperreal number
The system of hyperreal numbers represents a rigorous method of treating the infinite and infinitesimal quantities. The hyperreals, or nonstandard reals, *R, are an extension of the real numbers R that contains numbers greater than anything of the form1 + 1 + \cdots + 1. \, Such a number is...
s generate elaborate systems of infinitesimals with amazing properties.
See also
- Orders of magnitude
- floating-point numbers
- large numbersLarge numbersThis article is about large numbers in the sense of numbers that are significantly larger than those ordinarily used in everyday life, for instance in simple counting or in monetary transactions...
- human scaleHuman scaleHuman scale is the set of physical quantities, and quantities of information, characterizing the human body, its motor, sensory, or mental capabilities, and human social institutions.- Science vs...
- fractionFraction (mathematics)A fraction represents a part of a whole or, more generally, any number of equal parts. When spoken in everyday English, we specify how many parts of a certain size there are, for example, one-half, five-eighths and three-quarters.A common or "vulgar" fraction, such as 1/2, 5/8, 3/4, etc., consists...
s - Law of small numbersLaw of small numbersThe law of small numbers may refer to* The Law of Small Numbers , authored by Ladislaus Bortkiewicz** The Poisson distribution...

