
State variable filter
Encyclopedia
A state variable filter is a type of active filter
. It consists of one or more integrator
s, connected in some feedback configuration. Any LTI system can be described as a state-space model
, with n state variables for an nth-order system. A state variable filter realizes the state-space model directly. The instantaneous output voltage of one of the integrators corresponds to one of the state-space model's state variables.
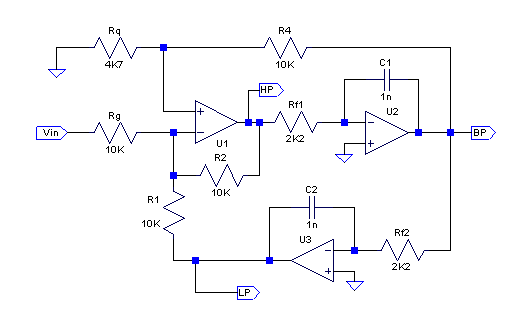
The signal input is marked Vin; the LP, HP and BP outputs give the lowpass, highpass and bandpass filtered signals respectively.
For simplicity, we set:



Then:


The pass-band gain for the LP and HP outputs is given by:

It can be seen that the frequency of operation and the Q factor
can be varied independently. This and the ability to switch between different filter responses make the state-variable filter widely used in analogue synthesizers.
Values for a resonance frequency of 1 kHz are Rf1=Rf2=10k, C1=C2=15nF and R1=R2=10k
Active filter
An active filter is a type of analog electronic filter that uses an amplifier stage. Amplifiers included in a filter design can be used to improve the performance, stability and predictability of a filter. An amplifier prevents the impedance of source or load stages from affecting the...
. It consists of one or more integrator
Integrator
An integrator is a device to perform the mathematical operation known as integration, a fundamental operation in calculus.The integration function is often part of engineering, physics, mechanical, chemical and scientific calculations....
s, connected in some feedback configuration. Any LTI system can be described as a state-space model
State space (controls)
In control engineering, a state space representation is a mathematical model of a physical system as a set of input, output and state variables related by first-order differential equations...
, with n state variables for an nth-order system. A state variable filter realizes the state-space model directly. The instantaneous output voltage of one of the integrators corresponds to one of the state-space model's state variables.
Kerwin-Huelsman-Newcomb (KHN) Biquad Filter Example
The example given below can produce simultaneous lowpass, highpass and bandpass outputs from a single input. This is a second-order (biquad) filter. Its derivation comes from rearranging a high-pass filter's transfer function, which is the ratio of two quadratic functions. The rearrangement reveals that one signal is the sum of integrated copies of another. That is, the rearrangement reveals a state variable filter structure. By using different states as outputs, different kinds of filters can be produced. In more general state variable filter examples, additional filter order is possible with more integrators (i.e., more states).
The signal input is marked Vin; the LP, HP and BP outputs give the lowpass, highpass and bandpass filtered signals respectively.
For simplicity, we set:



Then:


The pass-band gain for the LP and HP outputs is given by:

It can be seen that the frequency of operation and the Q factor
Q factor
In physics and engineering the quality factor or Q factor is a dimensionless parameter that describes how under-damped an oscillator or resonator is, or equivalently, characterizes a resonator's bandwidth relative to its center frequency....
can be varied independently. This and the ability to switch between different filter responses make the state-variable filter widely used in analogue synthesizers.
Values for a resonance frequency of 1 kHz are Rf1=Rf2=10k, C1=C2=15nF and R1=R2=10k

