
Tarski's plank problem
Encyclopedia
In mathematics, Tarski's plank problem is a question about coverings of convex regions in n-dimensional Euclidean space by "planks": regions between two hyperplanes. Tarski
asked if the sum of the widths of the planks must be at least the minimum width of the convex region. The question was answered affirmatively by
.
C in Rn and a hyperplane H, the width of C parallel to H, w(C,H), is the distance between the two supporting hyperplane
s of C that are parallel to H. The smallest such distance (i.e. the infimum
over all possible hyperplanes) is called the minimal width of C, w(C).
The (closed) set of points P between two distinct, parallel hyperplanes in Rn is called a plank, and the distance between the two hyperplanes is called the width of the plank, w(P). Tarski conjectured that if a convex body C of minimal width w(C) was covered by a collection of planks, then the sum of the widths of those planks must be at least w(C). That is, if P1,…,Pm are planks such that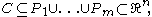
then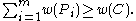
Bang proved this is indeed the case.
, and the question becomes how many planks does one need to completely cover a convex
tabletop of minimal width w? Bang's theorem shows that, for example, a circular table
of diameter
d feet can't be covered by less than d planks of wood of width one foot each.
Alfred Tarski
Alfred Tarski was a Polish logician and mathematician. Educated at the University of Warsaw and a member of the Lwow-Warsaw School of Logic and the Warsaw School of Mathematics and philosophy, he emigrated to the USA in 1939, and taught and carried out research in mathematics at the University of...
asked if the sum of the widths of the planks must be at least the minimum width of the convex region. The question was answered affirmatively by
.
Statement
Given a convex bodyConvex body
In mathematics, a convex body in n-dimensional Euclidean space Rn is a compact convex set with non-empty interior.A convex body K is called symmetric if it is centrally symmetric with respect to the origin, i.e. a point x lies in K if and only if its antipode, −x, also lies in K...
C in Rn and a hyperplane H, the width of C parallel to H, w(C,H), is the distance between the two supporting hyperplane
Supporting hyperplane
Supporting hyperplane is a concept in geometry. A hyperplane divides a space into two half-spaces. A hyperplane is said to support a set S in Euclidean space \mathbb R^n if it meets both of the following:...
s of C that are parallel to H. The smallest such distance (i.e. the infimum
Infimum
In mathematics, the infimum of a subset S of some partially ordered set T is the greatest element of T that is less than or equal to all elements of S. Consequently the term greatest lower bound is also commonly used...
over all possible hyperplanes) is called the minimal width of C, w(C).
The (closed) set of points P between two distinct, parallel hyperplanes in Rn is called a plank, and the distance between the two hyperplanes is called the width of the plank, w(P). Tarski conjectured that if a convex body C of minimal width w(C) was covered by a collection of planks, then the sum of the widths of those planks must be at least w(C). That is, if P1,…,Pm are planks such that
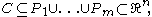
then
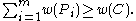
Bang proved this is indeed the case.
Nomenclature
The name for the problem, specifically for the sets of points between parallel hyperplanes, comes from the visualisation of the problem in R2. Here, hyperplanes are just straight lines and so planks become the space between two parallel lines. Thus the planks can be thought of as (infinitely long) planks of woodPlank (wood)
A plank is a piece of timber, flat, elongated and rectangular, with parallel faces, higher or longer than wide, used in the construction of ships, houses, bridges, etc......
, and the question becomes how many planks does one need to completely cover a convex
Convex
'The word convex means curving out or bulging outward, as opposed to concave. Convex or convexity may refer to:Mathematics:* Convex set, a set of points containing all line segments between each pair of its points...
tabletop of minimal width w? Bang's theorem shows that, for example, a circular table
Table (furniture)
A table is a form of furniture with a flat and satisfactory horizontal upper surface used to support objects of interest, for storage, show, and/or manipulation...
of diameter
Diameter
In geometry, a diameter of a circle is any straight line segment that passes through the center of the circle and whose endpoints are on the circle. The diameters are the longest chords of the circle...
d feet can't be covered by less than d planks of wood of width one foot each.

