Taut foliation
Encyclopedia
In mathematics
, a taut foliation is a codimension
1 foliation
of a 3-manifold
with the property that there is a single transverse circle intersecting every leaf. By transverse circle, is meant a closed loop that is always transverse to the tangent field of the foliation. Equivalently, by a result of Dennis Sullivan
, a codimension 1 foliation is taut if there exists a Riemannian metric that makes each leaf a minimal surface
.
Taut foliations were brought to prominence by the work of William Thurston
and David Gabai
.
It is closely related to the concept of Reebless foliation. A taut foliation cannot have a Reeb component, since the component would act like a "dead-end" from which a transverse curve could never escape; consequently, the boundary torus of the Reeb component has no transverse circle puncturing it. A Reebless foliation can fail to be taut but the only leaves of the foliation with no puncturing transverse circle must be compact, and in particular, homeomorphic to a torus.
The existence of a taut foliation implies various useful properties about a closed 3-manifold. For example, a closed, orientable 3-manifold, which admits a taut foliation with no sphere leaf, must be irreducible
, covered by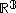
, and have negatively curved fundamental group
.
Mathematics
Mathematics is the study of quantity, space, structure, and change. Mathematicians seek out patterns and formulate new conjectures. Mathematicians resolve the truth or falsity of conjectures by mathematical proofs, which are arguments sufficient to convince other mathematicians of their validity...
, a taut foliation is a codimension
Codimension
In mathematics, codimension is a basic geometric idea that applies to subspaces in vector spaces, and also to submanifolds in manifolds, and suitable subsets of algebraic varieties.The dual concept is relative dimension.-Definition:...
1 foliation
Foliation
In mathematics, a foliation is a geometric device used to study manifolds, consisting of an integrable subbundle of the tangent bundle. A foliation looks locally like a decomposition of the manifold as a union of parallel submanifolds of smaller dimension....
of a 3-manifold
3-manifold
In mathematics, a 3-manifold is a 3-dimensional manifold. The topological, piecewise-linear, and smooth categories are all equivalent in three dimensions, so little distinction is made in whether we are dealing with say, topological 3-manifolds, or smooth 3-manifolds.Phenomena in three dimensions...
with the property that there is a single transverse circle intersecting every leaf. By transverse circle, is meant a closed loop that is always transverse to the tangent field of the foliation. Equivalently, by a result of Dennis Sullivan
Dennis Sullivan
Dennis Parnell Sullivan is an American mathematician. He is known for work in topology, both algebraic and geometric, and on dynamical systems. He holds the Albert Einstein Chair at the City University of New York Graduate Center, and is a professor at Stony Brook University.-Work in topology:He...
, a codimension 1 foliation is taut if there exists a Riemannian metric that makes each leaf a minimal surface
Minimal surface
In mathematics, a minimal surface is a surface with a mean curvature of zero.These include, but are not limited to, surfaces of minimum area subject to various constraints....
.
Taut foliations were brought to prominence by the work of William Thurston
William Thurston
William Paul Thurston is an American mathematician. He is a pioneer in the field of low-dimensional topology. In 1982, he was awarded the Fields Medal for his contributions to the study of 3-manifolds...
and David Gabai
David Gabai
David Gabai, a mathematician, is currently a professor at Princeton University. Focused on low-dimensional topology and hyperbolic geometry, he is a leading researcher in those subjects....
.
It is closely related to the concept of Reebless foliation. A taut foliation cannot have a Reeb component, since the component would act like a "dead-end" from which a transverse curve could never escape; consequently, the boundary torus of the Reeb component has no transverse circle puncturing it. A Reebless foliation can fail to be taut but the only leaves of the foliation with no puncturing transverse circle must be compact, and in particular, homeomorphic to a torus.
The existence of a taut foliation implies various useful properties about a closed 3-manifold. For example, a closed, orientable 3-manifold, which admits a taut foliation with no sphere leaf, must be irreducible
Irreducible (mathematics)
In mathematics, the concept of irreducibility is used in several ways.* In abstract algebra, irreducible can be an abbreviation for irreducible element; for example an irreducible polynomial....
, covered by
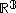, and have negatively curved fundamental groupFundamental group
In mathematics, more specifically algebraic topology, the fundamental group is a group associated to any given pointed topological space that provides a way of determining when two paths, starting and ending at a fixed base point, can be continuously deformed into each other...
.