
Toeplitz operator
Encyclopedia
In operator theory
, a Toeplitz operator is the compression of a multiplication operator
on the circle to the Hardy space
.
Mg on L2(S1). Let P be the projection from L2(S1) onto the Hardy space H2. The Toeplitz operator with symbol g is defined by
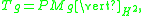
where " | " means restriction.
A bounded operator on H2 is Toeplitz if and only if its matrix representation, in the basis {zn, n ≥ 0}, has constant diagonals.
Operator theory
In mathematics, operator theory is the branch of functional analysis that focuses on bounded linear operators, but which includes closed operators and nonlinear operators.Operator theory also includes the study of algebras of operators....
, a Toeplitz operator is the compression of a multiplication operator
Multiplication operator
In operator theory, a multiplication operator is a linear operator T defined on some vector space of functions and whose value at a function φ is given by multiplication by a fixed function f...
on the circle to the Hardy space
Hardy space
In complex analysis, the Hardy spaces Hp are certain spaces of holomorphic functions on the unit disk or upper half plane. They were introduced by Frigyes Riesz , who named them after G. H. Hardy, because of the paper...
.
Details
Let S1 be the circle, with the standard Lebesgue measure, and L2(S1) be the Hilbert space of square-integrable functions. A bounded measurable function g on S1 defines a multiplication operatorMultiplication operator
In operator theory, a multiplication operator is a linear operator T defined on some vector space of functions and whose value at a function φ is given by multiplication by a fixed function f...
Mg on L2(S1). Let P be the projection from L2(S1) onto the Hardy space H2. The Toeplitz operator with symbol g is defined by
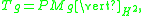
where " | " means restriction.
A bounded operator on H2 is Toeplitz if and only if its matrix representation, in the basis {zn, n ≥ 0}, has constant diagonals.

