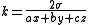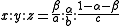Trilinear coordinates
Encyclopedia
In geometry
, the trilinear coordinates of a point relative to a given triangle
describe the relative distances from the three sides of the triangle. Trilinear coordinates are an example of homogeneous coordinates
. They are often called simply "trilinears".
where a, b, c are the respective sidelengths BC, CA, AB, and σ = area of ABC. ("Comma notation" for trilinears should be avoided, because the notation (x, y, z), which means an ordered triple, does not allow, for example, (x, y, z) = (2x, 2y, 2z), whereas the "colon notation" does allow x : y : z = 2x : 2y : 2z.)
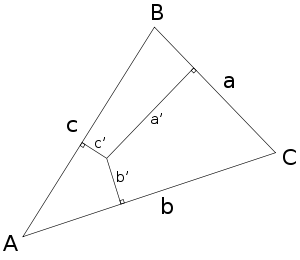
Note that, in general, the incenter is not the same as the centroid
; the centroid has barycentric coordinates
1 : 1 : 1 (these being proportional to actual signed areas of the triangles BGC, CGA, AGB, where G = centroid.)
are collinear if and only if the determinant
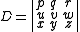
equals zero. The dual of this proposition is that the lines
concur in a point if and only if D = 0.
Also, if the actual directed distances are used when evaluating determinant D, then (area of (PUX)) = KD, where K = abc/8σ2 if triangle PUX has the same orientation as triangle ABC, and K = - abc/8σ2 otherwise.
Many cubic curves are easily represented using trilinears. For example, the pivotal self-isoconjugate cubic Z(U,P), as the locus of a point X such that the P-isoconjugate of X is on the line UX is given by the determinant equation

Among named cubics Z(U,P) are the following:
There are formulas for converting between trilinear coordinates and 2D Cartesian coordinates. Given a reference triangle ABC express the position of the vertex B in terms of an ordered pair of Cartesian coordinates and represent this algebraically as a vector a using vertex C as the origin. Similarly define the position vector of vertex A as b. Then any point P associated with the reference triangle ABC can be defined in a 2D Cartesian system as a vector P = αa + βb. If this point P has trilinear coordinates x : y : z then the conversion formulas are as follows:
conversely
If an arbitrary origin is chosen where the Cartesian coordinates of the vertices are known and represented by the vectors A, B and C and if the point P has trilinear coordinates x : y : z, then the Cartesian coordinates of P are the weighted average of the Cartesian coordinates of these vertices using the barycentric coordinates ax, by and cz as the weights.
Hence
where |C−B| = a, |A−C| = b and |B−A| = c.
Geometry
Geometry arose as the field of knowledge dealing with spatial relationships. Geometry was one of the two fields of pre-modern mathematics, the other being the study of numbers ....
, the trilinear coordinates of a point relative to a given triangle
Triangle
A triangle is one of the basic shapes of geometry: a polygon with three corners or vertices and three sides or edges which are line segments. A triangle with vertices A, B, and C is denoted ....
describe the relative distances from the three sides of the triangle. Trilinear coordinates are an example of homogeneous coordinates
Homogeneous coordinates
In mathematics, homogeneous coordinates, introduced by August Ferdinand Möbius in his 1827 work Der barycentrische Calcül, are a system of coordinates used in projective geometry much as Cartesian coordinates are used in Euclidean geometry. They have the advantage that the coordinates of points,...
. They are often called simply "trilinears".
Examples
The incenter has trilinears 1 : 1 : 1; that is, the (directed) distances from the incenter to the sidelines BC, CA, AB of a triangle ABC are proportional to the actual distances, which are the ordered triple (r, r, r), where r is the inradius of triangle ABC. Note that the notation x:y:z using colons distinguishes trilinears from actual directed distances, (kx, ky, kz), which is the usual notation for an ordered triple, and which may be obtained from x : y : z using the numberwhere a, b, c are the respective sidelengths BC, CA, AB, and σ = area of ABC. ("Comma notation" for trilinears should be avoided, because the notation (x, y, z), which means an ordered triple, does not allow, for example, (x, y, z) = (2x, 2y, 2z), whereas the "colon notation" does allow x : y : z = 2x : 2y : 2z.)

- A = 1 : 0 : 0
- B = 0 : 1 : 0
- C = 0 : 0 : 1
- incenter = 1 : 1 : 1
- centroidCentroidIn geometry, the centroid, geometric center, or barycenter of a plane figure or two-dimensional shape X is the intersection of all straight lines that divide X into two parts of equal moment about the line. Informally, it is the "average" of all points of X...
= bc : ca : ab = 1/a : 1/b : 1/c = csc A : csc B : csc C. - circumcenter = cos A : cos B : cos C.
- orthocenter = sec A : sec B : sec C.
- nine-point center = cos(B − C) : cos(C − A) : cos(A − B).
- symmedian point = a : b : c = sin A : sin B : sin C.
- A-excenter = −1 : 1 : 1
- B-excenter = 1 : −1 : 1
- C-excenter = 1 : 1 : −1.
Note that, in general, the incenter is not the same as the centroid
Centroid
In geometry, the centroid, geometric center, or barycenter of a plane figure or two-dimensional shape X is the intersection of all straight lines that divide X into two parts of equal moment about the line. Informally, it is the "average" of all points of X...
; the centroid has barycentric coordinates
Barycentric coordinates (mathematics)
In geometry, the barycentric coordinate system is a coordinate system in which the location of a point is specified as the center of mass, or barycenter, of masses placed at the vertices of a simplex . Barycentric coordinates are a form of homogeneous coordinates...
1 : 1 : 1 (these being proportional to actual signed areas of the triangles BGC, CGA, AGB, where G = centroid.)
Formulas
Trilinears enable many algebraic methods in triangle geometry. For example, three points- P = p : q : r
- U = u : v : w
- X = x : y : z
are collinear if and only if the determinant
Determinant
In linear algebra, the determinant is a value associated with a square matrix. It can be computed from the entries of the matrix by a specific arithmetic expression, while other ways to determine its value exist as well...
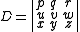
equals zero. The dual of this proposition is that the lines
- pα + qβ + rγ = 0
- uα + vβ + wγ = 0,
- xα + yβ + zγ = 0
concur in a point if and only if D = 0.
Also, if the actual directed distances are used when evaluating determinant D, then (area of (PUX)) = KD, where K = abc/8σ2 if triangle PUX has the same orientation as triangle ABC, and K = - abc/8σ2 otherwise.
Many cubic curves are easily represented using trilinears. For example, the pivotal self-isoconjugate cubic Z(U,P), as the locus of a point X such that the P-isoconjugate of X is on the line UX is given by the determinant equation

Among named cubics Z(U,P) are the following:
- Thomson cubic: Z(X(2),X(1)), where X(2) = centroidCentroidIn geometry, the centroid, geometric center, or barycenter of a plane figure or two-dimensional shape X is the intersection of all straight lines that divide X into two parts of equal moment about the line. Informally, it is the "average" of all points of X...
, X(1) = incenter - Feuerbach cubic: Z(X(5),X(1)), where X(5) = Feuerbach point
- Darboux cubic: Z(X(20),X(1)), where X(20) = De Longchamps pointDe Longchamps pointIn geometry, the de Longchamps point of a triangle is the reflection of its orthocenter about its circumcenter. It is listed as X in the Encyclopedia of Triangle Centers. Its trilinear coordinates are\displaystyle\cos A - \cos B \cos C : \cos B - \cos C \cos A : \cos C - \cos A \cos BThe point is...
- Neuberg cubic: Z(X(30),X(1)), where X(30) = Euler infinity point.
Conversions
A point with trilinears α : β : γ has barycentric coordinates aα : bβ : cγ where a, b, c are the sidelengths of the triangle. Conversely, a point with barycentrics α : β : γ has trilinears α/a : β/b : γ/c.There are formulas for converting between trilinear coordinates and 2D Cartesian coordinates. Given a reference triangle ABC express the position of the vertex B in terms of an ordered pair of Cartesian coordinates and represent this algebraically as a vector a using vertex C as the origin. Similarly define the position vector of vertex A as b. Then any point P associated with the reference triangle ABC can be defined in a 2D Cartesian system as a vector P = αa + βb. If this point P has trilinear coordinates x : y : z then the conversion formulas are as follows:
conversely
If an arbitrary origin is chosen where the Cartesian coordinates of the vertices are known and represented by the vectors A, B and C and if the point P has trilinear coordinates x : y : z, then the Cartesian coordinates of P are the weighted average of the Cartesian coordinates of these vertices using the barycentric coordinates ax, by and cz as the weights.
Hence
where |C−B| = a, |A−C| = b and |B−A| = c.
External links
- Encyclopedia of Triangle Centers - ETC by Clark Kimberling; has trilinear coordinates (and barycentric) for more than 3200 triangle centers