
Triple torus
Encyclopedia
Triple torus or three-torus can refer to one of the two following concepts, both related to a torus
.
of three circles,
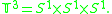
In contrast, the usual torus is the Cartesian product of two circles only.
The triple torus is a three-dimensional compact
manifold
with no boundary. It can be obtained by gluing the three pairs of opposite faces of a cube
. (After gluing the first pair of opposite faces the cube looks like a thick washer
, after gluing the second pair — the flat faces of the washer — it looks like a hollow torus, the last gluing — the inner surface of the hollow torus to the outer surface — is physically impossible in three-dimensional space so it has to happen in four dimensions.)
closed surface
with three holes, or, in other words, a surface of genus
three. It can be obtained by attaching three handles to a sphere
or by gluing (taking the connected sum
) of three tori
.
Torus
In geometry, a torus is a surface of revolution generated by revolving a circle in three dimensional space about an axis coplanar with the circle...
.
Three-dimensional torus
The three-dimensional torus, or triple torus, is defined as the Cartesian productCartesian product
In mathematics, a Cartesian product is a construction to build a new set out of a number of given sets. Each member of the Cartesian product corresponds to the selection of one element each in every one of those sets...
of three circles,
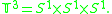
In contrast, the usual torus is the Cartesian product of two circles only.
The triple torus is a three-dimensional compact
Compact space
In mathematics, specifically general topology and metric topology, a compact space is an abstract mathematical space whose topology has the compactness property, which has many important implications not valid in general spaces...
manifold
Manifold
In mathematics , a manifold is a topological space that on a small enough scale resembles the Euclidean space of a specific dimension, called the dimension of the manifold....
with no boundary. It can be obtained by gluing the three pairs of opposite faces of a cube
Cube
In geometry, a cube is a three-dimensional solid object bounded by six square faces, facets or sides, with three meeting at each vertex. The cube can also be called a regular hexahedron and is one of the five Platonic solids. It is a special kind of square prism, of rectangular parallelepiped and...
. (After gluing the first pair of opposite faces the cube looks like a thick washer
Washer (hardware)
A washer is a thin plate with a hole that is normally used to distribute the load of a threaded fastener, such as a screw or nut. Other uses are as a spacer, spring , wear pad, preload indicating device, locking device, and to reduce vibration...
, after gluing the second pair — the flat faces of the washer — it looks like a hollow torus, the last gluing — the inner surface of the hollow torus to the outer surface — is physically impossible in three-dimensional space so it has to happen in four dimensions.)
Torus-like surface with three holes
In the theory of surfaces, a triple torus refers to a smoothSmooth function
In mathematical analysis, a differentiability class is a classification of functions according to the properties of their derivatives. Higher order differentiability classes correspond to the existence of more derivatives. Functions that have derivatives of all orders are called smooth.Most of...
closed surface
Surface
In mathematics, specifically in topology, a surface is a two-dimensional topological manifold. The most familiar examples are those that arise as the boundaries of solid objects in ordinary three-dimensional Euclidean space R3 — for example, the surface of a ball...
with three holes, or, in other words, a surface of genus
Genus (mathematics)
In mathematics, genus has a few different, but closely related, meanings:-Orientable surface:The genus of a connected, orientable surface is an integer representing the maximum number of cuttings along non-intersecting closed simple curves without rendering the resultant manifold disconnected. It...
three. It can be obtained by attaching three handles to a sphere
Sphere
A sphere is a perfectly round geometrical object in three-dimensional space, such as the shape of a round ball. Like a circle in two dimensions, a perfect sphere is completely symmetrical around its center, with all points on the surface lying the same distance r from the center point...
or by gluing (taking the connected sum
Connected sum
In mathematics, specifically in topology, the operation of connected sum is a geometric modification on manifolds. Its effect is to join two given manifolds together near a chosen point on each...
) of three tori
Torus
In geometry, a torus is a surface of revolution generated by revolving a circle in three dimensional space about an axis coplanar with the circle...
.

