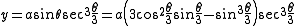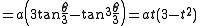Tschirnhausen cubic
Encyclopedia
In geometry
, Tschirnhausen cubic, is a plane curve
defined by the polar equation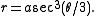
, de L'Hôpital
and Catalan
. It was given the name Tschirnhausen cubic in a 1900 paper by R C Archibald, though it is sometimes known as de L'Hôpital's cubic or the trisectrix of Catalan.
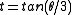 . Then applying triple-angle formulas
. Then applying triple-angle formulas
gives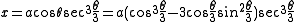
giving a parametric
form for the curve. The parameter t can be eliminated easily giving the Cartesian equation
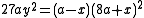 .
.
If the curve is translated horizontally by 8a then the equations become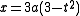
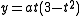
or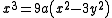 .
.
This gives an alternate polar form of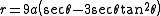 .
.
Geometry
Geometry arose as the field of knowledge dealing with spatial relationships. Geometry was one of the two fields of pre-modern mathematics, the other being the study of numbers ....
, Tschirnhausen cubic, is a plane curve
Plane curve
In mathematics, a plane curve is a curve in a Euclidean plane . The most frequently studied cases are smooth plane curves , and algebraic plane curves....
defined by the polar equation
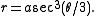
History
The curve was studied by von TschirnhausEhrenfried Walther von Tschirnhaus
Ehrenfried Walther von Tschirnhaus was a German mathematician, physicist, physician, and philosopher...
, de L'Hôpital
Guillaume de l'Hôpital
Guillaume François Antoine, Marquis de l'Hôpital was a French mathematician. His name is firmly associated with l'Hôpital's rule for calculating limits involving indeterminate forms 0/0 and ∞/∞...
and Catalan
Eugène Charles Catalan
Eugène Charles Catalan was a French and Belgian mathematician.- Biography :Catalan was born in Bruges , the only child of a French jeweller by the name of Joseph Catalan, in 1814. In 1825, he traveled to Paris and learned mathematics at École Polytechnique, where he met Joseph Liouville...
. It was given the name Tschirnhausen cubic in a 1900 paper by R C Archibald, though it is sometimes known as de L'Hôpital's cubic or the trisectrix of Catalan.
Other equations
Put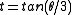 . Then applying triple-angle formulas
. Then applying triple-angle formulasDe Moivre's formula
In mathematics, de Moivre's formula , named after Abraham de Moivre, states that for any complex number x and integer n it holds that...
gives
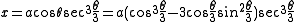
giving a parametric
Parametric equation
In mathematics, parametric equation is a method of defining a relation using parameters. A simple kinematic example is when one uses a time parameter to determine the position, velocity, and other information about a body in motion....
form for the curve. The parameter t can be eliminated easily giving the Cartesian equation
Cartesian coordinate system
A Cartesian coordinate system specifies each point uniquely in a plane by a pair of numerical coordinates, which are the signed distances from the point to two fixed perpendicular directed lines, measured in the same unit of length...
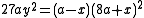 .
.If the curve is translated horizontally by 8a then the equations become
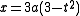
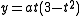
or
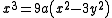 .
.This gives an alternate polar form of
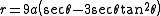 .
.