Unicoherent
Encyclopedia
A topological space
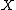is said to be unicoherent if it is connected
and the following property holds:
For any closed, connected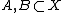
with 
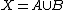, the intersection 
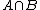is connected.
For example, any closed interval on the real line is unicoherent, but a circle is not.
Topological space
Topological spaces are mathematical structures that allow the formal definition of concepts such as convergence, connectedness, and continuity. They appear in virtually every branch of modern mathematics and are a central unifying notion...
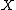is said to be unicoherent if it is connectedConnected space
In topology and related branches of mathematics, a connected space is a topological space that cannot be represented as the union of two or more disjoint nonempty open subsets. Connectedness is one of the principal topological properties that is used to distinguish topological spaces...
and the following property holds:
For any closed, connected
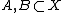with 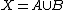, the intersection 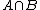is connected.For example, any closed interval on the real line is unicoherent, but a circle is not.