
Wall-Sun-Sun prime
Encyclopedia
In number theory
, a Wall–Sun–Sun prime or Fibonacci–Wieferich prime is a certain kind of prime number
which is conjectured to exist although none are known.
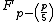 , where the Legendre symbol
, where the Legendre symbol
 is defined as
is defined as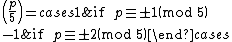
In 2007, Richard J. McIntosh and Eric L. Roettger showed that if any exist, they must be > 2.
Dorais and Klyve extended this range to 9.7 without finding such a prime.
, Zhi Hong Sun and Zhi Wei Sun; Z. H. Sun and Z. W. Sun showed in 1992 that if the first case of Fermat's last theorem
was false for a certain prime p, then p would have to be a Wall–Sun–Sun prime. As a result, prior to Andrew Wiles
' proof of Fermat's last theorem, the search for Wall–Sun–Sun primes was also the search for a counterexample
to this centuries-old conjecture
.
Number theory
Number theory is a branch of pure mathematics devoted primarily to the study of the integers. Number theorists study prime numbers as well...
, a Wall–Sun–Sun prime or Fibonacci–Wieferich prime is a certain kind of prime number
Prime number
A prime number is a natural number greater than 1 that has no positive divisors other than 1 and itself. A natural number greater than 1 that is not a prime number is called a composite number. For example 5 is prime, as only 1 and 5 divide it, whereas 6 is composite, since it has the divisors 2...
which is conjectured to exist although none are known.
Definition
A prime p > 5 is called a Wall–Sun–Sun prime if p2 divides the Fibonacci numberFibonacci number
In mathematics, the Fibonacci numbers are the numbers in the following integer sequence:0,\;1,\;1,\;2,\;3,\;5,\;8,\;13,\;21,\;34,\;55,\;89,\;144,\; \ldots\; ....
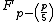 , where the Legendre symbol
, where the Legendre symbolLegendre symbol
In number theory, the Legendre symbol is a multiplicative function with values 1, −1, 0 that is a quadratic character modulo a prime number p: its value on a quadratic residue mod p is 1 and on a quadratic non-residue is −1....
 is defined as
is defined as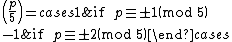
Existence
It has been conjectured that there are infinitely many Wall–Sun–Sun primes. No Wall–Sun–Sun primes are known .In 2007, Richard J. McIntosh and Eric L. Roettger showed that if any exist, they must be > 2.
Dorais and Klyve extended this range to 9.7 without finding such a prime.
History
Wall–Sun–Sun primes are named after D. D. WallD. D. Wall
Donald Dines Wall was a mathematician working primarily on number theory. He obtained his Ph.D. on normal numbers from University of California, Berkeley in 1949, where his adviser was Derrick Henry Lehmer...
, Zhi Hong Sun and Zhi Wei Sun; Z. H. Sun and Z. W. Sun showed in 1992 that if the first case of Fermat's last theorem
Fermat's Last Theorem
In number theory, Fermat's Last Theorem states that no three positive integers a, b, and c can satisfy the equation an + bn = cn for any integer value of n greater than two....
was false for a certain prime p, then p would have to be a Wall–Sun–Sun prime. As a result, prior to Andrew Wiles
Andrew Wiles
Sir Andrew John Wiles KBE FRS is a British mathematician and a Royal Society Research Professor at Oxford University, specializing in number theory...
' proof of Fermat's last theorem, the search for Wall–Sun–Sun primes was also the search for a counterexample
Counterexample
In logic, and especially in its applications to mathematics and philosophy, a counterexample is an exception to a proposed general rule. For example, consider the proposition "all students are lazy"....
to this centuries-old conjecture
Conjecture
A conjecture is a proposition that is unproven but is thought to be true and has not been disproven. Karl Popper pioneered the use of the term "conjecture" in scientific philosophy. Conjecture is contrasted by hypothesis , which is a testable statement based on accepted grounds...
.
External links
- Chris Caldwell, The Prime Glossary: Wall–Sun–Sun prime at the Prime PagesPrime pagesThe Prime Pages is a website about prime numbers maintained by Chris Caldwell at the University of Tennessee at Martin.The site maintains the list of the "5,000 largest known primes", selected smaller primes of special forms, and many "top twenty" lists for primes of various forms...
. - Richard McIntosh, Status of the search for Wall–Sun–Sun primes (October 2003)

