
5-simplex honeycomb
Encyclopedia
| 5-simplex honeycomb | |
|---|---|
| (No image) | |
| Type | Uniform honeycomb Convex uniform honeycomb In geometry, a convex uniform honeycomb is a uniform tessellation which fills three-dimensional Euclidean space with non-overlapping convex uniform polyhedral cells.Twenty-eight such honeycombs exist:* the familiar cubic honeycomb and 7 truncations thereof;... |
| Family | Simplectic honeycomb |
| Schläfli symbol | {3[6]} |
| Coxeter–Dynkin diagrams | |
| 5-face types | {3,3,3,3} Hexateron In five dimensional geometry, a 5-simplex is a self-dual regular 5-polytope. It has 6 vertices, 15 edges, 20 triangle faces, 15 tetrahedral cells, and 6 pentachoron facets. It has a dihedral angle of cos−1, or approximately 78.46°.- Alternate names :... t1{3,3,3,3} Rectified hexateron In five-dimensional geometry, a rectified 5-simplex is a convex uniform 5-polytope, being a rectification of the regular 5-simplex.There are three unique degrees of rectifications, including the zeroth, the 5-simplex itself. Vertices of the rectified 5-simplex are located at the edge-centers of the... t2{3,3,3,3} |
| 4-face types | {3,3,3} t1{3,3,3} Rectified 5-cell In four dimensional geometry, the rectified 5-cell is a uniform polychoron composed of 5 regular tetrahedral and 5 regular octahedral cells. Each edge has one tetrahedron and two octahedra. Each vertex has two tetrahedra and three octahedra. In total it has 30 triangle faces, 30 edges, and 10... |
| Cell types | {3,3} Tetrahedron In geometry, a tetrahedron is a polyhedron composed of four triangular faces, three of which meet at each vertex. A regular tetrahedron is one in which the four triangles are regular, or "equilateral", and is one of the Platonic solids... t1{3,3} Octahedron In geometry, an octahedron is a polyhedron with eight faces. A regular octahedron is a Platonic solid composed of eight equilateral triangles, four of which meet at each vertex.... |
| Face types | {3} Triangle A triangle is one of the basic shapes of geometry: a polygon with three corners or vertices and three sides or edges which are line segments. A triangle with vertices A, B, and C is denoted .... |
| Vertex figure | t04{34} |
| Coxeter group Coxeter group In mathematics, a Coxeter group, named after H.S.M. Coxeter, is an abstract group that admits a formal description in terms of mirror symmetries. Indeed, the finite Coxeter groups are precisely the finite Euclidean reflection groups; the symmetry groups of regular polyhedra are an example... s |
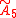 , [3[6]] , [3[6]] |
| Properties | vertex-transitive Vertex-transitive In geometry, a polytope is isogonal or vertex-transitive if, loosely speaking, all its vertices are the same... |
In five-dimensional Euclidean geometry
Euclidean geometry
Euclidean geometry is a mathematical system attributed to the Alexandrian Greek mathematician Euclid, which he described in his textbook on geometry: the Elements. Euclid's method consists in assuming a small set of intuitively appealing axioms, and deducing many other propositions from these...
, the 5-simplex honeycomb or hexateric honeycomb is a space-filling tessellation
Tessellation
A tessellation or tiling of the plane is a pattern of plane figures that fills the plane with no overlaps and no gaps. One may also speak of tessellations of parts of the plane or of other surfaces. Generalizations to higher dimensions are also possible. Tessellations frequently appeared in the art...
(or honeycomb
Honeycomb (geometry)
In geometry, a honeycomb is a space filling or close packing of polyhedral or higher-dimensional cells, so that there are no gaps. It is an example of the more general mathematical tiling or tessellation in any number of dimensions....
or pentacomb). Each vertex is shared by 12 5-simplexes, 30 rectified 5-simplexes, and 20 birectified 5-simplexes. These facet types occur in proportions of 2:2:1 respectively in the whole honeycomb.
This vertex arrangement
Vertex arrangement
In geometry, a vertex arrangement is a set of points in space described by their relative positions. They can be described by their use in polytopes....
is called the A5 lattice or 5-simplex lattice. The 30 vertices of the stericated 5-simplex vertex figure represent the 30 roots of the
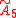 Coxeter group.
Coxeter group.Related polytopes and honeycombs
This honeycomb is one of 12 unique uniform honycombs constructed by the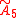 Coxeter group
Coxeter groupCoxeter group
In mathematics, a Coxeter group, named after H.S.M. Coxeter, is an abstract group that admits a formal description in terms of mirror symmetries. Indeed, the finite Coxeter groups are precisely the finite Euclidean reflection groups; the symmetry groups of regular polyhedra are an example...
. The Coxeter–Dynkin diagrams of the other 11 are:
,
,
,
,
,
,
,
,
,
,
.
Projection by folding
The 5-simplex honeycomb can be projected into the 3-dimensional cubic honeycombCubic honeycomb
The cubic honeycomb is the only regular space-filling tessellation in Euclidean 3-space, made up of cubic cells. It has 4 cubes around every edge, and 8 cubes around each vertex. Its vertex figure is a regular octahedron....
by a geometric folding operation that maps two pairs of mirrors into each other, sharing the same vertex arrangement
Vertex arrangement
In geometry, a vertex arrangement is a set of points in space described by their relative positions. They can be described by their use in polytopes....
:
 |
|
|---|---|
 |
See also
- Regular and uniform honeycombs in 5-space:
- Penteractic honeycomb
- Demipenteractic honeycomb
- Truncated 5-simplex honeycombTruncated 5-simplex honeycombIn five-dimensional Euclidean geometry, the truncated 5-simplex honeycomb or truncated hexateric honeycomb is a space-filling tessellation...
- Omnitruncated 5-simplex honeycombOmnitruncated 5-simplex honeycombIn five-dimensional Euclidean geometry, the omnitruncated 5-simplex honeycomb or omnitruncated hexateric honeycomb is a space-filling tessellation . It is composed entirely of omnitruncated 5-simplex facets....

