
Acnode
Encyclopedia
An acnode is an isolated point not on a curve
, but whose coordinates satisfy the equation of the curve. The term "isolated point
" or "hermit point" is an equivalent term.
Acnodes commonly occur when studying algebraic curve
s over field
s which are not algebraically closed, defined as the zero set of a polynomial of two variables. For example the equation
has an acnode at the origin of , because it is equivalent to
, because it is equivalent to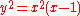
and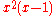 is non-negative when
is non-negative when  ≥ 1 and when
≥ 1 and when  . Thus, over the real numbers the equation has no solutions for
. Thus, over the real numbers the equation has no solutions for  except for (0, 0).
except for (0, 0).
In contrast, over the complex numbers the origin is not isolated since square roots of negative real numbers exist.
An acnode is a singularity
of the function, where both partial derivatives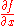 and
and 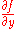 vanish. Further the Hessian matrix
vanish. Further the Hessian matrix
of second derivatives will be positive definite
or negative definite. Hence the function has a local minimum or a local maximum.
Curve
In mathematics, a curve is, generally speaking, an object similar to a line but which is not required to be straight...
, but whose coordinates satisfy the equation of the curve. The term "isolated point
Isolated point
In topology, a branch of mathematics, a point x of a set S is called an isolated point of S, if there exists a neighborhood of x not containing other points of S.In particular, in a Euclidean space ,...
" or "hermit point" is an equivalent term.
Acnodes commonly occur when studying algebraic curve
Algebraic curve
In algebraic geometry, an algebraic curve is an algebraic variety of dimension one. The theory of these curves in general was quite fully developed in the nineteenth century, after many particular examples had been considered, starting with circles and other conic sections.- Plane algebraic curves...
s over field
Field (mathematics)
In abstract algebra, a field is a commutative ring whose nonzero elements form a group under multiplication. As such it is an algebraic structure with notions of addition, subtraction, multiplication, and division, satisfying certain axioms...
s which are not algebraically closed, defined as the zero set of a polynomial of two variables. For example the equation

has an acnode at the origin of
 , because it is equivalent to
, because it is equivalent to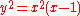
and
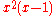 is non-negative when
is non-negative when  ≥ 1 and when
≥ 1 and when  . Thus, over the real numbers the equation has no solutions for
. Thus, over the real numbers the equation has no solutions for  except for (0, 0).
except for (0, 0).In contrast, over the complex numbers the origin is not isolated since square roots of negative real numbers exist.
An acnode is a singularity
Singularity theory
-The notion of singularity:In mathematics, singularity theory is the study of the failure of manifold structure. A loop of string can serve as an example of a one-dimensional manifold, if one neglects its width. What is meant by a singularity can be seen by dropping it on the floor...
of the function, where both partial derivatives
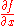 and
and 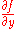 vanish. Further the Hessian matrix
vanish. Further the Hessian matrixHessian matrix
In mathematics, the Hessian matrix is the square matrix of second-order partial derivatives of a function; that is, it describes the local curvature of a function of many variables. The Hessian matrix was developed in the 19th century by the German mathematician Ludwig Otto Hesse and later named...
of second derivatives will be positive definite
Positive-definite matrix
In linear algebra, a positive-definite matrix is a matrix that in many ways is analogous to a positive real number. The notion is closely related to a positive-definite symmetric bilinear form ....
or negative definite. Hence the function has a local minimum or a local maximum.

