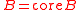Algebraic interior
Encyclopedia
In functional analysis
, a branch of mathematics, the algebraic interior or radial kernel of a subset of a vector space
is a refinement of the concept of the interior
. It is the subset of points contained in a given set that it is absorbing
with respect to, i.e. the radial points of the set.
Formally, if is a linear space then the algebraic interior of
is a linear space then the algebraic interior of  is
is
Note that in general , but if
, but if  is a convex set
is a convex set
then . In fact, if
. In fact, if  is a convex set then if
is a convex set then if 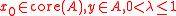 then
then 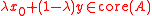 .
.
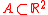 such that
such that 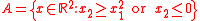 then
then  , but
, but  and
and  .
.
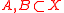 then:
then:
Functional analysis
Functional analysis is a branch of mathematical analysis, the core of which is formed by the study of vector spaces endowed with some kind of limit-related structure and the linear operators acting upon these spaces and respecting these structures in a suitable sense...
, a branch of mathematics, the algebraic interior or radial kernel of a subset of a vector space
Vector space
A vector space is a mathematical structure formed by a collection of vectors: objects that may be added together and multiplied by numbers, called scalars in this context. Scalars are often taken to be real numbers, but one may also consider vector spaces with scalar multiplication by complex...
is a refinement of the concept of the interior
Interior (topology)
In mathematics, specifically in topology, the interior of a set S of points of a topological space consists of all points of S that do not belong to the boundary of S. A point that is in the interior of S is an interior point of S....
. It is the subset of points contained in a given set that it is absorbing
Absorbing set
In functional analysis and related areas of mathematics an absorbing set in a vector space is a set S which can be inflated to include any element of the vector space...
with respect to, i.e. the radial points of the set.
Formally, if
 is a linear space then the algebraic interior of
is a linear space then the algebraic interior of  is
is
Note that in general
 , but if
, but if  is a convex set
is a convex setConvex set
In Euclidean space, an object is convex if for every pair of points within the object, every point on the straight line segment that joins them is also within the object...
then
 . In fact, if
. In fact, if  is a convex set then if
is a convex set then if 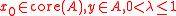 then
then 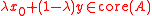 .
.Example
If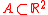 such that
such that 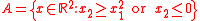 then
then  , but
, but  and
and  .
.Properties
Let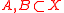 then:
then:
-
 is absorbingAbsorbing setIn functional analysis and related areas of mathematics an absorbing set in a vector space is a set S which can be inflated to include any element of the vector space...
is absorbingAbsorbing setIn functional analysis and related areas of mathematics an absorbing set in a vector space is a set S which can be inflated to include any element of the vector space...
if and only if .
. -
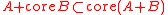
-
 if
if